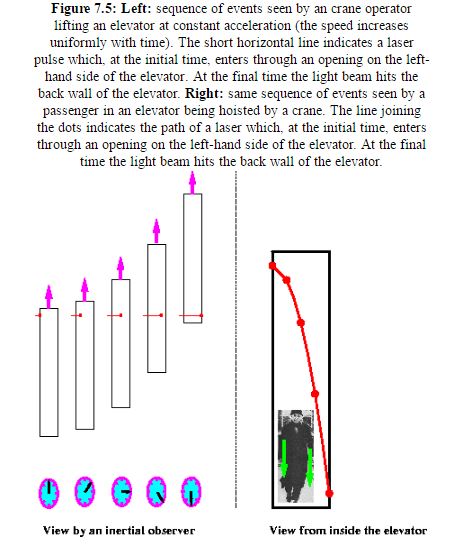
I have been told that light rays seen from inside an accelerated elevator will seen as following a curved path, parabola. Like the image below. But what happens when a pulse of light entered into a non accelerating but uniformly going upward. It seemed to me that like the image below the pulse will entered at the top of the elevator and then after an amount of time light will move forward a bit. Meanwhile the elevator will go a bit upward. so when viewing from inside it will appear that the light rays have again followed a parabolic path! in a non accelerating elevator! I know that can't be true. Where am I going wrong?
$\begingroup$
$\endgroup$
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
3
The path won't be parabolic if the elevator is moving at constant velocity; rather, it will be a straight line. To see this, let's define the vertical position of the light pulse to be $z_L = 0$. If the elevator is moving at constant velocity, the position of the elevator floor as a function of time is $$ z_E(t) = z_0 + v t $$ where $t$ is the amount of time elapsed.1 Thus, the vertical distance $h$ between the light pulse and the elevator floor will be $$ h = z_L - z_E = - z_0 - v t, $$ i.e., the pulse's vertical velocity is constant. (Note that $d^2 h/dt^2 = - d^2 z_E/dt^2 = 0$.) Its sideways velocity is also constant, so the light pulse moves at a constant velocity. This does not allow one to distinguish between an elevator "at rest" and an elevator moving at constant velocity, since light pulse move with constant velocity in both cases.
By comparison, if the elevator is accelerating, then $d^2 z_E/dt^2 \neq 0$, which implies that $d^2h/dt^2 \neq 0$ as well. Thus, as viewed in the elevator frame, the light ray will be accelerating towards the floor of the elevator.
1 As measured by clocks outside the elevator. I'm glossing over quite a few details of the Lorentz transformations here, but the argument is basically the same when they are included.
answered Nov 17, 2016 at 14:55
-
$\begingroup$ You didn't notice that I mentioned light rays were entering from outside a source whose vertical velocity is zero with respect to an outside frame of reference. Where's the vertical velocity of elevator isn't zero with respect to the same frame. $\endgroup$ Commented Nov 17, 2016 at 15:10
-
$\begingroup$ I'm not sure what you mean. I did assume the light rays were moving at zero vertical velocity according to an external frame: $dz_L/dt = 0$. I also assumed that the elevator was moving at a non-zero (but constant) velocity with respect to the external frame ($dz_E /dt = v = \text{const.}$) The point is that the vertical velocity of the light ray with respect to the elevator floor will change if and only if there is a change in the velocity of the elevator as measured in the external frame. $\endgroup$ Commented Nov 17, 2016 at 15:18
-
$\begingroup$ Thanks. First time I didn't quiet catch your answer. Now I got it. again thanks for your quick reply. $\endgroup$ Commented Nov 17, 2016 at 16:32
$\begingroup$
$\endgroup$
Your picture is used to help visualize a thought experiment where the speed could be constant or accelerating depending on the statement. In reality you would only see a light spot on the right wall. If the elevator was moving at normal speeds, the spot would be directly across from the opening whether moving at constant or accelerated speeds. The faster the elevator moves, the lower the spot will be. When the speed is constant the light spot is stationary. If the speed is accelerating faster then the spot slowly moves down the wall. Keep in mind the light is not curving but the wall of the elevator is rising before the light gets there.
answered Nov 11, 2019 at 6:33