The Lagrangian of a single particle of mass $m$ is
$$ L=\dfrac{1}{2}m\dot{\textbf{r}}^2 -\dfrac{1}{2}kr^2+m\dot{\textbf{r}} \cdot (\vec{\omega} \times \textbf{r}) \tag{1}$$
where $\vec{\omega}=\omega \textbf{e}_3$.
Hint: the generalized coordinates are $(r,\theta)$ because the particle moves in $(x,y)$ plane for all time $t>0$. The motion equations are
$\hspace{6cm}\cases{r=a \cos(\Omega t)+\dfrac{\dot{r}(0)}{\Omega}\sin(\Omega t), \\ \theta=\theta_0 -\omega t,}$
where $r(0)=a$ and $k,\omega>0$. The relation between the two frequencies is
$\hspace{6cm}\Omega \equiv \sqrt{\dfrac{k}{m}+\omega^2}.$
The constants of the motion are the Hamiltonian $H$ and the polar generalized momentum $p_\theta$.
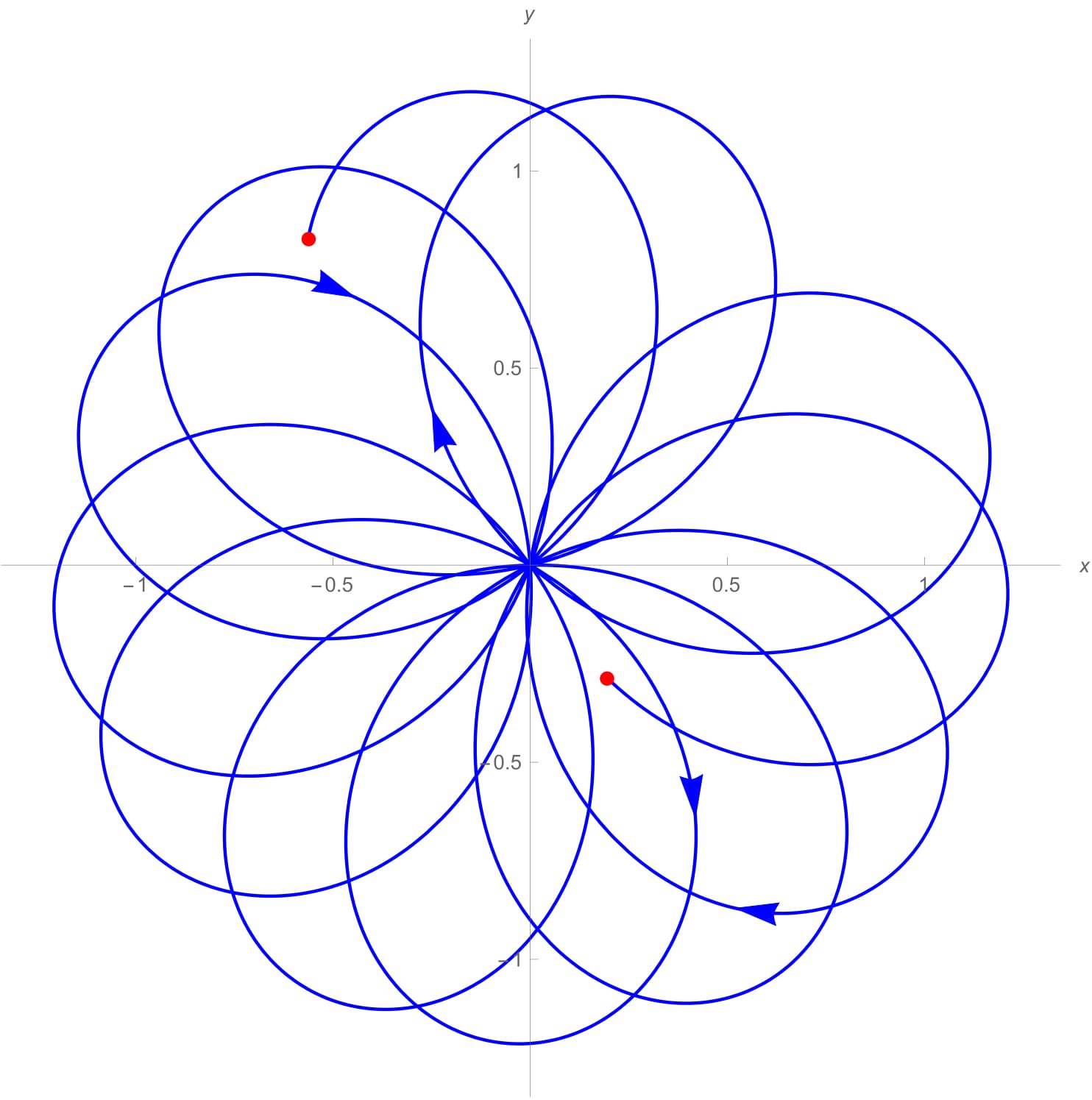
Here some trayectories in $\mathbb{R}^2$ for different parameters ($0\leq t \leq 4\pi)$:
The question is what does the last term in the above Lagrangian mean: (energy dimension)
$\hspace{6cm} E=m\dot{\textbf{r}} \cdot (\vec{\omega}\times \textbf{r}) $