Comparing the plots for the total (inelastic) cross sections as a function of the centre of mass energy for $pp$ and $e^+e^-$ collisions:
one notes that the trend at high energy is opposite: the $pp$ cross section increases while the $e^+e^-$ decreases. Is there a (simple) explanation for this?
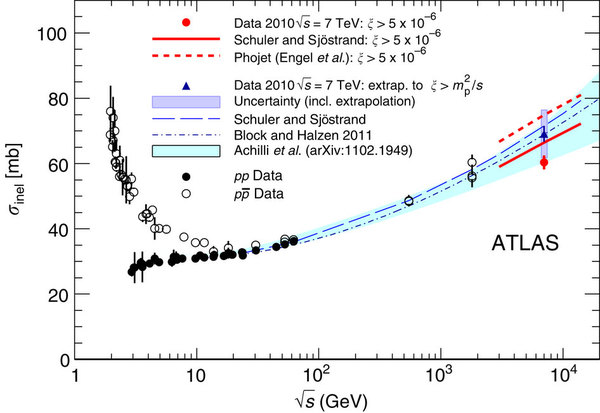
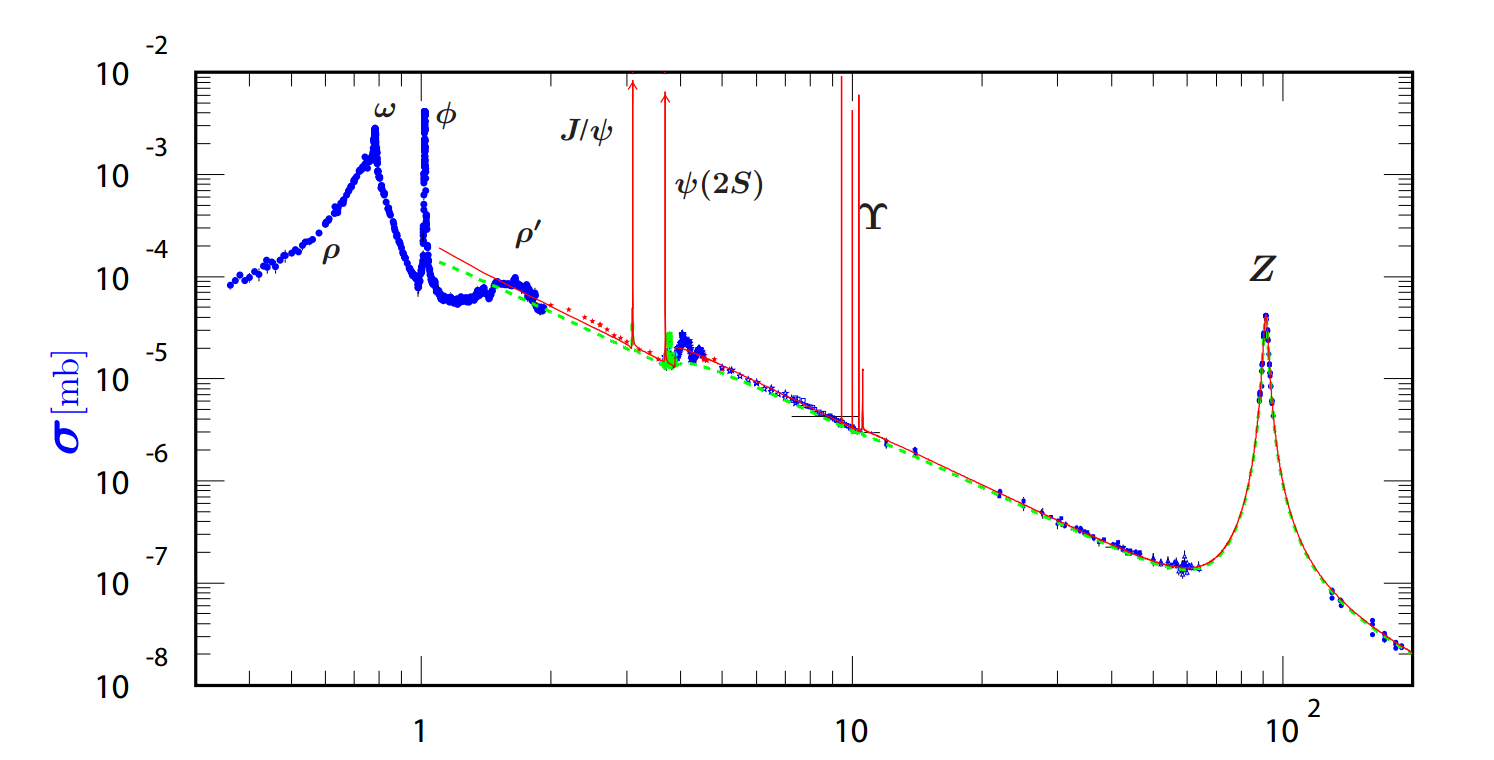
There are two different answers: the growth of the PDF's, or the Regge trajectory of the Pomeron.
Roughly, the reason why the proton-proton cross section grows with Mandelstam $s$ is because the parton density functions (PDF's) of a proton, in particular it's gluon PDF, grows faster than $s$, thus outcompeting the natural fall off rate of $\frac{1}{s}$ of a two particle cross section. The growth of the electron PDF's on the other hand do not outcompete the $\frac{1}{s}$ falloff rate.
A completely different answer comes from a different framework for QCD called Regge kinematics. The high energy cross section of QCD is controlled by a reggeon called the pomeron. Because the Regge trajectory has a positive slope, the cross section rises. In a sense, this is just repeating the statement that the cross section rises, but one can calculate the slope of the Regge trajectory of the Pomeron (see the BFKL equation) and find that it is indeed positive.
If you don't know what a parton density function is: The parton density function of a hadron is a measure of how many particles it contains. You might complain that a proton only has three particles, two up quarks and a down, but this description is only accurate at low energies and is mainly useful for hadron spectroscopy (classifying the hadrons). When calculating a cross section in QCD, the framework is such that you presume that there is some likelihood of finding any particle inside of a proton, for example $P_{\gamma}(E_{\gamma})$ may denote the likelihood of finding a photon of energy $E$ inside of the proton. Without going into too many details, the intuition is obvious, the more particles inside of a hadron, the greater the cross section.
