How does Ohm's law apply to superconductors?

Question

As radio amateurs we've all learned the various relationships of power, voltage, current and resistance as expressed in Ohm's Law.

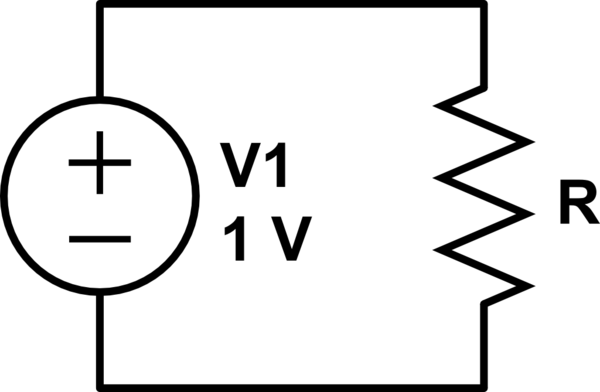
My question relates to the following simple circuit showing an ideal power source capable of providing infinite current with no drop in the voltage it supplies. The resistor is made of a superconductor material. Let's ignore the effects of current density on superconductors for now. When the temperature of the superconductor material is lowered to the point at which its resistance drops to zero, how much power is being dissipated by the resistor?

Is it given by...

(Door #1) $P = I^{2}R\ = I^{2} \cdot 0 = 0 Watts$

or is it given by...

(Door #2) $P = E^{2}/R = E^{2}/0 = \infty Watts$

or is it...

(Door #3) some other equation or relationship?

I've got to know the answer before I invest in converting all my shack's wiring to superconductors: if there's a short circuit will there suddenly be 0 Watts or $\infty Watts$ that will turn my neighborhood into an unsightly cinder?!

---

Don't get too hung up on the mathematics. This question is related to physics. I'll accept any answer that explains which "door" is the right one, and why.

For those who doubt that the problem can be approximated mathematically, consider that it can be investigated experimentally. Imagine assembling a huge bank of fully-charged super-high capacitance capacitors, and connecting them via high-current wires to either end of a big thick hunk of high critical current density superconductor. We will, of course, select capacitors and connecting wires (and switch if desired) such that their total internal resistance will limit the current density through the superconductor to just below its critical current density so that it remains in its superconductive state throughout the experiment - and also keeps the capacitors and wires from melting. Just for the sake of argument let's say we generate a few million amps across the superconductor if only for a few milliseconds. That is a bit shy of infinite current, but quite enough to get a good idea as to which solution applies to the superconductor's behavior.

If you like the answer provided behind door #1 then you should be happy to stand next to experimental apparatus while the experiment is conducted.

If you like the answer provided behind door #2 then you probably want to remove yourself to the next county before the switch is thrown.

If you like the answer provided behind door #3 then who knows?

Whichever door you prefer, please explain the physics behind your selection.

Since the circuit model can be approximated experimentally, we know that it must be possible to derive a mathematical approximation by simply modeling the experiment.

Answer 1

… an ideal power source capable of providing infinite current with no drop in the voltage it supplies. … Let's ignore the effects of current density on superconductors for now. …

In these phrases is the explanation for the contradictory possibilities you have computed: you have supposed an impossible circuit.

As a mathematical model, the behavior of your circuit is undefined; it is an inconsistent overdetermined system. There is no value of the current which satisfies the equations defining the ideal components as you have connected them.

As a physical system, when you close the circuit, the current will rise from zero (at a rate determined by the inductance of the system, which depends on its shape and size) and quickly reach a limit where either

• P < ∞: the source is no longer operating as a voltage source, as its output voltage lowers (either due to internal resistance or deliberate foldback),
• P > 0: the “resistor” is no longer operating as a superconductor, as its maximum current density is exceeded,

or some of both effects at once. You cannot escape these limitations.

Answer 2

As Kevin Reid aptly explains, the circuit you have drawn is not realizable. But, let's take the closest physical thing you could build, assuming:

• your voltage source can supply enough energy that we don't hit its limits
• like all physical things, this apparatus has non-zero size

Then, the circuit you actually built is this:

Because you are using superconductors, there is no resistance. However, there is inductance, because a superconductor can not be made with zero size, and any electrical circuit with non-zero area (that's all of them, I think) has some inductance. The inductance of your circuit is determined by its area, and the magnetic permeability of the stuff around it.

In this case, the current increases according to the definition of inductance:

$$ v = L \frac{\mathrm di}{\mathrm dt} $$

That is, current will increase linearly, at a rate determined the inductance. Along with the current, the magnetic field increases. You can run this for some finite amount of time, as long as your voltage source remains able to supply enough current.

As always, power is the product of voltage and currend ($P=IE$), which can be used to calculate the power required from the voltage source, or the power absorbed by the inductor. For this circuit they are the same for either component, and since voltage is constant and current is increasing, power increases linearly with time. However, the inductor does not get hot as a resistor would, because the electrical energy is being stored in a growing magnetic field, not dissipated as heat as in a resistor. If your voltage source is anything like the ones in my shack, it's probably getting hot due to inefficiencies.

If you run this for a while, you can get a really strong magnet. If you run it until your voltage source can't supply any more current, then swap the voltage source for a superconducting short, then the magnetic field and the current persists, and you are well on your way to building an MRI scanner.

The real problem to consider for your shack is not what happens when you replace all your conductors with superconductors, but what happens when they stop being superconductors. You have all that energy stored in the inductance which must go somewhere, eventually.

Answer 3

As radio amateurs we've all learned the various relationships of power, voltage, current and resistance as expressed in Ohm's Law

Ohm's law is:

$$ E = IR \tag{1} $$

This doesn't directly say anything about power. There is the related Joule's first law, which relates to electrical power converted to heat in resistive materials:

$$ P = I^2 R \tag{2} $$

Although this has a power term, it still isn't a definition of electrical power. There are many things which generate or consume electrical power but aren't resistive and don't get hot, or at least, not all their electrical power becomes heat. Consider: motors, antennas, or LEDs. Electrical power is just the product of current and voltage:

$$ P = IE \tag{3} $$

Joule's first law (equation 2) can be derived from this definition of electrical power and Ohm's law. Simply substitute $E$ in equation 3 with equation 1:

$$ P = I(IR) = I^2 R $$

Similarly, $P = E^2/R$ is the result of solving Ohm's law for $I$ and substituting.

However, these equations ($P=I^2 R$ and $P = E^2/R$) only calculate the electrical power in a resistance. Useful, because resistance is very common, but it is not universal. The only universal definition of electrical power is $P=IE$.

A superconductor has no resistance, so power can not be calculated by $P=I^2 R$ or $P = E^2/R$. As Kevin Reid explains, the circuit you have presented can't be solved. You must incorporate in your circuit the things that will necessarily exist in any realizable circuit, such as inductance (as I explain in my other answer) or series resistance due to non-superconducting components (as you elude to in your bank of capacitors experiment). Then you can solve for current and voltage, multiply them, and calculate power.

So the answer is door #3: some other relationship. That relationship is $P = IE$, with the caveat that you must first make your circuit solvable.