What I am asking is this: Why can't a body be solid, then solid-ish, then solid-like, then liquid-like, then liquid-ish, then liquid, then vapor-like and then vapor? Why is there a rigid temperature boundaries between solid, liquid and vapor? Why doesn't water simply change "states" in a continuous manner?
-
$\begingroup$ "Why can't a body be solid, then solid-ish, then solid-like, then liquid-like, then liquid-ish, then liquid, then vapor-like and then vapor?" Actually it can --- this is often the case for mixtures, at least regarding the solid-liquid transition. But the question of why water and other pure substances don't behave this way is an interesting one, and actually quite difficult to answer. $\endgroup$– N. VirgoCommented Mar 15, 2014 at 10:59
-
$\begingroup$ vapor continually comes off any water surface. even ice vaporizes too, that is how clothes can be dried in very cold dry weather. It does not wait, just the functional dependence grows up to 100C maximum vaporization. $\endgroup$– anna vCommented Mar 15, 2014 at 11:36
-
$\begingroup$ What i infer from this page is this: when we transfer heat to a one unit liquid water, then instead of the entire unit to become vapor-like or vapor-ish, a little amount of the liquid absorbs ALL the heat available to jump to vapor state, and the remaining part of the unit liquid remains liquid, just because the state of being liquid and vapor is more favorable than any other phase that can be imagined in between. The heat transferred to a unit liquid does not makes it way to the entire unit uniformly... $\endgroup$– PremCommented Mar 16, 2014 at 5:06
-
$\begingroup$ You could also check out Andrews' experiment where in the graph of pressure versus specific volume, we observe a gas go through a phase of metastable equilibrium before being liquefied. In that metastable state the substance is neither gas nor liquid, but something intermediate. $\endgroup$– Pallavi RoyCommented Mar 19, 2014 at 7:50
4 Answers
The difference between solid and liquid lies in the atomic structure. Ice is crystalline (and therefore in an ordered state) while water has no such ordering. It is amorphous.
So the reason for the abrupt change in state is that something cannot be ordered and unorderd at the same time. Now you may say "Hey, why not have some regions that are orderes and connect to other regions, that are unordered?" That state exists, and is commonly known as wet snow with varying degrees of "wetness".
An edge cases I'd like to mention: Crystallization takes time. Glass and some thermoplastics are amorphous in their solid states, and subsequently do not exhibit a sharp change in properties but transit slowly from solid to liquid.
The distinction between liquid and gas phase does indeed vanish at some point, mostly at higher pressure. Beyond the so called critical point, the two states are indistinguishable. Therefore, it is possible to get from liquid water to vapor without a phase change.
In essence, abrupt phase changes from solid to liquid exist for materials that have a high tendece to form crystals. Most pure substances can be arranged in a regular manner, and therefore tend to form crystals. Glass (SiO2) is often mixed with other substances to hinder crystallization. You can make anything amorphous by cooling it rapidly.
The phase change from liquid to solid is less abrupt than you may think. It exists only for low pressure and low temperatures. How low, depends on the substance.
Most of the above holds true for any substance, not only water.
-
3$\begingroup$ But liquid and vapour are both amorphous, and yet liquids have a sharp boiling point. I also contest your claim that a material cannot be ordered and unordered at the same time. We commonly find systems described by an order parameter that can have a continuous range of values. $\endgroup$ Commented Mar 15, 2014 at 11:56
-
$\begingroup$ To extend on John Rennie's comment: In a common ferromagnet the phase transition is second order, the material is ordered and unordered at the same time, the length of the ordered regions changes with temperature continuously. Glasses on the other hand are a bad example because they do not exhibit a phase transition with long range order but belong to a different class of transitions. $\endgroup$ Commented Mar 15, 2014 at 23:07
-
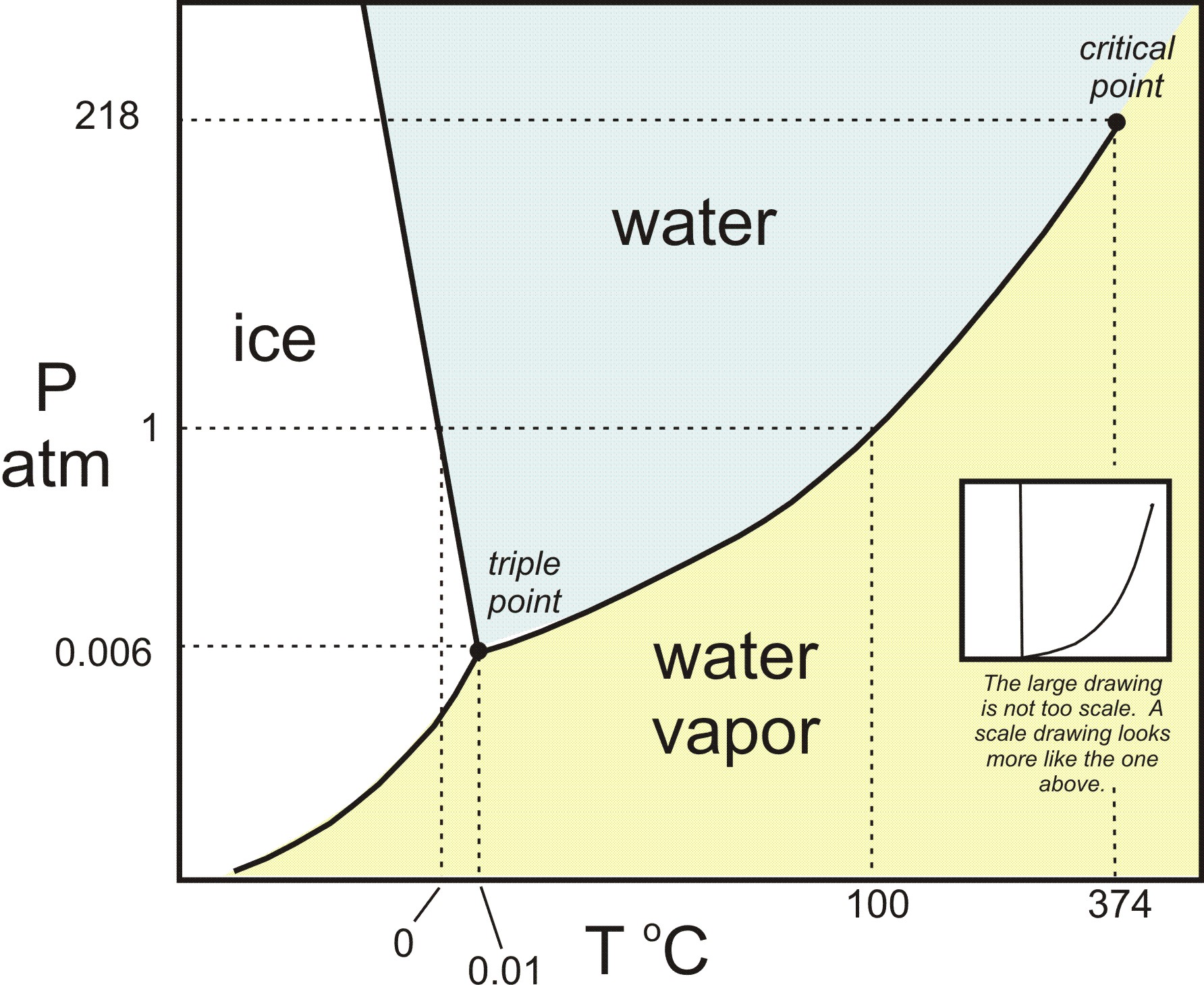
$\begingroup$ That chart cuts off the solid-liquid line. Do we not show the area where it meets the y axiz (i.e. 0 Kelvin) because we don't really know, or because textbooks are old, or another reason? Once any atoms get to 0 kelvin, they become solid. The line would be asymptotically vertical, and "inclusive" towards being solid rather than liquid. But what about very slightly above 0K? In that temperature region, what does the line look like when zoomed in? Linear as always? $\endgroup$ Commented Sep 15, 2014 at 0:18
-
$\begingroup$ The line never meets the y-axis. Wikipedia has a more comprehensive chart: en.wikipedia.org/wiki/Ice#mediaviewer/… $\endgroup$ Commented Sep 15, 2014 at 12:53
There are quite strong but short-range attractive forces which are keeping molecules in crystal mesh or in liquid. Strength and short range of the forces results in instability of “solid-ish”, “liquid-ish” or “vapour-like” form of material.
To illustrate the instability consider a thought experiment. We have a magnet and a piece of ferromagnetic metal. We put piece of metal on the table. When we approach with the magnet from the top there are three possible cases - distance between magnet and metal can be:
- far enough - there is no visible attraction
- too close - the piece of metal is lifted and clicks into the magnet
- some distance in between - the piece of metal is hovering between the table and the magnet (distance is being kept just right - not too far to fall off and not too close to be lifted up). As we can see this situation is quite difficult to maintain. “Solid-ish” or “liquid-ish” form of material corresponds to this case.
This (not completely accurate) analogy answers why there is no solid-ish form of water and no liquid-ish form of vapour under normal conditions (under atmospheric pressure).
However the question “why water waits for 100 degrees” remains. Our problem is that the water vapour is transparent to human eyes and we cannot distinguish it from the air. If we could see the water vapour and if we observe water being heated (and finally boiled) carefully enough we would see continuous (non-abrupt) changes. It turns out that the water does not wait for 100 degrees.
When water is being heated from 0 to 100 degrees (under atmospheric pressure) there is higher and higher concentration of a water vapour above the water level. There is 100% concentration at the boiling temperature. To describe the process nicely term “partial pressure” of a water vapour is being used.
Pressure of a water vapour at boiling temperature is sufficient to inflate small bubbles of air dispersed in the water - which is visible as boiling. Water gets additional surfaces through which it evaporates. When we heat the water more rapidly the additional surfaces are created more rapidly and evaporation gets faster. Evaporation cools the water down and its the reason why temperature stops at 100 degrees.
Now lets have a look at mechanism how water is being cooled down by evaporation. Temperature is related to average speed of molecules. Distribution of velocities is non-uniform. Molecules escaping the water are acting against short-range attractive forces trying to keep them in the water.
Only the fastest molecules manage to escape. When fastest molecules escape the average speed is lowered. So the water is colder. On the other hand the escaping molecules were being attracted back (during the escape) and therefore slowed down. Once the escaped molecules are in the vapour they are slow. The average speed of molecules of vapour is lowered. So the vapour is colder.
Fill a bowl with water, let it stand for a few days and then see how much water is left. Water vaporizer all the time depending on the conditions. Boiling means that vapor bells are created everywhere through the liquid. Below boiling temperature the vaporization only occurs at the boundary surface with the air.
According to John Rennie I might have misread the question, so I will add some in depth explanation. But as Raja is a first year high school student, which means 13 year of age in my country, I try to keep it simple. Of course ,age could be higher in India, and his basic knowledge be better than a 13 year old Dutch kid. Saw from Raja's profile his age is 17.
First we have to keep in mind the macro point of view versus the micro point of view, macro meaning matter, phase, density, temperature etc. Micro meaning molecules, forces, velocity, distance, energy etc. What happens with the molecules in the microworld determines the behavior of the matter in the macroworld. Molecules in matter have kinetic energy as well as potential energy. The kinetic energy of the molecules depends on the temperature of the matter. The potential energy depends on the distance between the molecules as well as on the attractive forces the molecules exerts on each other. These forces are usually greater as the distance between the molecules are smaller.
The forces are what determine the phase of the matter. If they are large enough the molecules are held in place relative to each other. This is a solid.
If the forces are lower the molecules can move relative to each other, but still stay with each other. This is a liquid. You can observe this behavior when you spill some water on the table. The water will spread, but not indefinitely. Als when two drops are close enough they will join into one drop.
In gaseous matter the forces between the molecules are very small and the molecules move freely about.
So to change phase of a matter the forces need to be lowered enough to let the molecules move relative to reach other. This is done by adding kinetic energy to the molecules, letting them vibrate in their place. If the velocity becomes big enough the molecules can move away from each other. Temperature is an indication of the average kinetic energy of the molecules. So to have all molecules move at high enough velocity the matter needs a minimum temperature. Increasing the distance between the molecules means work has to be done, as you want to move the molecules against the attracting forces. This will increase the potential energy of the molecules. At the same time the attracting forces are lowered.
When you heat matter the supplied energy is at first mostly used to enlarge the kinetic energy of the molecules, raising temperature. When the melting point is reached the supplied energy is mostly used to enlarge the potential energy of the molecules, keeping the average kinetic energy constant which we observe as a fixed melting point.
As I wrote at the beginning, water will vaporize at temperatures below the boiling point. This is because some molecules gain kinetic energy through collisions with neighboring molecules. The kinetic energy of the molecule is than converted in potential energy as it moves away from the other molecules. If the gained energy is high enough the molecule will move far enough to be considered free of the water. Since the average kinetic energy stays the same the temperature of the water does not change.
-
3$\begingroup$ I don't think this answers Raja's question. I think he's asking why there is a first order phase transition between ice-water and water-steam. This strikes me as a surprisingly subtle question and one that isn't trivial to answer. $\endgroup$ Commented Mar 15, 2014 at 8:31
-
$\begingroup$ @JohnRennie but it does address a misconception in the question, where the OP thinks that below boiling temperature there's no vaporization. $\endgroup$– RuslanCommented Mar 15, 2014 at 9:18
-
1$\begingroup$ @Ruslan: that could have been done in a comment $\endgroup$ Commented Mar 15, 2014 at 9:19
-
$\begingroup$ Extended the answer after John Rennies comment $\endgroup$ Commented Mar 15, 2014 at 11:25
There is a continuous range of behaviors, as one raises the thermal energy in a material. However, it is a very discrete continuous range =) It's like a staircase. In theory the stairs can move and bend under the weight of your foot; in practice, we treat them as hard rigid steps.
For an analogy, let's start with a deck of cards, sorted and in-suit. This is a highly ordered system, and we are going to claim it relates to ice. In ice, the intermolecular forces are tremendously strong compared to the thermal energy. It holds everything in place. In our card analogy, we can compare it to some very thick inflexible cards, like they were made out of iron. If you try to shuffle them with a riffle shuffle, you find you cannot. The cards simply refuse to move, no matter how many times you try to "shuffle" them. Likewise, the molecules in ice are locked such that is is extraordinarily unlikely for thermal energy to move a molecule to a new place. This makes it rigid.
Now, let's turn up the heat. In this card analogy, we'll make the cards a little more flexible. In the ice, we're adding more thermal energy, letting things bounce around more quickly. At some point, the cards bend enough to permit a shuffle, just as the intermolecular forces are finally overwhelmed by some of the thermal motion, permitting movement.
Now if you shuffled a stiff deck once, it wouldn't change the order by that much. There's a reason you have to shuffle a deck multiple times before dealing it. Nature... never shuffles once. Thermal randomness is a very fast operation, like shuffling the deck a thousand times. So once you have enough flexibility in the cards to overcome the stiffness preventing a shuffle, they get really shuffled, really fast. There is indeed a state of matter that is solid-ish, a partially shuffled deck, but it lasts for only a very brief period of time as the increasing mobility permits more high energy molecules to collide with the solid ones, jostling them. Very quickly, the assumptions of rigid objects fail us. We see motion and fluidity.
So we can shuffle the deck for a while. But the molecules are still held together by the hydrogen bonds. No atoms can escape. Well, every now and then, one escapes -- vaporizing. It's really embarrassing to have a card escape your deck while shuffling, but it happens if you're shuffling thousands of times. But what if we make the deck more and more flexible, and storing more and more of the energy in the springiness of the cards? At some point, your skill at shuffling cards fails you, the cards go everywhere, and you play a game of 52 card pickup.
In the water, this happens at a point where the intermolecular forces can no longer force the water to stay in one fluid mass, held down by gravity. The energy of the atoms is so great, that they can escape, and they can do so at any point.
Now why are these edges so crisp? We are talking about thermal effects, so there's a degree of randomness in the explaination. Each molecule has a chance to have a velocity high enough to start acting more fluid, or more gassious. So there is, in theory, a probability density function which describes which portion of the molecules are acting ice like, which are acting water like, and which are acting gas like.
However, there's a lot of atoms. And when you have a lot of them, the central limit theorem starts to come into play. It says that if you sum up enough draws from a random variable with a finite variance $\sigma^2$, the sum gets closer and closer to looking like a normal distribution with variance $\frac{\sigma^2}{\sqrt N}$, where $N$ is the number of draws.
Take enough draws, like the 33,460,000,000,000,000,000,000,000 atoms in a single cubic centimeter of water and this random variable's variance gets really really tight. So tight that it can be hard, or even impossible, to measure them amidst other effects (like you breathing on the water, or any ions dissolved). So for practical purposes, we treat it as-if there was no variance. We treat it as if there were simply 3 (or four) discrete states of matter.
Incidentally, you'll come across this in quantum mechanics, later. Someone will tell you that light is both a wave and a particle, and you'll scratch your head, trying to make sense of that, and trying to tie it into your reality. They'll tell you that the behaviors in the QM world are random, after you spend years learning nice easy deterministic physics in school. Just remember, that your reality consists of billions and billions of photons, and when you have enough of them, the central limit theorem starts to take effect, and all of those complicated randomnesses turn into something much simpler -- classical mechanics, with its nice deterministic behaviors.
Okay, maybe not that much simpler. Water is still complicated. But you get the idea!
