I will try to answer these questions from different views.
Macroscopic view
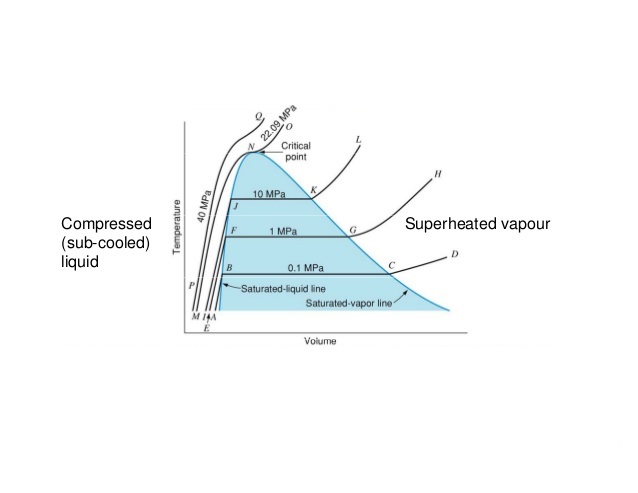
The "quantitative" rather than qualitative difference in a liquid-gas phase transition is due to the fact that the molecules arrangement does not change so much (there is no qualitative difference) but the value of the compressibility changes a lot (quantitative difference). This can be easily seen in the Van der Waals isotherms below the critical temperature,
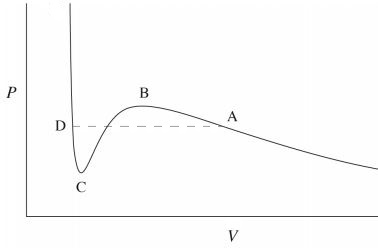
The phase transition occurs at the dashed line $AD$. For volumes smaller than $V_D$, the high slope of the curve means that one needs a huge amount of pressure in order to decrease a small amount of volume. This characterizes a liquid phase which has a very low compressibility. For the slope is much lesser and the compressibility is high, which characterizes a gas. In between $V_D$ and $V_A$ there is a mixed phase characterizes by a divergent compressibility, i.e. the volume changes at constant pressure.
Above the critical temperature there is no longer such a radical change in the compressibility. The Van der Waals isotherm is the following
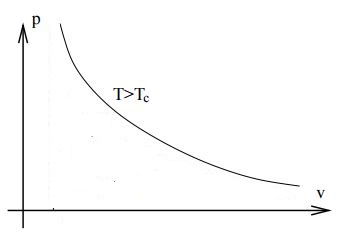
As you mentioned the density continually increase with the pressure. You can also see from the Van der Waals equation, when written as
$$p=\frac{NkT}{V-Nb}-a\frac{N^2}{V^2},$$
that at very high temperatures it behaves like
$$p\rightarrow \frac{NkT}{V-Nb},$$
which is not qualitatively different from an ideal gas isotherm. There is no liquid phase.
Microscopic view
Let us consider a substance below its critical temperature. After a phase transition from gas to liquid, a meniscus (interface) appears between the liquid portion and a vapor (gas) portion which is present due to the kinetic distribution of velocities. The vapor has much smaller density so a molecule in the bulk of the liquid have more bonds than a molecule in the surface (interface). Each bond has a negative binding energy (bonded states) so the molecules in the surface have an excess of energy.
This gives rise to a (positive) surface energy density which is nothing but the surface tension of the interface. When we increase the temperature, the vapor density increases and at some point it equals the liquid density. At this point the number of bonds for the molecules in the bulk and in the surface equals so that there is no surface tension. This means there is no meniscus and no phase transition. There must be a critical point therefore.