17
$\begingroup$

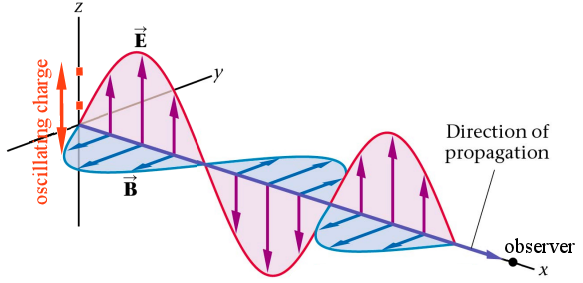
enter image description here

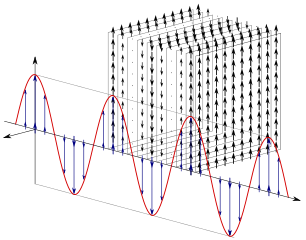
In most physics textbooks, the oscillating electric and magnetic fields generated by a moving photon are depicted as above.

In this image, the photon is moving along x axis, the electric field is oscillating long z axis ( in z-x plane), and the magnetic field is oscillating along y axis (in x-y plane).

I am having two fundamental problems with this picture.

  1. Does the electric field oscillate strictly in the x-z plane only? Does it completely vanish just a miniscule distance away from this plane? This does not make sense intuitively.

  2. If there is just a single photon moving along x axis, the electric and magnetic fields must die out after the photon has passed. This means that the amplitude of E and B at a given point on x axis must fade out as the photon goes away. So how does this picture change in the case of a single moving photon?

Improve this question
$\endgroup$
1
  • 5
    $\begingroup$ Friendly reminder that comments should be used to improve the question, not to provide brief answers. $\endgroup$
    – rob ♦
    Commented Jul 5, 2021 at 21:58

1 Answer 1

Reset to default
22
$\begingroup$

The picture does not show the fields of a moving photon. There is no such entity in QED. There is a quantized field whose excited levels can be classified as photons. A coherent superposition of photons may result in a macroscopic electromagnetic field.

What that picture represents are the macroscopic electric and magnetic fields in a plane-wave solution of the classical Maxwell's equations. The picture requires careful interpretation. It is not a kind of snapshot of the spatial features of the electromagnetic wave. It should be interpreted as a 2D projection of a 3D plot representing the values of the ${\bf E}$ and the ${\bf B}$ fields as a function of the x coordinate in a linearly polarized plane wave. Such plane wave in 3D starts from $x=-\infty$ and arrives at $x=+\infty$. Vectors ${\bf E}$ and ${\bf B}$ are the same in every plane perpendicular to the $x-$axis, at every distance from the $x-$axis, while their size and orientation periodically vary as functions of $x$. A more faithful representation of the situation described by the plane wave field may be obtained from this Wikipedia image:

$\qquad\qquad\qquad\qquad\qquad$Representation of 3D wave nature of electromagnetic field

Of course, such an infinite object cannot exist. However, real electromagnetic waves can always be represented by a superposition of such unphysical building blocks (Fourier analysis).

There is no possibility of associating such a plane wave to a single photon. One reason is that there is an uncertainty relation between the phase and the number of photons. Therefore, if the number is well defined (one photon), there will be maximum uncertainty for the phase. The picture shows an oscillation with a well definite phase.

Improve this answer
$\endgroup$
11
  • $\begingroup$ Good answer! Defining a "phase" observable can be tricky, but we can avoid that issue by saying it like this: The number-of-photons observable does not commute with the electric or magnetic field observables, so a photon cannot have well-defined values of the EM fields. $\endgroup$
    – Chiral Anomaly
    Commented Jul 5, 2021 at 21:33
  • $\begingroup$ <quote> " Vectors E and B are the same in every plane perpendicular to the x−axis, at every distance from the x−axis " - Giorgio, so are you saying that E and B move out in concentric circles from origin, like the ripples on the surface of water? $\endgroup$
    – dionysus
    Commented Jul 5, 2021 at 21:42
  • $\begingroup$ @dionysus No, E field is uniform in y direction and B is uniform in z direction. $\endgroup$
    – Ben51
    Commented Jul 5, 2021 at 22:05
  • $\begingroup$ @dionysus I have added a link to a picture providing a representation of the field of a linear polarized plane wave. $\endgroup$
    – GiorgioP-DoomsdayClockIsAt-90
    Commented Jul 5, 2021 at 22:34
  • 2
    $\begingroup$ @dionysus I would add that you shouldn't be discouraged by the complexity of the photon concept. Indeed, it is quite a sophisticated concept, but it can be mastered by studying physics step by step. Unfortunately, popsci has the tendency to present complex concepts in an apparently familiar way, at the risk of paving the way to misconceptions. $\endgroup$
    – GiorgioP-DoomsdayClockIsAt-90
    Commented Jul 6, 2021 at 5:16

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.