thin film interference of light

Question

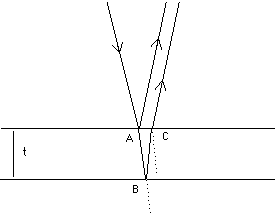
In a thin film interference (reflective system) I know that condition for maxima is $$2\mu t\cos(r)=(2n\pm 1)\frac{\lambda}{2}$$ and for minima is $$2\mu t\cos(r)=n\lambda$$ and for transitive system it's just the opposite. but what happens if then film is very small such that $$\lim_{t \to 0}$$ i.e. thin film is too thin? My teacher told my that condition for minima is satisfied because then $\delta x = \lambda /2$ and hence film appears dark. How is this possible? and similarly what happens of film in too thick ? I am guessing interference does't happen then , but what would be explanation for it ?

Answer 1

At the reflection in an interface from low to high refractive index, a phase shift of $\pi/2$ occurs; no such phase shift occurs on the second interface (high to low). As a consequence, for sufficiently thin films, there is indeed destructive interference- so your very thin film looks dark. You can sometimes see this on soap bubbles just before they pop - the go from shiny and colorful to (patches of) dull and colorless, usually at the top (as liquid is pulled to the bottom of the bubble by gravity).

An explanation of the phase shift is given in this answer - note that the premise of that specific question is backwards but the answer is correct...

Answer 2

If the film is thinner than half a wavelength then there is no thin-film interference (until you get into complex surface plasmon effects).

Thin-film interference works with thick films so long as they are multiples of half a 1 or 1/2 a wavelength. The main practical difficulty is making thick films that are uniform refractive index, and flat on the top.

You can demonstrate interference fringes in air with relative large distances https://en.wikipedia.org/wiki/Newton%27s_rings