Here are some depictions of electromagnetic wave, similar to the depictions in other places:
Isn't there an error? It is logical to presume that the electric field should have maximum when magnetic field is at zero and vise versa, so that there is no moment when the both vectors are zero at the same time. Otherwise one comes to a conclusion that the total energy of the system becomes zero, then grows to maximum, then becomes zero again which contradicts the conservation law.
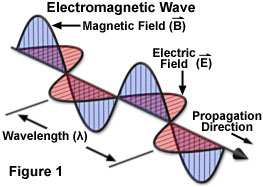
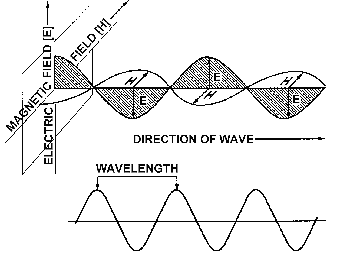
The depictions you're seeing are correct, the electric and magnetic fields both reach their amplitudes and zeroes in the same locations. Rafael's answer and certain comments on it are completely correct; energy conservation does not require that the energy density be the same at every point on the electromagnetic wave. The points where there is no field do not carry any energy. But there is never a time when the fields go to zero everywhere. In fact, the wave always maintains the same shape of peaks and valleys (for an ideal single-frequency wave in a perfect classical vacuum), so the same amount of energy is always there. It just moves.
To add to Rafael's excellent answer, here's an explicit example. Consider a sinusoidal electromagnetic wave propagating in the $z$ direction. It will have an electric field given by
$$\mathbf{E}(\mathbf{r},t) = E_0\hat{\mathbf{x}}\sin(kz - \omega t)$$
Take the curl of this and you get
$$\nabla\times\mathbf{E}(\mathbf{r},t) = \left(\hat{\mathbf{z}}\frac{\partial}{\partial y} - \hat{\mathbf{y}}\frac{\partial}{\partial z}\right)E_0\sin(kz - \omega t) = -E_0 k\hat{\mathbf{y}}\cos(kz - \omega t)$$
Using one of Maxwell's equations, $\nabla\times\mathbf{E} = -\frac{\partial \mathbf{B}}{\partial t}$, you get
$$-\frac{\partial\mathbf{B}(\mathbf{r},t)}{\partial t} = -E_0 k\hat{\mathbf{y}}\cos(kz - \omega t)$$
Integrate this with respect to time to find the magnetic field,
$$\mathbf{B}(\mathbf{r},t) = -\frac{E_0 k}{\omega}\hat{\mathbf{y}}\sin(kz - \omega t)$$
Comparing this with the expression for $\mathbf{E}(\mathbf{r},t)$, you find that $\mathbf{B}$ is directly proportional to $\mathbf{E}$. When and where one is zero, the other will also be zero; when and where one reaches its maximum/minimum, so does the other.
For an electromagnetic wave in free space, conservation of energy is expressed by Poynting's theorem,
$$\frac{\partial u}{\partial t} = -\nabla\cdot\mathbf{S}$$
The left side of this gives you the rate of change of energy density in time, where
$$u = \frac{1}{2}\left(\epsilon_0 E^2 + \frac{1}{\mu_0}B^2\right)$$
and the right side tells you the electromagnetic energy flux density, in terms of the Poynting vector,
$$\mathbf{S} = \frac{1}{\mu_0}\mathbf{E}\times\mathbf{B}$$
Poynting's theorem just says that the rate at which the energy density at a point changes is the opposite of the rate at which energy density flows away from that point.
If you plug in the explicit expressions for the wave in my example, after a bit of algebra you find
$$\frac{\partial u}{\partial t} = -\omega E_0^2\left(\epsilon_0 + \frac{k^2}{\mu_0\omega^2}\right)\sin(kz - \omega t)\cos(kz - \omega t) = -\epsilon_0\omega E_0^2 \sin\bigl(2(kz - \omega t)\bigr)$$
(using $c = \omega/k$) and
$$\nabla\cdot\mathbf{S} = \frac{2}{\mu_0}\frac{k^2}{\omega}E^2 \sin(kz - \omega t)\cos(kz - \omega t) = \epsilon_0 \omega E_0^2 \sin\bigl(2(kz - \omega t)\bigr)$$
thus confirming that the equality in Poynting's theorem holds, and therefore that EM energy is conserved.
Notice that the expressions for both sides of the equation include the factor $\sin\bigl(2(kz - \omega t)\bigr)$ - they're not constant. This mathematically shows you the structure of the energy in an EM wave. It's not just a uniform "column of energy;" the amount of energy contained in the wave varies sinusoidally from point to point ($S$ tells you that), and as the wave passes a particular point in space, the amount of energy it has at that point varies sinusoidally in time ($u$ tells you that). But those changes in energy with respect to space and time don't just come out of nowhere. They're precisely synchronized in the manner specified by Poynting's theorem, so that the changes in energy at a point are accounted for by the flux to and from neighboring points.
There is no contradiction with conservation law, you are just applying it wrongly. To do it right, you have to consider a small closed region and check whether the variation of energy inside this domain is the same as (minus) the energy flux through its boundaries. In the case of a electromagnetic wave, when the energy density decreases inside the region, it just means that the energy has left the region you are considering.
In a (small) vicinity of any given point the instantaneous total energy $u=u(t)$ varies in time. The average total energy $\langle u\rangle=\frac{E_0^2}{2c \mu_0}$ does not:
$$\frac{d \langle u\rangle}{dt}=0.$$
Thus, energy is conserved for any small vicinity for any point.
Look at the original, Heinrich Hertz, 1889! If E=B=0 on a plane, S=0 => no energy Transport through that plane. I agree with Annix: "The pictures may be depicting a static wave, but certainly, not a propagating wave."
Without the detailed mathematics you need to use is Maxwell's equations, for example
$$\nabla \times \mathbf E = -\dfrac{\partial \mathbf B}{\partial t}$$
and a sketch of an electromagnetic wave travelling in the positive z-direction.
In simple terms the equation tells you that the rate of change of the electric field with position in the z-direction is equal to the rate of change of flux with time.
Consider position $L$ with the electric field and the magnetic field being at maximum positive values.
The gradient of the electric field in the z-direction is zero.
The sketch shows the magnetic field at a slightly later time with the magnetic field hardly changing hardly at all and in the limit not at all.
So you have $0$ (gradient of the electric field equal to $0$ (minus rate of magnetic field).
At this position the magnetic field is at a maximum but it is the rate of change of the magnetic field which is important.
Consider position $M$ where the gradient of the electric field in the z-direction is at a maximum magnitude but negative.
This is where the magnetic field is changing most rapidly with time.
Even though the magnetic field/flux is zero at this position it is the rate of change of magnetic field which is important and note that it is a positive increase.
With the rate of change of magnetic field a maximum multiplying it by minus one equates it to the maximum negative value of the rate of change with position in the z-direction of electric field.
A similar analysis will show that the sketch graph is consistent with another of Maxwell's equations.
$$\nabla \times \mathbf B = \mu_0 \epsilon_0 \dfrac{\partial \mathbf E}{\partial t}$$
There is no standard answer to this question, it involves a dark cloud in the physical world: wave particle duality. According to classical electromagnetic theory, electromagnetic waves naturally transmit energy. Electromagnetic waves must be active power waves in order to transmit energy, and the electric and magnetic fields of these waves must be in phase. Only in phase waves can carry energy. This is Plan 1.
But in quantum mechanics, energy is clearly carried by particles, while in electromagnetic theory, energy is carried by photons. What should we do? Let the electromagnetic wave be a probability wave. And say that this wave can collapse. The wave collapses to the point where the particles are absorbed, which is the absorber atom. On the screen is a dot. This is Plan 2.
Actually, neither of the above solutions is good. The best approach would be to establish a theoretical wave with reactive power, so that the wave does not transfer energy. Then, we can establish a theory of photons, where energy is transferred by photons. This is Plan 3.
How to establish a photon theory? Photons are actually an energy flow (momentum flow), which is a point at both the starting and receiving points. This shape of energy flow needs to be composed of waves emitted by the light source and waves emitted by the light sink. The waves emitted by the light source are ordinary retarded waves, while the waves emitted by the light sink are leading waves. Leading waves are waves that violate causal relationships, and the time in the wave is negative. The theory containing advanced waves was first proposed by Dirac in 1939 to explain the problem of self force. Later, Wheeler and Feynman developed it into the absorber theory in 1945. On this basis, Cramer proposed the interpretation of quantum mechanics trading in 1986. Retarded waves and leading waves can form a mutual energy flow, which is like photons and can transfer energy. In this way, electromagnetic waves do not need to transmit energy.
Electromagnetic waves do not transmit energy, they are reactive power waves that possess the characteristics of probability waves in quantum mechanics. Not transmitting energy. The electric and magnetic fields of this wave maintain a 90 degree phase difference.
Therefore, the core of the problem is whether energy is transmitted by photons or waves. If energy is transmitted by waves, then the waves must be of active power, with the electric and magnetic fields in phase. If energy is transmitted by particles, such as photons. So the wave must be probabilistic, or it must be reactive power, and the wave does not transfer energy. Maintain a 90 degree phase difference between the electric and magnetic fields!
What we're talking about here is not a standard answer, it's just my own opinion, not necessarily correct. For reference only.
