Relationship between the material properties of an edge and the fringes behind this edge

Question

The double-slit experiment shows fringes on a screen. Closing one of the slits there is still an interference pattern on the screen behind the slit. Making the slit wider we still see fringes between the shadows and the exposed area. Even with single photons or electrons. So a single edge is enough to get an intensity distribution on a screen.

On the other hand, using a polarisation filter it is possible to let through about 50% of the light (for equally distributed electric fields of the involved photons). Using a second filter, 90° rotated to the first, no light (of suitable wavelength) goes through.

The amazing fact is that using a third filter between the other two - best under 45° - some light goes through. That means that there has to be an influence of of the slits. The slits rotate the photon's electric field. But slits are made from edges and an edge (a term from geometry) in reality is some material. So to be precise, there has to be an interaction between the photons and the material of the edges.

In the experiment with electrons an electrostatical potential changes the fringes dimensions:

This pictures of the intensity distributions were made by G. Möllenstedt(not available in the English Wikipedia) and H. Düker in a biprisma experiment:

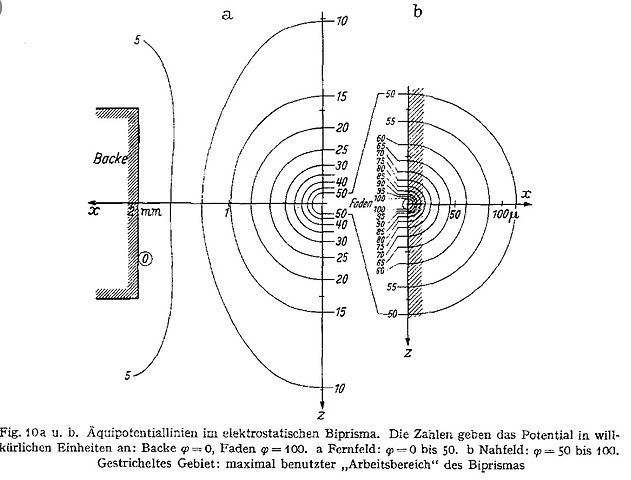
And it was given an explanation by the help of an electrolytic trough model (Elektrolytischer Trog), why the intensity distribution changes:

According to Möllenstedt, Düker, Beobachtungen und Messungen an Biprisma-Interferenzen mit Elektronenwellen, Zeitschrift f. Physik, Band 145, 1956, S. 377

Does in an experiment with light

• the use of different materials of the slit plate or
• the use of a material with different temperatures
• or different electrostatic potentials

changes the fringes dimensions (widths and positions) too?

Answer 1

I think that here there are two different questions.

First of all, polarizers are not materials with slits in a given direction (it seems to me that you are suggesting it), at least not in the optical regime, they are materials that interact with light in such a way that they let pass light with a certain kind of polarization. Therefore in the experiment with three polarizers what is happening is: let me call $\hat{x}$ and $\hat{y}$ the basis of the transverse plane, therefore after the first polarizer we would have a field with polarization $\vec{E}_1 = E_0 \hat{y}$, the polarizer at $\theta=45^o$ would let pass the componet of this field on its axis, that it would be the result of rotating with this angle the intial basis, in other words in the second polarizer basis' $\vec{E}_2^{rot} = E_0 \cos{45^o} ~ \hat{y}^{rot} $, transforming back again in the intial basis we would have $\vec{E}_2 = E_0 \cos{45^o} ( \cos{45^o} \hat{x} + \sin{45^o} \hat{y}) $, since the third polarizer is in the $\hat{x}$ axis its output would be the x component of $\vec{E}_2$, i.e. $\vec{E}_3 = (E_0/2) \hat{x}$.

To sum up, the polarizer in the middle of the other two rotates the initial polarization plane of the wave by taking its components in a rotated basis, and therefore permits that there would be a component normal to the original polarization plane, which would not happen otherwise.

About the two slit experiment, as you suggest different materials produce different results, it you heat up the air a lot you will see changes because of the change of the refractive index, but if you ask about changes only in the material, I would say that the only changes that could affect the experiment (apart from modifying the slits) would be to use materials with different transparency, because if you let pass a percentage of the light that would be blocked by a metalic surface otherwise, you will get interference not only between the light coming from the two slits but also with the background light that has passed through the areas of the material that were 100% reflective before.