How can magnets be used to pick up pieces of metal when the force from a magnetic field does no work?

Question

I learned that the force from a magnetic field does no work. However I was wondering how magnets can be used to pick up pieces of metal like small paperclips and stuff. I also was wondering how magnets can stick to pieces of metal like a refrigerator?

Answer 1

The Lorentz force $\textbf{F}=q\textbf{v}\times\textbf{B}$ never does work on the particle with charge $q$. This is not the same thing as saying that the magnetic field never does work. The issue is that not every system can be correctly described as a single isolated point charge

For example, a magnetic field does work on a dipole when the dipole's orientation changes. A nonuniform magnetic field can also do work on a dipole. For example, suppose that an electron, with magnetic dipole moment $\textbf{m}$ oriented along the $z$ axis, is released at rest in a nonuniform magnetic field having a nonvanishing $\partial B_z/\partial z$. Then the electron feels a force $F_z=\pm |\textbf{m}| \partial B_z/\partial z$. This force accelerates the electron from rest, giving it kinetic energy; it does work on the electron. For more detail on this scenario, see this question.

You can also have composite (non-fundamental) systems in which the parts interact through other types of forces. For example, when a current-carrying wire passes through a magnetic field, the field does work on the wire as a whole, but the field doesn't do work on the electrons.

When we say "the field does work on the wire," that statement is open to some interpretation because the wire is composite rather than fundamental. Work is defined as a mechanical transfer of energy, where "mechanical" is meant to distinguish an energy transfer through a macroscopically measurable force from an energy transfer at the microscopic scale, as in heat conduction, which is not considered a form of work. In the example of the wire, any macroscopic measurement will confirm that the field makes a force on the wire, and the force has a component parallel to the motion of the wire. Since work is defined operationally in purely macroscopic terms, the field is definitely doing work on the wire. However, at the microscopic scale, what is happening is that the field is exerting a force on the electrons, which the electrons then transmit through electrical forces to the bulk matter of the wire. So as viewed at the macroscopic level (which is the level at which mechanical work is defined), the work is done by the magnetic field, but at the microscopic level it's done by an electrical interaction.

It's a similar but more complicated situation when you use a magnet to pick up a paperclip; the magnet does work on the paperclip in the sense that the macroscopically observable force has a component in the direction of the motion of the paperclip.

Answer 2

Although what Ben and others have said might be sufficient, I would like to state my point.

Consider a piece of conductor being lifted up by the magnetic force. The current is towards the right(with velocity $w$), and the magnetic field is going into the page. Hence, the magnetic force is upwards. Now, as the conductor moves up, it gains a velocity $u$ in the upward direction. Hence the magnetic force changes direction as shown in the figure, but the upward component remains the same.

Now, observe that the horizontal component of the magnetic force is acting against the current. To maintain the current, the battery responsible for the current does work against this force and is the source of work done.

A popular analogue in Classical Mechanics is that of the role of normal force in pushing a block upwards a slope. The normal force does no work but is required to move the block up the slope. It's role is simply to redirect $F_{mop}$ in the upward direction. This is exactly the role of magnetic force in lifting stuff.$

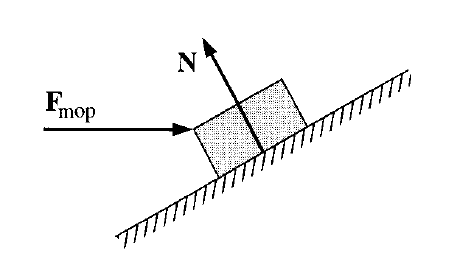
Source of Images and Knowledge:Griffiths' Introduction to Electrodynamics

Answer 3

The Lorentz force is the only force on a classical charged point particle (charge $q$ - see Ben Crowell's answer about nonclassical particles with fundamental magnetic moment such as the electron). The magnetic component of the Lorentz force $q \mathbf{v} \wedge \mathbf{B}$, as you know, is always at right angles to the velocity $\mathbf{v}$, so there is no work done "directly" by a magnetic field $\mathbf{B}$ on this charged particle.

However, it is highly misleading to say that the magnetic field cannot do work at all because:

1. A time varying magnetic field always begets an electric field which can do work on a classical point charge - you can't separate the electric and magnetic field from this standpoint. "Doing work" is about making a change on a system, and "drawing work from a system" is about letting the system change so that it can work on you. So we're always talking about a dynamic field in talking about energy transfer and in this situation you must think of the electromagnetic field as a unified whole. This is part of the meaning of the curl Maxwell equations (Faraday's and Ampère's laws). Moreover, once things (i.e. charges and current elements) get moving, it becomes easier sometimes to think about forces from reference frames stationary with respect to them: Lorentz transformations then "mix" electric and magnetic fields in a fundamental way.
2. A classical point charge belonging to a composite system (such as a "classical" electron in a metal lattice in a wire) acted on by the magnetic field through $q \mathbf{v} \wedge \mathbf{B}$ thrusts sideways on the wire (actually it shifts sideways a little until the charge imbalance arising from its displacement begets an electric field to support it in the lattice against the magnetic field's thrust). The magnetic field does not speed the charge up, so it does not work on the charge directly, but the sideways thrust imparted through the charge can do work on the surrounding lattice. Current elements not aligned to the magnetic field have torques on them through the same mechanism and these torques can do work. These mechanisms underly electric motors.
3. Another way to summarise statements 1. and 2. is (as discussed in more detail below) that magnetic field has energy density $\frac{|\mathbf{B}|^2}{2\mu_0}$. To tap the energy in this field, you must let the magnetic field dwindle with time, and electric field arising from the time varying magnetic field can work on charges to retrieve the work stored in the magnetic field.
4. The thinking of current elements shrunken down to infinitesimal sizes is a classical motivation for thinking about the interaction between magnetic fields and the nonclassical particles with fundamental magnetic moments, as in Ben Crowell's answer (I say a motivation because if you go too far classically with this one you have to think of electrons as spread out charges spinning so swiftly that their outsides would be at greater than light speed - an idea that put Wolfgang Pauli into quite a spin).

We can put most of the mechanisms discussed in statements 1. and 2. into symbols: suppose we wish to set up a system of currents of current density $\mathbf{J}$ in perfect conductors (so that there is no ohmic loss). Around the currents, there is a magnetic field; if we wish to increase the currents, we will cause a time variation in this magnetic field, whence an electric field $\mathbf{E}$ that pushes back on our currents. So in the dynamic period when our current changes, to keep the current increasing we must do work per unit volume on the currents at a rate of $\mathrm{d}_t w = -\mathbf{J} \cdot \mathbf{E}$.

However, we can rewrite our current system $\mathbf{J}$ with the help of Ampère's law:

$\mathrm{d}_t w = -\mathbf{J} \cdot \mathbf{E} = -(\nabla \wedge \mathbf{H}) \cdot \mathbf{E} + \epsilon_0 \mathbf{E} \cdot \partial_t \mathbf{E}$

then with the help of the standard identity $\nabla \cdot (\mathbf{E} \wedge \mathbf{H})=(\nabla \wedge \mathbf{E})\cdot\mathbf{H} - (\nabla \wedge \mathbf{H})\cdot\mathbf{E}$ we can write:

$\mathrm{d}_t w = -(\nabla \wedge \mathbf{E}) \cdot \mathbf{H} + \nabla \cdot (\mathbf{E} \wedge \mathbf{H})+\partial_t\left(\frac{1}{2}\epsilon_0 |\mathbf{E}|^2\right)$

and then with the help of Faraday's law:

$\mathrm{d}_t w = +\mu_0 \mathbf{H} \cdot \partial_t \mathbf{H} + \nabla \cdot (\mathbf{E} \wedge \mathbf{H})+\frac{1}{2}\epsilon_0 |\mathbf{E}|^2 = + \nabla \cdot (\mathbf{E} \wedge \mathbf{H})+ \partial_t\left(\frac{1}{2}\epsilon_0 |\mathbf{E}|^2+\frac{1}{2}\mu_0 |\mathbf{H}|^2\right)$

and lastly if we integrate this per volume expression over a volume $V$ that includes all of our system of currents:

$\mathrm{d}_t W = \mathrm{d}_t \int_V\left(\frac{1}{2}\epsilon_0 |\mathbf{E}|^2+\frac{1}{2}\mu_0 |\mathbf{H}|^2\right)\,\mathrm{d}\,V + \oint_{\partial V} (\mathbf{E} \wedge \mathbf{H}).\hat{\mathbf{n}} \,\mathrm{d}\,S$

(the volume integral becomes a surface integral by dint of the Gauss divergence theorem). For many fields, particularly quasi-static ones, as $V$ gets very big, the Poynting vector ($\mathbf{E} \wedge \mathbf{H}$ - which represents radiation), integrated over $\partial V$ is negligible, which leads us to the idea that the store of our work is the volume integral of $\frac{1}{2}\epsilon_0 |\mathbf{E}|^2+\frac{1}{2}\mu_0 |\mathbf{H}|^2$, so the magnetic field contributes to the stored work. It should be clear that this discussion is a general description of any dynamic electromagnetic situation and is wholly independent of the sign of $\mathrm{d}_t W$. So it applies equally whether we are working through the currents on the field or the field is working on us.

The above is very general: we can bring it into sharper focus with a specific example where it is almost wholly the magnetic field storing and doing work: say we have a sheet current circulating around in a solenoid shape so that there is a near-uniform magnetic field inside. For a solenoid of radius $r$, the flux through the solenoid is $\pi r^2 |\mathbf{B}|$ and the magnetic induction if the sheet current density is $\sigma$ amperes for each metre of solenoid is $|\mathbf{B}| = \mu_0 \sigma$. If we raise the current density, there is a back EMF (transient electric field) around the surface current which we must work against and the work done per unit length of the solenoid is:

$\mathrm{d}_t W = \sigma \pi r^2 \mathrm{d}_t |\mathbf{B}| = \frac{1}{2} \mu_0 \pi r^2 \mathrm{d}_t \sigma^2 = \pi r^2 \times \mathrm{d}_t \frac{|\mathbf{B}|^2}{2 \mu_0}$

This all assumes the rate of change is such that the wavelength is much, much larger than $r$. So now, the energy store is purely magnetic field: the electric field energy density $\frac{1}{2}\epsilon_0 |\mathbf{E}|^2$ is negligible for this example, as is the contribution from the Poynting vector (take the volume $V$ in the above argument to be a cylindrical surface just outside the solenoid: just outside the solenoid, the magnetic field vanishes and the Poynting vectors are radial at the ends of the cylinder so they don't contribute either. The above analysis works in reverse: if we let the currents run down, the electromagnetic field can work of the currents and thus the stored magnetic energy can be retrieved.

Answer 4

A magnet picks up pieces of iron because someone has configured that system to have the initial conditions such that this happens. The magnet was moved into a particular location near some pieces of ferro-magnetic metal, or vice versa.

The pieces of metal move because doing so reduces their potential energy in the magnetic field by a greater amount than it increases their gravitational potential.

The system releases energy. When the iron piece strikes the magnet and sticks to it, it produces a sound, and heat. It's not really a question or who or what does the work, but a situation in which a physical system has rearranged itself and changed energy from one form to another.

When the pieces are next to the magnet, they cause the field to be concentrated through them because they are highly permeable. As the magnet is covered with pieces, more and more of its field is concentrated through the pieces, and less and less of it is available for attracting new pieces. It is like a discharged battery.

Eventually you have to "recharge" the system by cleaning up the magnet so that you can keep using it. When you separate the pieces from the magnet, you have to put in energy.

Answer 5

From the formula of the Lorentz force it appears that the magnetic field does not do work by definition. The magnetic contribution is perpendicular to the displacement it causes. However the time derivative of the magnetic field is identical to the rotation of the electric field, so it implies the existence of an electric field that does work. So while formally B does not do work, a changing magnetic field is directly associated with work.

The root cause of this confusion is that E and B are not independent quantities, although from the Lorentz force alone they seem to be.

Answer 6

Below is opinion of Landau & Lifshitz.

Quote from "ELECTRODYNAMICS OF CONTINUOUS MEDIA" (Second Edition), page 128:

"When a conductor moves, the forces
$$\overrightarrow f=\overrightarrow j\times \overrightarrow H\frac{1}{c};\;\text{(1)}$$

($\overrightarrow j$ current density. $\overrightarrow H$ magnetic field)

do mechanical work on it.

At first sight it might appear that this contradicts the result that the Lorentz forces do no work on moving charges.

In reality, of course, there is no contradiction, since the work done by the Lorentz forces in a moving conductor includes not only the mechanical work but also the work done by the electromotive forces induced in the conductor during its motion.

These two quantities of work are equal and opposite.

In the expression (1) $\overrightarrow H$ is the true value of the magnetic field due both to external sources and to the currents themselves on which the force (1) acts.

The total force exerted by a magnetic field on a conductor carrying a current is given by the integral

$$\overrightarrow F=\int\overrightarrow j\times \overrightarrow H\frac{dV}{c};\;\text{(2)}$$

In calculating the total force from (2), however, we can take $\overrightarrow H$ to be simply the external field in which the conductor carrying a current is placed.

The field of the conductor itself cannot, by the law of conservation of momentum, contribute to the total force acting on the conductor."

End of the quote.

Answer 7

The work in picking up something is not done by the magnet, but by you!

Were a magnet and a piece of iron in free space (i.e. vacuum and no gravity), they'd simply start approaching one another, converting the potential energy of the magnetic field into kinetic energy. In gravity field, both would fall downwards, but e.g. if the magnet were above the iron, the magnet would fall slightly faster and the iron slightly slower due to the common attraction.

But now there's you (or e.g. a crane) holding the magnet in a fixed position (and the floor preventing the iron from falling via reactive forces). There are two scenarios:

• You put the magnet on the iron. In that case they simply stick together and when you lift the two, it is obviously you doing the work
• You hover the magnet over the iron. When close enough, the iron will rise to the magnet. But the magnet is also attracted to the iron and pulls downwards. But you won't let it get down, you strain your muscles slightly more in order to counter this. You are doing work when putting the magnetic above the iron, and it is (basically) exactly the amount required to add potential energy to the iron now attached to your magnet.

Answer 8

Consider a large wire oriented along X axis, carrying a current I in a static magnetic field along negative z axis. This will cause a force on the wire in upward direction and in rest frame and the work done is exactly the work done by the battery to maintain the current!! That negative work is done by the electric field in opposite direction of current in the frame of wire. This can be verified by performing Lorentz transform on the electromagnetic tensor in y direction. A magnetic field in z direction will have a component of electric field in x direction when Lorentz transformed in y direction. Below is the how electromagnetic tensor will look like in wire frame after Lorentz transform in y direction.
\begin{bmatrix}\gamma&0&-\gamma*v/c&0\\0&1&0&0\\-\gamma*v/c&0&\gamma&0\\0&0&0&1\end{bmatrix} \begin{bmatrix}0&0&0&0\\0&0&B&0\\0&-B&0&0\\0&0&0&0\end{bmatrix} \begin{bmatrix}\gamma&0&-\gamma*v/c&0\\0&1&0&0\\-\gamma*v/c&0&\gamma&0\\0&0&0&1\end{bmatrix}
Equals
\begin{bmatrix}0&\gamma*v*B/c&0&0\\-\gamma*v*B/c&0&\gamma*B&0\\0&-\gamma*B&\gamma&0\\0&0&0&0\end{bmatrix}

So in the wire frame, $$ E_x = -\gamma*v*B $$ and $$ B_z = \gamma*B $$ So, power delivered to unit length of wire by B is $$ P = \gamma*B*I*v $$ and, power delivered to unit length of wire by electric field is $$ P = -\gamma*v*B*I $$ As can be seen they cancel out and hence field has done zero work on the wire. The real work of lifting the wire is actually done by the battery.

Answer 9

The magnetic field causes the orientation without actually doing any work. The formula for work is not $F$, it is $W = \int F \cdot dr$, where the integral is along the path and $\cdot$ is the vector dot-product.

If you do your calculations correctly, you will see $W = 0$.

Answer 10

Well, here we go in maths.

W = SF.ds F = ma = m d2/dt2. F = qv x B = - vB x v = vmB x (v x dv/dt) F = vmB x 0.5x(v x ds2/d2t + dv/dt x dv/dv) F = When v = constant => dv/dt & other derivates are 0), thus SF.ds = 0