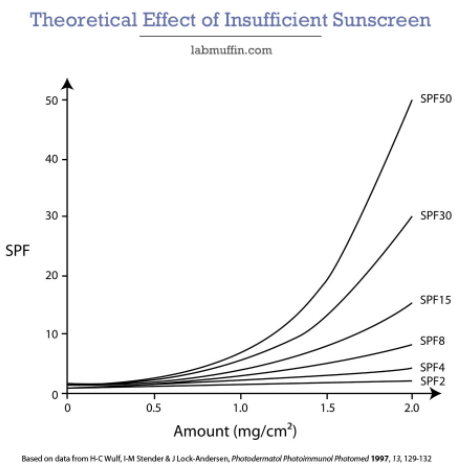
In the article How SPF Changes with How Much Sunscreen You Use (last updated Dec 29, 2017), the author says that it is disturbing that insufficient sunscreen (in theory) decreases protection exponentially:

The amount used in the sunscreen studies to determine SPF is $2$ milligrams of sunscreen per centimetre of skin.

On average, people apply only a quarter to a half of the recommended amount, and in most studies, it’s closer to $0.5$ mg/cm².

In physics, you’d expect protection to decrease exponentially with less sunscreen. This is based on a relationship called the Beer-Lambert law

it’s the middle of the graph that’s disturbing.

Applying half the recommended amount of sunscreen cannot give more than SPF $\mathbf{10}$, even with SPF $50$ sunscreen!

by $0.5$ mg/cm², we’re below SPF $3$ for all products.

Does this mean that applying more sunscreen theoretically gives increasing marginal utility?

Does the theory really contradict our intuition that the initial layer (of the recommended amount of sunscreen) is more beneficial—that is, makes a greater difference—than any subsequent layer?