Qs: How do I show that for a point object, for a ray at a large angle from the optical axis, spherical aberration (SA) produces a distorted focal length $f_{SA}$ that is shorter than the normal focal length $f$ (i.e. spherical aberration $\to$ more strongly bent ray)?
My attempt. Consider the diagram below.
From geometry, applying the cosine law to the triangle that links $l_1$ with $\phi$ $$l_1=\sqrt{(s_0+R)^2+R^2-2(s_0+R)Rcos(\phi))}$$ Similarly for $l_2$ $$l_2=\sqrt{R^2+\left(\frac{R-t(0)}{cos(\alpha)}\right)^2-2R\left(\frac{R-t(0)}{cos(\alpha)}cos(\alpha- \phi)\right)}$$ For $l_3$, used pythagoras $$l_3=\sqrt{(s_i-t(0))^2+(R-t(0))^2tan^2(\alpha)}$$ I want to find an expression that relates $l_1,l_2,l_3,s_i,s_0,R, n_1,n_2$ using $$OPL=n_1l_1+n_2l_2+n_1l_3$$, and (Fermat) $$\frac{dOPL}{d\phi}=0$$ so that I can show that $s_i$ is smaller than the normal focal length. However, I'm struggling to find the relation bewteen $\phi$ & $\alpha$.
Is there a simpler way to do this?
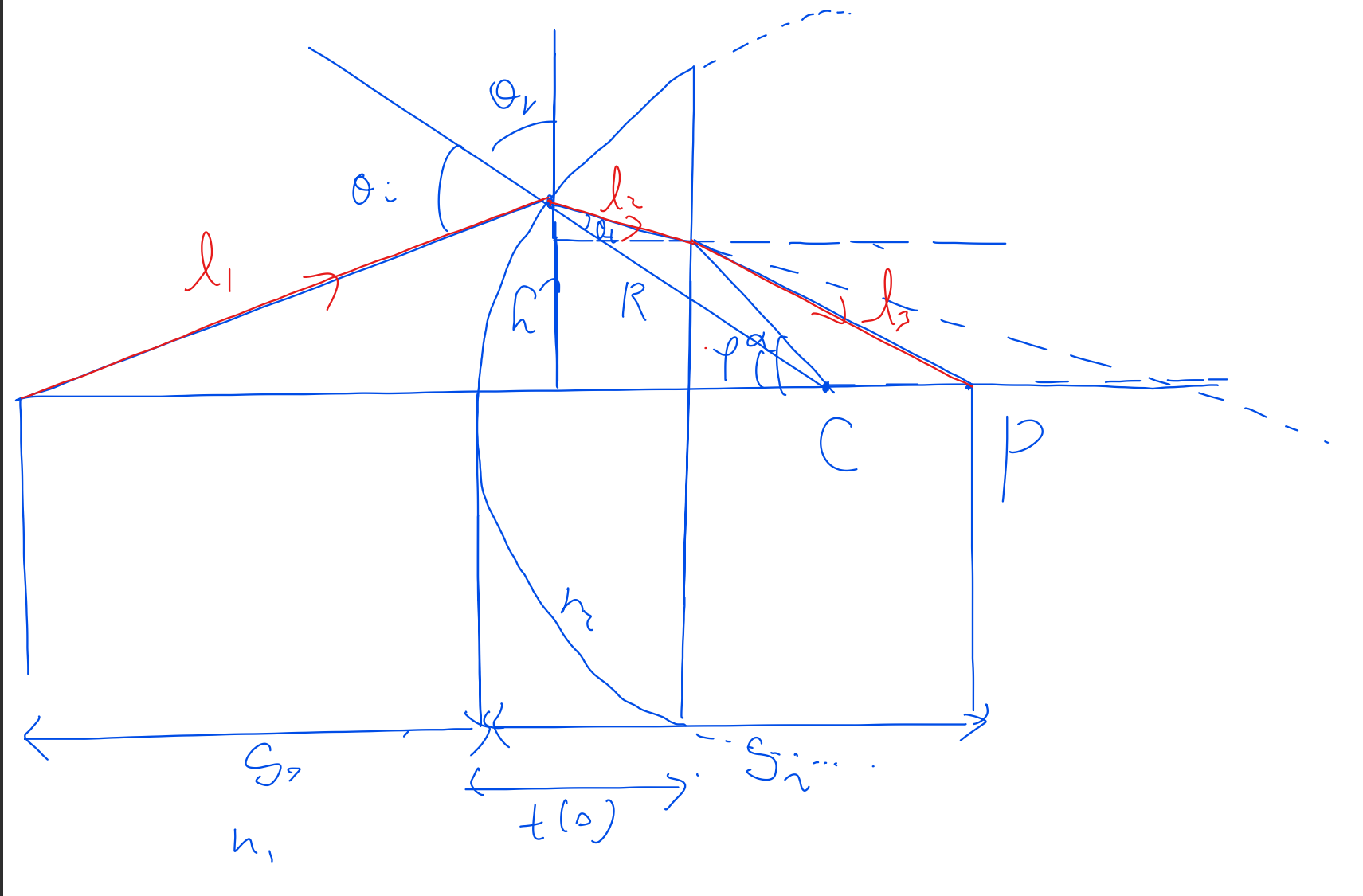 (Spherical lens with radius R, centre C and thickness $t(0)$ . The ray hits the optical axis at the point P. $s_o$ and $s_i$ are measured from the vertex)
(Spherical lens with radius R, centre C and thickness $t(0)$ . The ray hits the optical axis at the point P. $s_o$ and $s_i$ are measured from the vertex)
