I've seen many science popularisation documentaries and read few books (obviously not being scientist myself). I am able to process and understand basic ideas behind most of these. However for general relativity there is this one illustration, which is being used over and over (image from Wikipedia):
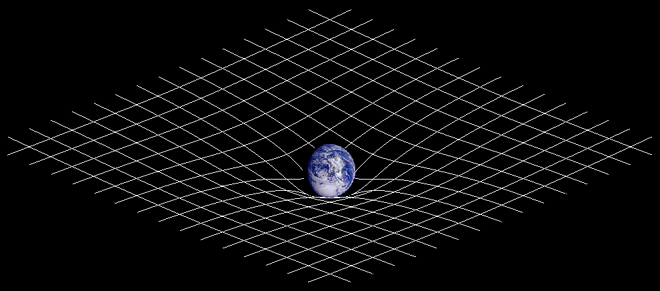
I always thought that general relativity gives another way how you can describe gravity. However for this illustration to work, there needs to be another force, pulling the object down (referring to a direction in the attached image). If I put two non-moving objects in the image, what force will pull them together?
So where is my understanding incorrect? Or is general relativity not about explaining gravity and just describes how heavy objects bends spacetime (in that case the analogy is being used not correctly in my opinion)?
UPDATE Thank you for the answers and comments. Namely the XKCD comics is a spot on. I understand that the analogy with bent sheet of fabric pretty bad, but it seems that it can be fixed if you don't bent the fabric, but just distort the drawn grid.
Would you be so kind and answer the second part of the question as well - whether general relativity is explaining gravitational force. To me it seems that it is not (bending of spacetime simply can not affect two non-moving objects). However most of the time it is being presented that it does.


