0
$\begingroup$

Why do objects curve the space-time down; why not up?

Could the space-time model created by Einstein affected by his knowledge that things (in earth) fall down ?

Note: please, be kind, I am not a physicist .

Improve this question
$\endgroup$
2

2 Answers 2

Reset to default
4
$\begingroup$

It sounds like you have been looking at the famous rubber sheet visualization. 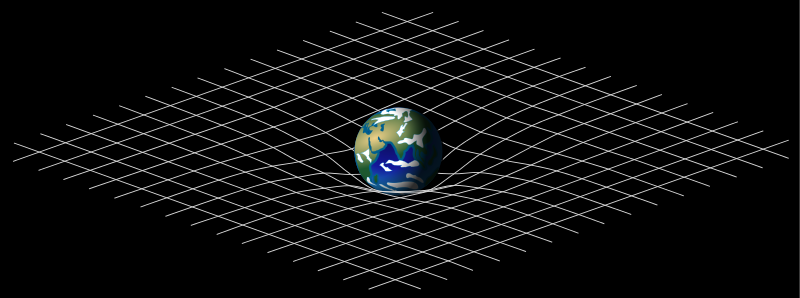enter image description here The rubber sheet visualization is generally frowned upon by people working with general relativity, because as you've realized, it isn't actually very helpful. After all, what makes masses on a rubber sheet curve it, is gravitation, so how can this "explanation" of gravitation be anything but a fake explanation?

The visualization is also a bit misleading in a technical sense. In the mathematical description, the curvature has one component for each two-dimensional plane through a point in spacetime [in technical language: it's a two-form]. The curvature illustrated with the rubber sheet is in a space-space plane. But it's very important to general relativity that we use a spacetime description, and take into account also the space-time components of the curvature.

Furthermore, the motion of objects on a curved rubber sheet does not correspond to the motion of objects according to general relativity. The motion on the rubber sheet is determined by the slope of the sheet. In general relativity, it's determined by a more complicated geometric object that is related to the curvature (in a precise technical way).

My opinion, which might be harsh, of the rubber sheet visualization is that I don't think there is anything in it that helps visualize general relativity, because it doesn't seem that there is anything in it that can be put into correspondence with the technical formulation of general relativity.

Unfortunately, it's really difficult to do honest, good visualizations of general relativity because it's fundamentally a theory of spacetime -- drawing 3-dimensional objects on paper is hard as is, but how do you draw the time dimension? Even so, the mathematical object that completely describes the curvature has 256 components in every point [only 20 independent ones, but still].

Now, since general relativity is a theory of geometry, it's possible, and I think very useful, to make pictures. Geometry is about distances, shapes, that's things drawing is good for! One very famous textbook has many figures that, unlike the rubber sheet, correctly and honestly illustrate for example curvature. But what you always have to keep in mind, is that because of the limitations, even such figures only illustrate the concepts in simplified settings such as 2-dimensional surfaces, as opposed to 4-dimensional spacetime. To treat the latter, you need the formal mathematical description.

There is in general a trade-off between simplicity and precision. Unfortunately in the case of general relativity, the exchange rate is very steep.

Improve this answer
$\endgroup$
0
0
$\begingroup$

There's no universal "down" or "up" direction. The spacetime "curving" represents a distorting of the relationship between the spatial axes and the time axis - it occurs in that 4-dimensional system.

This video contains a visual description that may clarify things: https://www.youtube.com/watch?v=wrwgIjBUYVc

Improve this answer
$\endgroup$
1
  • $\begingroup$ As it’s currently written, your answer is unclear. Please edit to add additional details that will help others understand how this addresses the question asked. You can find more information on how to write good answers in the help center. $\endgroup$
    – Community Bot
    Commented Jul 23, 2022 at 22:46

Not the answer you're looking for? Browse other questions tagged or ask your own question.