As pointed out in the comments, the method is correct aside from a careless error of using the diameter, when radius was clearly intended to be used by the argument.
I calculate the "area" of the earth, as seen from the sun, and then divide that by the surface area of a sphere of radius 1 AU, to get the portion of the sun's rays we absorb, and then I multiply that by the solar luminosity.
For a sanity check, I will propose another way to get the same thing. The sun is roughly a black body, but you didn't need this info since you started with the Luminosity. You can recalculate that value approximately as follows.
$$ L_{\circ} = \sigma T^4 A_s $$
Take out the area from this expression. Now you have $W/m^2$, but at the surface of the sun. Convert this to the average solar insolation at Earth's location, you can simply multiply by the ratio of areas of those two spheres. That is, multiply the sun's $W/m^2$ value by the area of the sun's surface divided by the area of the 1 AU sphere to get the $W/m^2$ at 1 AU. The ratio of areas of two shapes will be the ratios of linear dimensions, squared. It doesn't matter which.
Returning to your objective quantity, that is simply the area of Earth presented to the sun times the $W/m^2$ at 1 AU.
$$ P = \frac{ L_{\circ} }{ A_{AU} } A_{Earth} = \sigma T^4 \left( \frac{ 1 AU }{ R_{s} } \right)^2 A_{Earth} $$
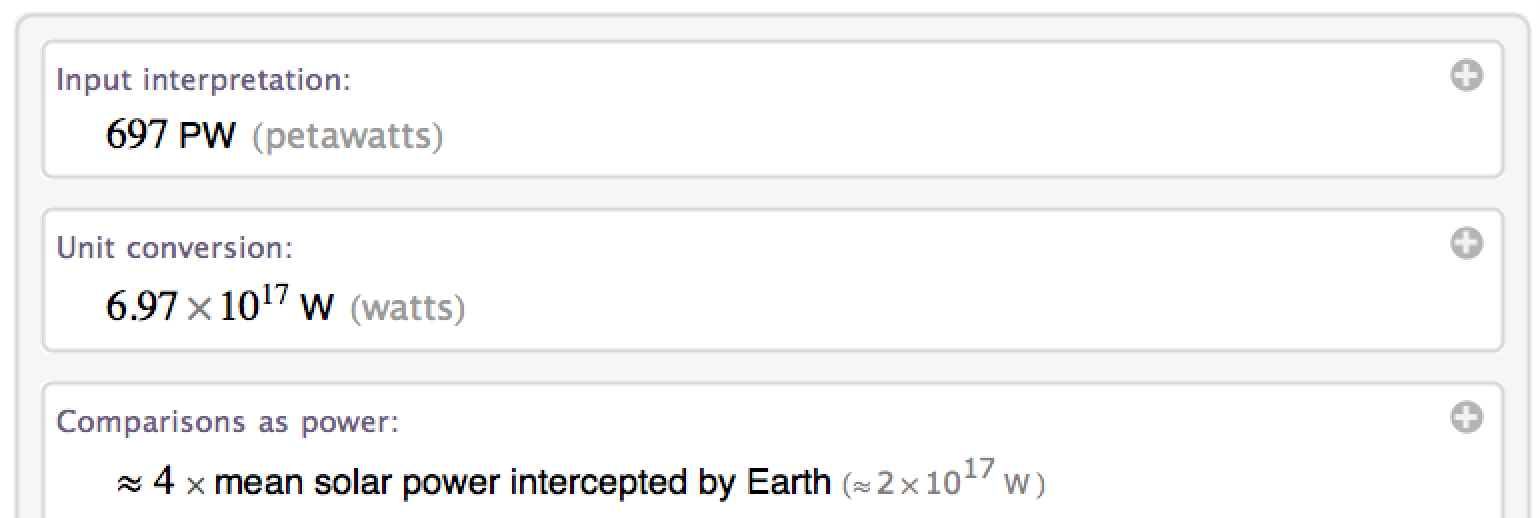
I can't say this would have caught your problem, but it's a useful sanity check in general. It avoids comparing $4 \pi r^2$s against $\pi r^2$s, which gets a little bit dizzying. Here is the calculation on Wolram Alpha. It produces $1.74 \times 10^{17} W$, which might be different only within the significant figures. It's probably close enough to validate that this is another correct calculation.
![\text{UnitConvert}\left[\frac{\pi \text{Quantity}[\text{AstronomicalData}[\text{Earth},\text{Diameter}],\text{m}]^2}{4 \pi \text{Quantity}[1,\text{astronomocal units}]^2} \text{L}_{\odot },\text{W}\right]](https://i.sstatic.net/516fe.png)