What unusual effects will we observe on the non-rotating Dyson sphere with Earth gravity around the Sun?

Question

First, let's place our sphere

Let's assume there is a two-layer non-rotating Dyson sphere around the Sun (ignore the scale):

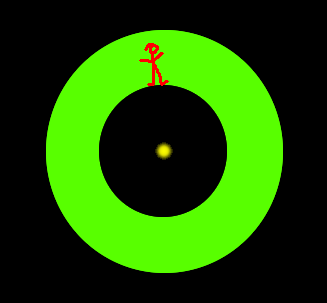
Green is empty air. How far should the inner border of this sphere be from the Sun surface for the man standing on it like shown in the picture to experience normal Earth gravity?

I googled around and found this:

$$g=\frac{GM}{r^2}$$

$g$ – gravitational acceleration on the earth's surface
$G$ – gravitational constant
$M$ – mass of the object (in our case the Sun)
$r$ – distance from the center of the mass of the object

So, for $g=9.8\ \mathrm{m/s^2}$, we have

$$\begin{align} r&=\sqrt{\frac{GM}{g}}\\ &=\sqrt{\frac{6.67\times{10}^{-11}\ \mathrm{m^3\ kg^{-1}\ s^{-2}}\times1.99\times{10}^{30}\ \mathrm{kg}}{9.8\ \mathrm{m\ s^{-2}}}}\\ &=3.68\times{10}^9\ \mathrm m \end{align}$$

So, around 3.68 million km, if we subtract Sun radius we get a little less than 3 million kilometers from the solar surface.

Now the main question

If my calculations are right, our inner shell is very close to the Sun, so it should experience a ton of various effects, like Sun's magnetic field, solar wind, etc. Let's ignore for the moment the actual way our non-rotating sphere manages to stay static and not fall towards the Sun. What effects will we see on the outer side of the inner shell (where the red stick man stands), e.g. Sun's magnetic field effects or maybe some non-Newtonian effects?

Why?

I'm researching this for a sci-fi book that's in the works and I want the sci part to be as accurate as possible, there will be some fictional elements of course—to serve the plot—but I'd like to limit contradictions to existing science as much as possible.

Answer 1

A Dyson sphere orbiting the Sun at 5 solar radii will have problems that will be faced such as:

1. Heat. The Sun's surface temperature is around 5777K. At 5 solar radii away, the solar irradiance or the intensity of light, W/m^2, would be around 2.1 E6 W/m^2. In comparison at the same distance as Earth is from the Sun, you would only get 1366 W/m^2. That amount of energy striking the inner Dyson sphere would melt any metal you put very quickly. Heat Dissipation is vital in keeping your Dyson sphere a Dyson sphere.

2. Solar Wind. The Solar wind is comprised of charged particles that are being expelled. They move at around 2.8 E5 m/s. So they are going to do a lot of damage to whatever equipment you are having on the sphere and the sphere itself. A solution to this is probably a magnetic shield which diverts the solar wind away. This brings me to the next problem.

3. Coronal Mass Ejections (CME). CMEs occur when built up when magnetic fields reconnect and a tremendous amount of energy is released. The solar wind to a CME is like a continuous water faucet to a barrel of water thrown all at once at you. A strong CME will do damage to city electrical grids, power lines and cause power surges on Earth if directed straight at us and Earth is 1.5 E11 m away. The sphere is 3.7 E9 m away which surrounds the entire star. If a super strong CME occurs like the Carrington Event in 1859, your equipment on the sphere and your Sphere is screwed.

TL;DR: (1) Too hot, (2) Too many charged particles, (3) Explosions.

---

That's the major effects of having a Dyson sphere. However in the future there's also going to be one more stuff. Assuming your sphere survives for 5 billion years.

4. Red Giant. The Sun will expand as it ages. It will engulf Mercury and Venus and probably Earth, Mars maybe before it dies. The Sphere is in the orbit of Mercury so when the Sun expands, goodbye Dyson Sphere.

There will also be a lot more other implications that I did not think on the spot. I may add some if I recall any more. However I do have some improvements that can be made.

If you want to have the effect of gravity on the sphere, you can spin the sphere, letting centrifugal "force" be the effect of gravity so you don't have to have the Dyson sphere so close to the sun but this brings a lot other bad stuff like people losing consciousness due to the Coriolis effect but that's a story for another day. Another solution is just don't put people on the Dyson sphere. However being a type 2 Civilization, we should have solutions to most problems that will be faced by then.

Answer 2

I will ignore the material-strength problems with constructing such a thing: let's just assume you have magic super-strong stuff.

Instead I'll focus just on temperature. First of all you want the gravity on the sphere to be $g$, or in other words:

$$g = \frac{GM}{r^2}$$

so

$$ \begin{align} r &= \sqrt{\frac{GM}{g}}\\ &\approx 3.68\times 10^9\,\mathrm{m} \end{align}$$

We also know the semi-major axis of Earth's orbit, $R\approx 1.5\times 10^{11}\,\mathrm{m}$, and the flux from the Sun at that distance (the solar constant, to climate scientists), $s_R \approx 1.4\times 10^3\,\mathrm{Wm^{-2}}$.

Conservation of energy gives the energy flux at any radius $r$:

$$s(r) = s_R\left(\frac{R}{r}\right)^2$$

So in particular this tells us the flux crossing the radius of the proposed sphere (which is $2.5\times 10^6\,\mathrm{Wm^{-2}}$).

Let's assume the sphere is a perfect black body: we know the flux it radiates is $\sigma T^4$, where $\sigma \approx 5.67\times 10^{-8}\,\mathrm{kg\,s^{-3}K^{-4}}$.

Putting this together we can compute its surface temperature:

$$T(r) = \left(\frac{s_R\left(\frac{R}{r}\right)^2}{\sigma}\right)^{\frac{1}{4}}$$

And given $r \approx 3.68\times 10^9\,\mathrm{m}$ from above we get

$$T \approx 2.51\times 10^3\,\mathrm{K}$$

This is well above the melting point of steel, but below that of tungsten. it would be white hot and the light from it would be somewhat bluer than a normal incandescent bulb (which run at about $2,400\,\mathrm{K}$ but not as blue as a tungsten-halogen bulb can be (and obviously also not as blue as the Sun).

It would, of course, radiate as much power as the Sun does.

---

So you are not going to be living on such a thing: Dyson spheres, in the sense you mean them, are not physically viable. If you want something which is physically plausible you want a ringworld: that requires magic super-strong stuff and, famously, requires dynamic stabilisation (Dyson spheres do as well), but are otherwise reasonably sane (for rather loose meaning of 'reasonable').