In carnot engine we know the steps like first isothermal, than adiabatic, then reverse isothermal and adiabatic. And we know that efficiency increase if the temperature of hot box(heat supllier) is high and cold box(heat sink) low.But how does this high and low temperature creates more efficiency intuitively?
$\begingroup$
$\endgroup$
2
New contributor
kashpia tahamin is a new contributor to this site. Take care in asking for clarification, commenting, and answering.
Check out our Code of Conduct.
-
$\begingroup$ The question in your title is different from the question in the body text. Can you clarify what you're asking by editing one or the other? $\endgroup$– ChemomechanicsCommented yesterday
-
$\begingroup$ To address the title question, unlimited adiabatic expansion can remove all energy from the working fluid of the Carnot cycle. However, recompression is needed to reset the system to draw more energy from the hot reservoir, and the work required for recompression is governed by the temperature of the cold reservoir. This answer may also be useful. Please also consider clarifying what level of answer you're looking for (e.g., what concepts you're already familiar with). $\endgroup$– ChemomechanicsCommented yesterday
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
As already pointed out, the question posed in the title of your post differs from the question in the body of your post. So I'll answer them separately.
To answer the question in the title, the lowest energy can theoretically approach zero.
The end of the adiabatic expansion corresponds to the temperature of the low temperature reservoir. That temperature can theoretically approach absolute zero where all molecular motion theoretically stops. For an ideal gas, where the internal energy is considered purely kinetic, that would mean the internal energy approaches zero. I said approach zero internal energy because absolute zero temperature can only be approached, never attained.
Insofar as intuitively explaining how the larger difference between the high and low temperatures creates more efficiency, one way is to think of the difference in temperature as a measure of thermal potential energy available to perform work. It is roughly analogous to the difference in elevation as a measure of the gravitational potential energy available to perform work. The greater the difference, the greater the energy available for doing work, all else being equal. This applies to all reversible heat engine cycles, not just the Carnot heat engine cycle.
The Carnot efficiency is the maximum possible efficiency because the temperature range is between two fixed temperature thermal reservoirs. The fact that all of the heat absorbed is from a single high temperature reservoir and all the heat rejected is to a single cold temperature reservoir results in the greatest possible efficiency for a reversible heat engine cycle.
On the other hand, in the case of a reversible heat engine cycle in which heat is absorbed and/or rejected over a range of temperatures, instead of two fixed temperatures like the Carnot cycle, the amount of work done over the cycle will be less for the same maximum and minimum temperatures resulting in lower efficiency.
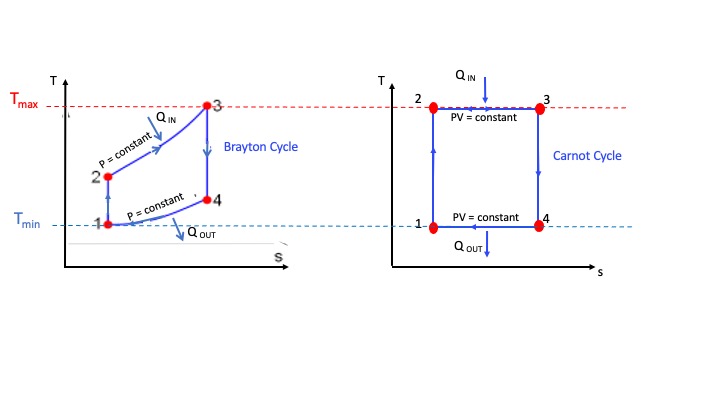
This can be seen in comparing the temperature-entropy graphs of the cycles. As an example, the figures below compare a Brayton heat engine cycle and Carnot cycle operating between the same maximum and minimum temperatures. The area enclosed in the cycle represents the net work done.
Hope this helps.
