Why adiabatic expansion happens in Carnot cycle?

Question

$A \rightarrow B$: Isothermal expansion happens. Since the internal energy purely depends on the temperature ($E_{int} = knRT$, $k = \frac{3}{2},\frac{5}{2},3$), then $\Delta E_{int}=0$ in an isothermal expansion. Thus all of the heat absorbed will be used in expanding the volume, and the temperature remains constant throughout the process. It's assumed that isothermal process happened otherwise it's not reversible, but the engine is assumed to be ideal.

in $B \rightarrow C$, the working substance is insulated, and thus $Q = 0$, so $\Delta E_{int} = W$ where $W$ is the work done by the environment to the system. By definition, $Q=0$ means it's adiabatic process. But why does adiabatic expansion happen and not adiabatic compression or not just doing nothing / staying still .

Answer 1

in $B \rightarrow C$, the working substance is isolated, and thus $Q = > 0$, so $\Delta E_{int} = W$ where $W$ is the work done by the environment to the system, not work done by the environment on the system.

In $B\rightarrow C$ the working substance is not isolated. It does work on the environment, not the other way around, expanding adiabatically and reversibly, with $Q=0$.

But why does adiabatic expansion happen and not adiabatic compression or not just doing nothing / staying still .

Because after the isothermal expansion occurs, the external pressure continues to be gradually reduced so that the gas can continue to expand adiabatically until the temperature of the gas drops to the temperature of the low temperature reservoir. Then the gas undergoes a reversible isothermal compression followed by a reversible adiabatic compression to complete the cycle.

but why ' the external pressure continues to be gradually reduced'? I have understood the rest.

It might help you if we look first at the reversible isothermal expansion that precedes the reversible adiabatic expansion since it, too, involves reducing the external pressure. .

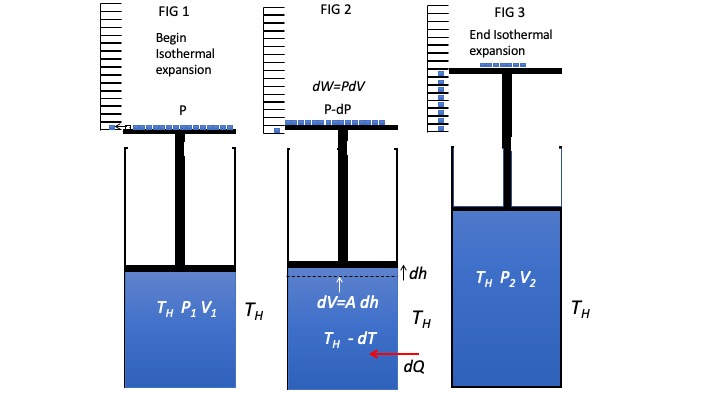
Consider the following thought experiment. Let's say you have an ideal gas in a vertically oriented frictionless cylinder and massless piston. See Fig 1 below which shows the beginning of the reversible isothermal expansion. On top of a platform connected to the piston is a weight which, in addition to the external air pressure, provides the external pressure on the system.

In order to carry out this expansion extremely slowly (reversibly) we can imagine the weight as a pile of sand (only a few grains shown). We begin the process by removing one grain of sand, as shown in Fig 1. That results in an infinitely small expansion of the gas, $dV=Adh$ as shown in Fig 2. The expansion causes an infinitely small decrease in the gas temperature $dT$ and an infinitely small transfer of heat $dQ$ into the gas to bring its temperature and pressure back into equilibrium with surroundings, awaiting the next removal of a grain of sand.

We continue the process one grain of sand at a time so that the gas is in thermal and mechanical equilibrium with the surroundings at all times, until we reach the final desired volume, as shown in Fig 3.

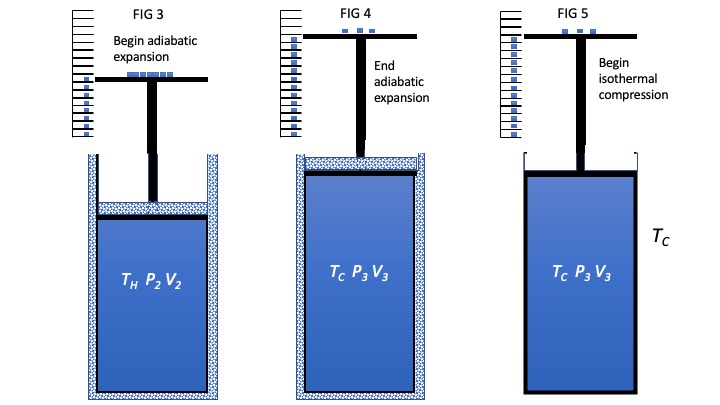
In Fig 4 we surround the piston and cylinder with thermal insulation so that no heat transfer can occurs. This is the beginning of the adiabatic expansion. We remove the next grain of sand, reducing the external pressure by $dP$. The infinitesimal expansion $dV$ results in an infinitesimal decrease in temperature. We continue the process of reducing the pressure until the final desired temperature, $T_C$ is reached in Fig 5. We then remove the thermal insulation in Fig 6 in preparation for the isothermal compression that follows.

Hope this helps.

Answer 2

Thanks a lot to BobD for answering this -- it took me a while to understand the answer though.

Quoting BobD: "It might help you if we look first at the reversible isothermal expansion that precedes the reversible adiabatic expansion since it, too, involves reducing the external pressure."

I couldn't first understand why the external pressure needed to be reduced. It seemed to me (due to lack of clarity) that supplying heat in step 1 was enough to cause expansion and there was no need to reduce the external pressure!

The point I missed to see was that, both the heat source/reservoir and the fluid in step 1 are supposed to be at temperature T_H. How then do we expect heat to flow from the source to the fluid? The only way is by controlling the external pressure. As BobD describes, as the external pressure is decreased by a tiny amount, the fluid expands a tiny bit and suffers a tiny drop in temperature and allows heat flow from the source! Supplying all the heat at the max temperature T_H and rejecting some of the heat at the min temperature is what makes the Carnot engine most efficient!