That is an picture of the best we can argue about what happens in spacetime, avoiding non-physical notions as "instantaneous changes" when a couple of measurments are performed on an entangled state of two-particles (and nothing further happens in spacetime for that system).
In the picture, $M_1$ and $M_2$ are the causally separated regions in spacetime where measurements take place.
The state of the two particles is:
(a) the entangled one $$\psi_1\otimes \psi_2 + \psi_2 \otimes \psi_1.$$
everywhere in the region causally separated with $M_1\cup M_2$ and in the causal past of both them.
(b) the "collapsed" one $$\psi_1\otimes \psi_2$$ everywhere in the causal region which can receive physical information from the outcomes. (The other possibility is $\psi_2\otimes \psi_1$ everywhere therein.)
If we assume that observables localised in spatially separated regions commute, this physical setup cannot give rise to causal problems also adding (thus changing the figure) further measurments on the system with experiments localised in bounded regions of the spacetime.
All that was discussed, into a very general way, by K.Hellwig and K. Kraus in "Formal Description of Measurements in Local Quantum Field Theory" Phys Rev D vol 1 n.2 1970.
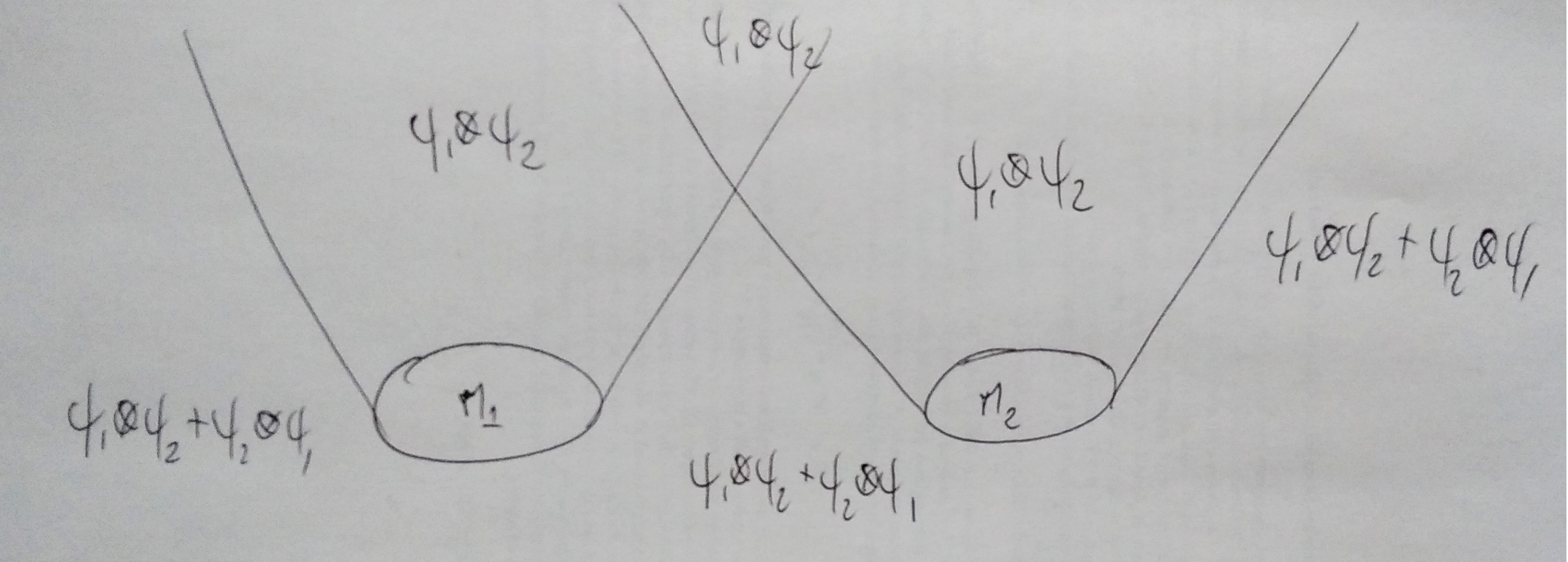
The puzzling issue, concerning entangled states and causally separated regions, is how the state can appear to be $$\psi_1\otimes \psi_2$$ close to the both the regions $M_1$ and $M_2$ (in their causal future). That is because no information can be transmitted between these two regions and the outcomes cannot "synchronize" with each other.
This may seem rather strange but, in principle, it does not give rise to a physical or logical contradiction. Special Relativity and Quantum Theory coexist peacefully up to now. First of all because in quantum mechanics the outcomes of measurments are intrinsically stochastic and there is no way to force a special outcome and construct a Morse telegraph between $M_1$ and $M_2$. But there are also more sophisticated arguments.
If we want to define some kind of (classical-like) mechanism that produces this sort of correlation, we know, after Bell's analysis, that the mechanism cannot be both realistic (the outcomes are already fixed before the measurements) and local (superluminal transmission of physical information is not admitted).
I emphasize that, in my view, there is no need for such a mechanism (especially of classical type). Nothing really requires it. The search for a deeper mechanism is mainly due to our philosophical prejudices. Pursuing some of them, in the past, has led us to new fundamental physics, but nothing ensures that this is the case with these strange properties of entanglement in QM.
ADDENDUM. The weirdness above does not depend on the physical solidity/inconsistency of processes such as "collapse" of the state.
We can consider the collapse simply as an effective and convenient description as I now try to explain. In this view, the quantum system interacts with the measuring instrument and the environment, but the evolution of the overall system is always unitary and no "collapse" occurs at any time.
In the measurement process the state of the system entangles with the state of the instrument and the environment. Before the interaction the states were factorized (t least the one of the measured system with the one of the rest of the overall system. The final state is instead given by a certain coherent superposition of products od instrument states and states of the system. The instrument states point out the corresponding outcomes of the measurement process.
If we intend to formally deal only with the system, we can ignore the instrument and the environment after the interaction with the instrument. This is equivalent to dealing with an effective state of the system produced by the partial trace operation.
(In the description above, a "decoherence mechanism" plays a central role, but I will not insist on this point here).
The partial trace operation produces an effective mixed state of the system as a superposition of the various possible outcomes of the instrument.
In this whole picture, I am assuming that quantum mechanics deals with ensembles and not with the individual systems that belong to the ensembles.
The specific system we have at hand (here a single pair of entangled particles) simultaneously shares three quantum states:
(1) the overall pure state of the overall system,
(2) the mixture obtained by partially tracing the previous state,
(3) a specific pure state in this mixture.
By adopting one of these three states, QM produces different statistical predictions, but these are compatible because they refer to three different ensembles to which the system belongs.
By adopting the mixture description, we can move on to focus on the subensemble (of the initial ensemble) associated with the specific branch of the effective mixture to which our specific system belongs.
This gives rise to the apparent phenomenon of "collapse".
However, even in this approach, where collapse does not play a magical role and is not the reason for the strangeness, something remains weird.
It is the fact that, even though the measurement process takes place in two causally separated parts, the outcomes in these regions are strongly correlated. The responsibility for this lies with the entangled state.

