Comparison of processes for a thermally insulated chamber containing hot gas and some metal [closed]

Question

I have the following problem from a textbook on thermodynamics:

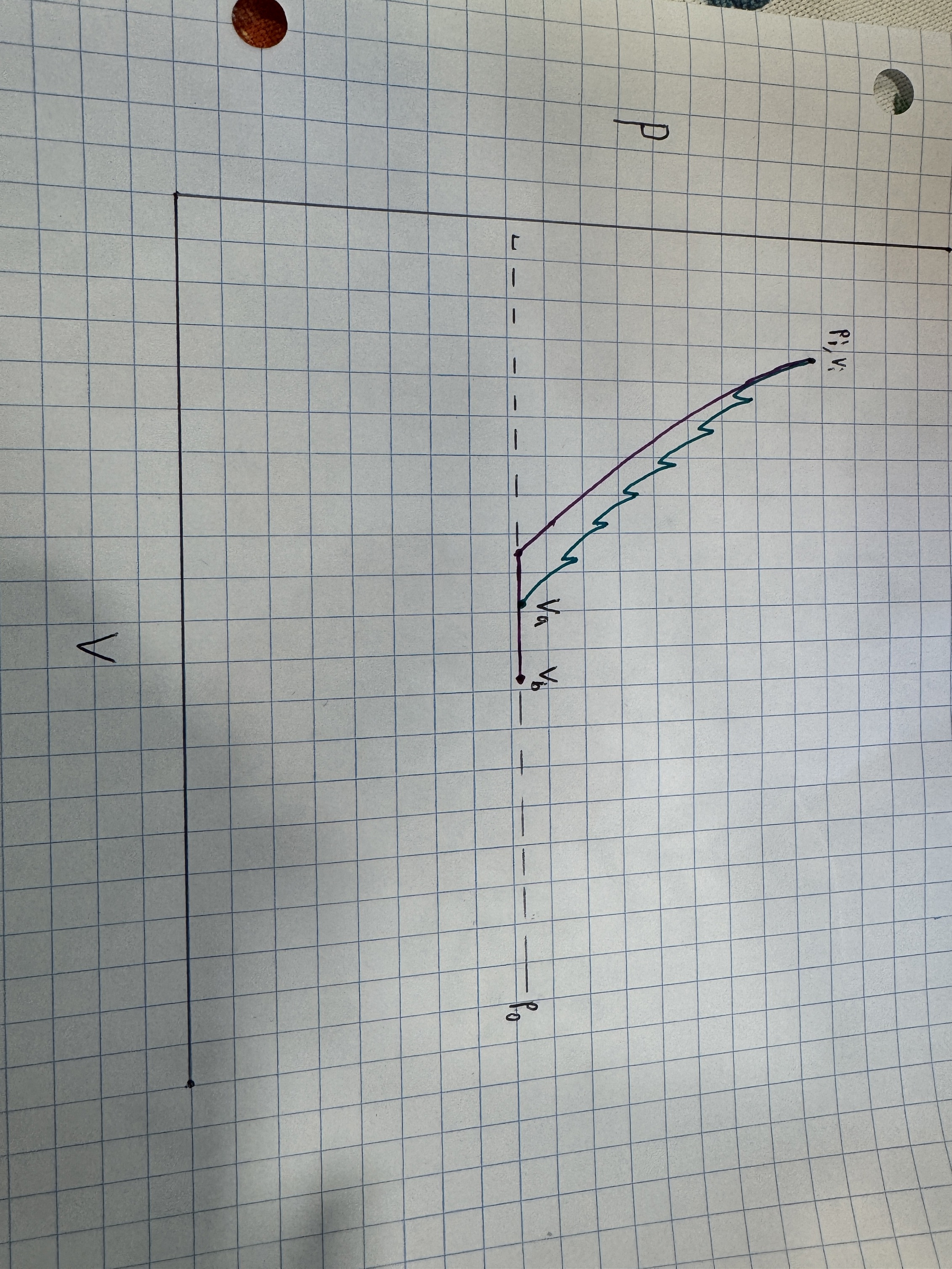
A thermally insulated chamber contains some hot gas and a lump of metal. Initially the gas and the lump are at the same temperature $T_i$. The volume of the chamber can be changed by moving a frictionless piston. Assuming the heat capacities of the gas and the metal lump are comparable, sketch on one diagram the pressure-volume relation for the system ...

• (a) ... if the pressure is reduced to atmospheric pressure $p_0$ slowly enough for the temperature of the metal lump to be equal to that of the gas at all stages.
• (b) ... if the pressure is reduced to $p_0$ fast enough for the metal lump not to cool at first (but the process is still quasistatic for the gas) after which the piston is further moved so as to maintain the pressure at $p_0$ until the metal lump and the gas attain the same temperature.

Use the first law to explain whether or not the final volume will be the same in these two processes. Explain which process finishes at the lower temperature. [Hint: consider the work done and use the fact that internal energy is a function of state for any given system.]

The problem appears before the chapter on entropy, so I'm trying to answer it using ideas involving energy and state variables. Unfortunately, I haven't been able to reach a conclusive solution to any part of this problem despite trying a few different approaches. Here is one of my attempts.

Assume the gas is ideal and choose it as the system to be treated. Let $p_i$ and $V_i$ be the initial pressure and volume respectively. In order to sketch the p-V diagrams correctly relative to one another, we'd have to compare the final states of the system after each process. Since the final pressure is the same for both and the system has just two degrees of freedom, we'd only have to compare one other state variable. I decided to focus on the temperature as it relates simply to the internal energy.

Process (b) appears to be an adiabatic expansion (since no heat is exchanged with the system at first) followed by an isobaric expansion by heating from the metal. Let $V_n$ and $T_n$ be the intermediate volume and temperature immediately following the initial expansion. Then,

$$ p_iV_i^\gamma = p_0 V_n^\gamma \implies T_i^\gamma p_i^{1-\gamma} = T_n^\gamma p_0^{1-\gamma}, $$

so that $T_n = T_i \left(\frac{p_i}{p_0}\right)^\frac{1-\gamma}{\gamma}$. In order for the gas and the metal to reach thermal equilibrium at some final temperature $T_b$, the heat output from the metal would equal the heat input to the gas:

$$ \begin{aligned} Q = C_p\Delta T_{metal} &= -C_p\Delta T_{gas}\\ T_b - T_i &= -(T_b - T_n)\\ T_b &= \frac{T_i + T_n}{2}, \end{aligned} $$

so that $T_b = \frac{T_i}{2}\left(1 + \left(\frac{p_i}{p_0}\right)^\frac{1-\gamma}{\gamma}\right)$ is the final temperature of the system.

Now consider process (a). Let $V_a$ and $T_a$ be the final volume and temperature of the system. The equation of state allows us to find $V_a$ in terms of the other variables:

$$ p_i V_i = Nk_BT_i,\\ p_0 V_a = Nk_BT_a, $$

so $V_a = \frac{p_i T_a}{p_0 T_i} V_i$. I believe that the process is reversible, so the work done by the external atmosphere is given by:

$$W = -\int^{V_a}_{V_i}p_0 \mathrm{d}V = -p_0(V_a - V_i).$$

Then, assuming that the volume of the metal remains unchanged by the difference in pressure, it follows that the heat provided to the system by the metal is $Q = -C_v(T_a - T_i)$. Furthermore, the first law tells us that the difference in internal energy $U$ is given by

$$\Delta U = W + Q = C_v \Delta T ~ \text{(for an ideal gas).}$$

Upon substitution of my expressions for $Q$, $W$, and $V_a$, a bit of calculation brought me to

$$(C_v + C_p)T_a = \left(\frac{p_0}{p_i}(C_p - C_v) - 2C_v\right)T_i.$$

At this point, it seems unlikely that I would be able to make any useful comparison between my expressions for $T_a$ and $T_b$. Taking a few steps back, I tried to calculate the work a different way:

$$W = -\int^{V_a}_{V_i}p_0 \mathrm{d}V = -p_0 \int^{T_a}_{T_i} \left. \frac{\partial V}{\partial T} \right|_{p} \mathrm{d}T = (C_v - C_p)\Delta T,$$

but this just leads to standard known results for p-V systems when applied to $\Delta U = W + Q$, and I'm no closer to reaching a solution.

I know it is better to ask about specific concepts here but, as it stands, I'm having a lot of trouble with thermodynamics problems as a whole. My lack of intuition for the subject leads me to believe that I'm not understanding several of the foundational concepts, so I would really appreciate any pointers and clarifications for any errors in my work or reasoning here.

Answer 1

Process (a) will finish with a lower temperature.

It is certainly possible to compute the final volumes of both processes, but we can also analyze this situation more conceptually.

In process (a), the piston expands slowly to allow the lump to give off heat and stay at the same temperature as the surrounding gas. We can break this process into many discrete 'steps,' where the piston (quasistatically) expands, then the lump and gas temperatures equalize, and then the piston expands again, and so forth. If we just consider the gas, this is equivalent to a repeating series of adiabatic expansions and isochoric heatings.

On the other hand, in process (b), the piston expands rapidly, and then the lump gives off heat while the gas maintains its pressure, so the process is like an adiabatic expansion followed by an isobaric one.

As the problem's hint suggests, we will want to consider the work done by the gas to solve the problem. Since $W = \int P dV$, we can visually see the work done on a P-V diagram (attached at the end of this post). Because process (b) expands directly, the pressure at each volume will be lower. Now, let the final volumes of processes (a) and (b) be $V_a, V_b$.

If $V_b \leq V_a$, the total work done by the gas will be less in process (b), since the volume over which the gas expands is lower (or equal) and the pressure during the expansion is lower. Thus, that the gas has done less work and, therefore, lost less energy, so its final energy should be higher. But if it has a higher energy, it must also have a higher volume, since the energy of a gas is proportional to $PV$.

So ultimately, process (b) will have a higher final volume, and since $T \propto PV$, it will also have a higher final temperature.