1
$\begingroup$

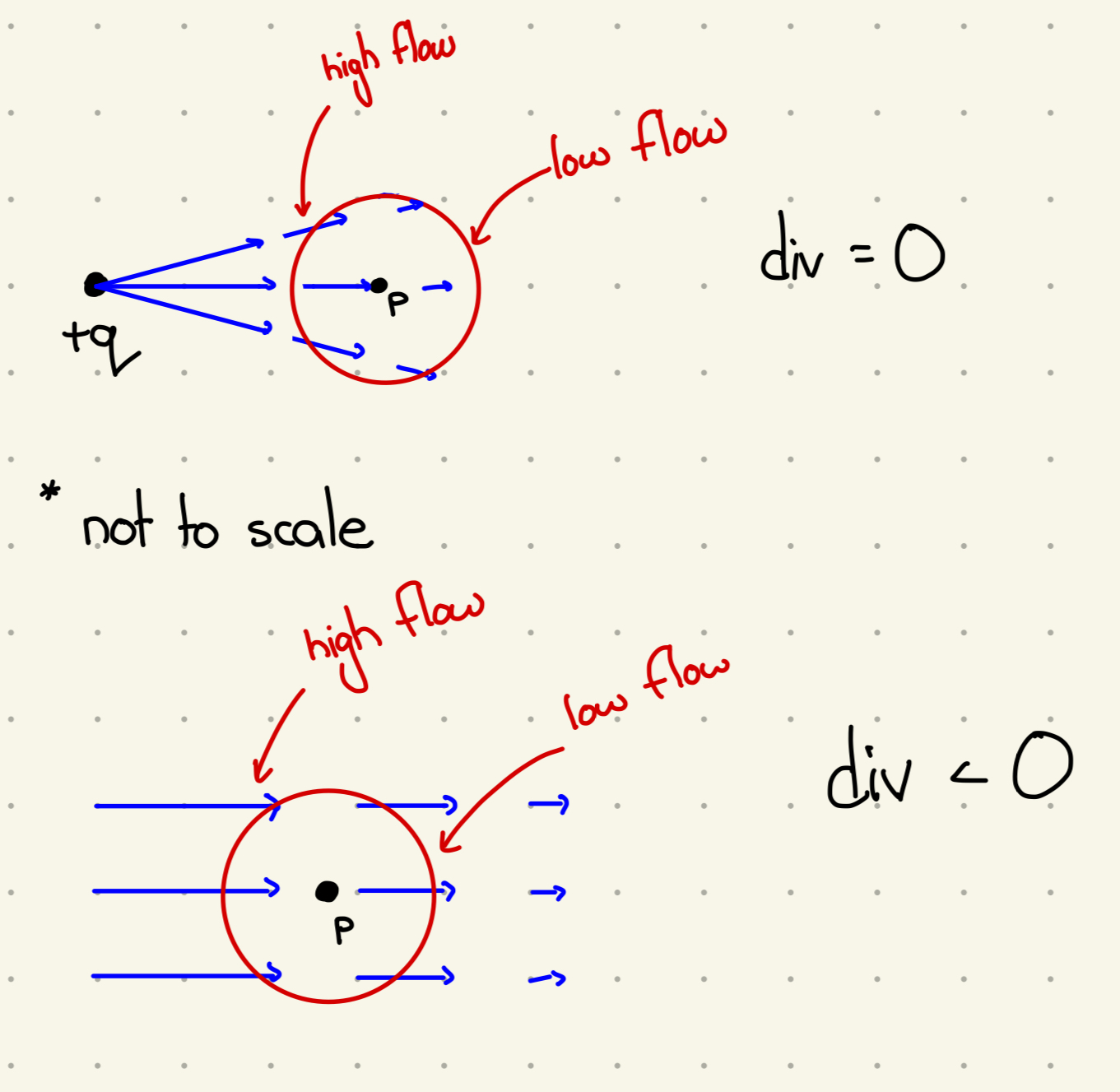
I understand that for a positive point charge at the origin, the resulting electric field extends out radially and has magnitude that decreases by $\frac{1}{r^2}$. However, I do not understand why the divergence (anywhere but the origin) is 0. If I imagine a point in space away from the origin, there are vectors on either side with different magnitudes. When thinking about this like a 'flow', I can't figure out how there is 0 divergence if the flow is decreasing.

I am even more confused when thinking about a field such as $\textbf{F}= x\hat{x}$. Many YouTube videos and textbooks state there is a positive divergence as the flow rate it increasing with $x$. So, why wouldn't the same logic apply for a flow rate decreasing by $1/r^2$ (negative divergence)?

Here is a picture which demonstrates my confusion (I know the field shown is not $\textbf{F}$)

enter image description here

Improve this question
$\endgroup$
1
  • $\begingroup$ The flow per unit area decreases as $1/r^2$, but the effective area for the flow increases as $r^2$. These effects cancel, so flow in = flow out. $\endgroup$
    – d_b
    Commented Nov 22 at 8:23

1 Answer 1

Reset to default
1
$\begingroup$

However, I do not understand why the divergence (anywhere but the origin) is 0. If I imagine a point in space away from the origin, there are vectors on either side with different magnitudes. When thinking about this like a 'flow', I can't figure out how there is 0 divergence if the flow is decreasing.

(emphasis is mine)

Literally thinking of a flow is a good analogy. Let us consider a flow in water, e.g., flow in a bathtub due to water flowing from the tap. There is flow away from the tap, by for any volume that is not right under the tap, the volume of water flowing in through its sides is the same as the flow out through the sides. The only volume where this is not the case is right under the tap (i.e., the source.)

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thank you. I also have imagined that if I draw a sphere around a point in space (away from the point charge), the density of field lines is greater on one side of the circle, but over a smaller area of the circle. Summing up over the circle would result in the next flux being 0, or in other words the same number of field lines entering as exiting. $\endgroup$
    – Ben
    Commented Nov 22 at 8:38
  • $\begingroup$ @Ben I find it easier thinking of a cube, rather than a sphere - you can find many derivations of divergence theorem (or continuity equation) with illustrations like this one. $\endgroup$
    – Roger V.
    Commented Nov 22 at 8:46

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.