Why do telescopes converge light instead of diverge?

Question

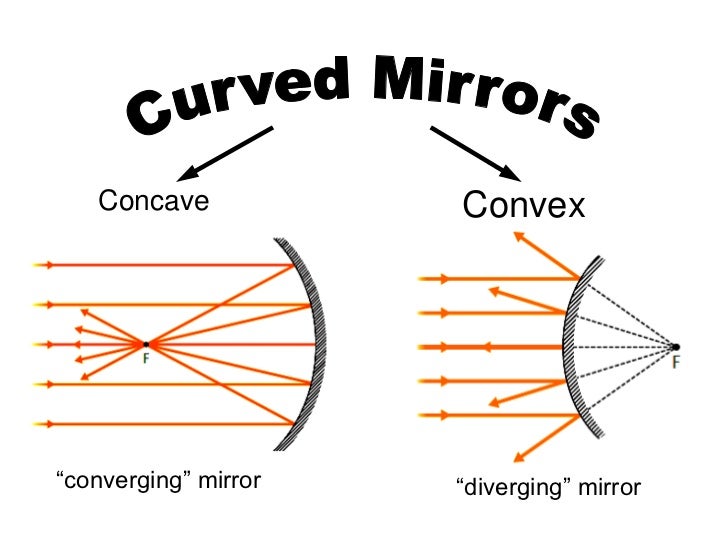
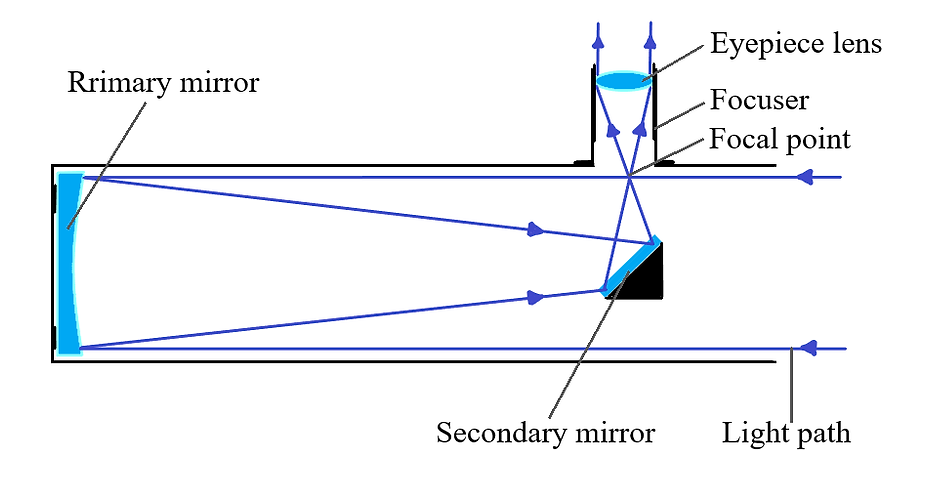
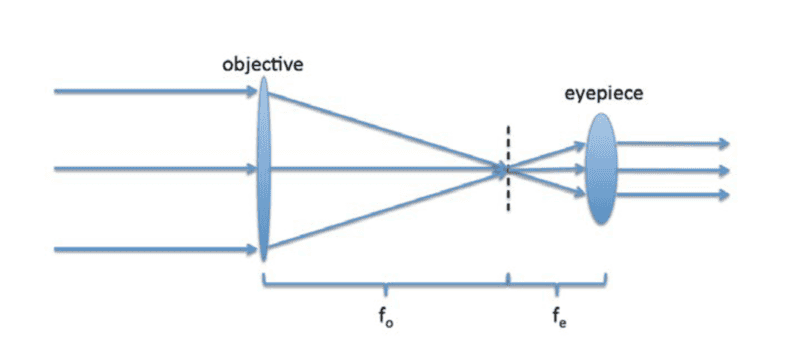
I am an astronomy hobbyist and have a 6" Newtonian at my home. While I was collimating my telescope it hit me that why is there a concave mirror at the back instead of a convex mirror. Why do telescopes converge light instead of diverging them?

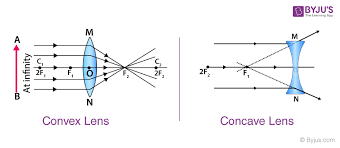
In this diagram it is evident that the concave lens is divergent i.e. the concave lens diverges light. Any two points(let's call them $A$ and $B$)(both the points are present in the light rays) perpendicular to the principal axis will be further separated when the light rays pass through the lens. Therefore $A'B'$ will be larger than $AB$. And hence the image will be magnified.

The same is inversed in the other case where the $l(AB)>l(A'B')$

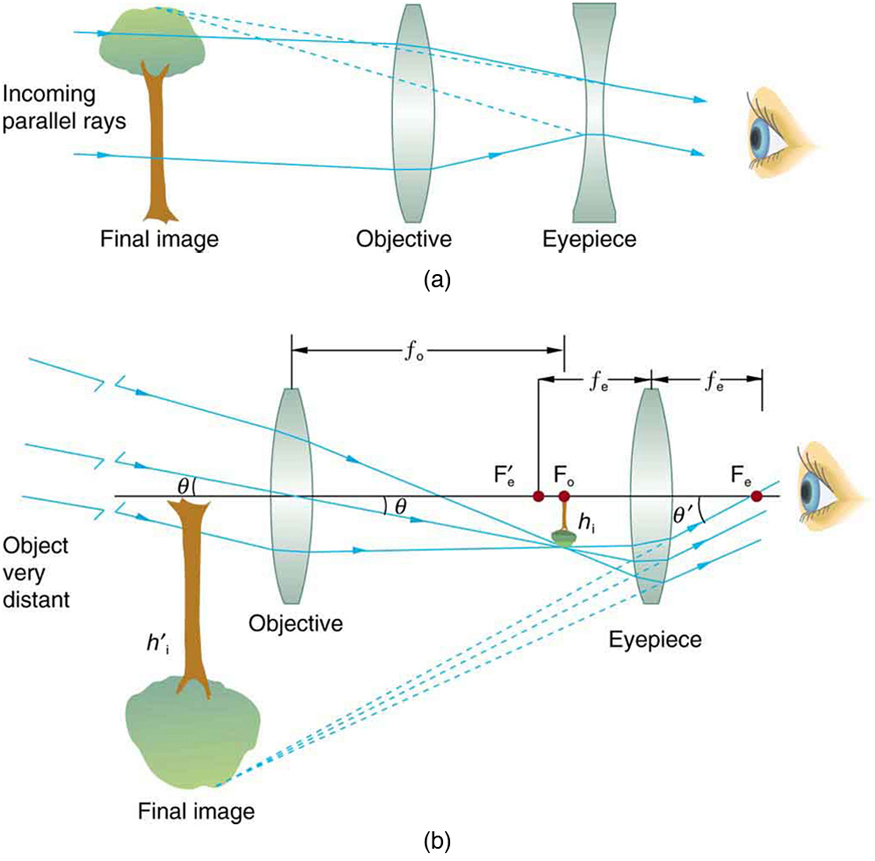
Then why do telescopes converge light instead of diverging them? It is evident that they converge lightrays as the Refractors use a convex lens, A Newtonian uses of concave mirror and a Catadioptric uses both of them.

Answer 1

The Galilean telescope uses concave lens as a eyepiece and the resulting effect following the Galilean construct is to produce an erect image at infinity, shorter than a astronomical telescopy with same power.

To analyse the working of an optical instrument, you can't just look the effect of one lens; instead, you should consider an hypothetical object placed in front of the first lens and to trace the rays, passing all the lens until to form the final image. Look the image below

Image by College Physics. Authored by: OpenStax College. Located at: http://cnx.org/contents/031da8d3-b525-429c-80cf-6c8ed997733a/College_Physics.

In a) we have the case of the Galilean telescope. Observe how the concave lens acts in this case. In b) we have the setup of the astronomical telescope. It forms a small image in front of the eyepiece (usually with approximately the same size of the object) and the effect of the convex eyepiece is to form a bigger final image, as we wish.

Answer 2

From the two images contained in your question you already know that a convex lens converges light and a concave lens diverges light. Now let's look how curved mirrors do.

_{(image from Anin Girls High - Reflection at curved surfaces)}

As you can see, a concave mirror converges light, and a convex mirror diverges light.

Then, by combining a large concave mirror and a small convex lens (both converging the light rays) you get the Newtonian telescope.

_{(image from How does a newtonian reflector telescope work)}

This is functionally very similar to the Keplerian telescope. You get this by combining a large convex lens and a small convex lens (both converging the light rays as well).

_{(image from Physics Forums - Keplerian telescope)}

Answer 3

The reason for a convex lens to cause convergence of light, is that the light path through the center of the lens has an extra time delay, due to the slow speed of light in the thick glass, compared to the rays passing through the thinner regions of the lens edge.

The reason for a concave mirror to cause convergence of light, is that the light path to the center of the mirror and back toward the source has a greater length (causing greater delay) than the light paths to the rim of that same concave mirror.

In both cases, a time delay differential acts to make simultaneous achievement of wave-propagation-times from the incoming beam area (presumed to be a plane wave) at the refractive or reflective focus point.

The effect therefore is of constructive interference of the light that arrives at that point, and high light intensity (squared amplitude) is the result. A third way to achieve convergence of light is by gravitational lensing... which results from altering the passage of time by gravity.

As for why convergence creates a telescope effect, parallel rays (a good approximation of the light from a small object at a distance) go into a telescope, and a broad fan of rays coming from a large image out into a small pupil, is described as light convergence.

Answer 4

Think about how big the aperture to let the light in is, and how small the aperture to look in the telescope is. The large area of light is concentrated to brighten the image; but only the light coming from a certain target.