How do telescopes work as magnifiers?

Question

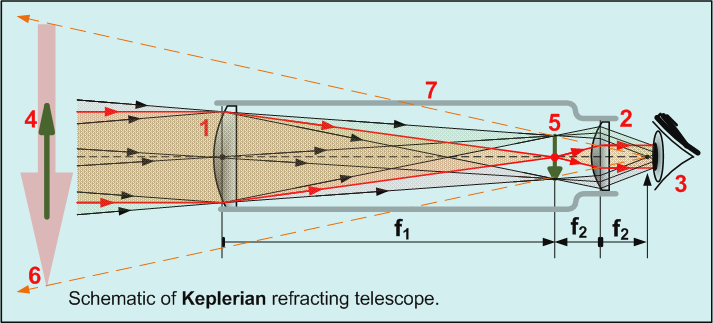
I'm searching a long time for an answer without helpful results. Here are some examples of unhelpful answers:

• The lenses bend the light in such a way that they appear bigger — that's not an answer at all, because the question is what is that “such a way”.

• The eyepiece magnifies the image the same way a magnifying glass does — instead of explaining, he is saying that it's the same as another thing that I may not understand how it works, or why it is the same.

• Describing where the light converge and diverge — it's necessary to explain how do that cause magnification.

• Talking about the advantages of reflecting telescopes over refractive, or other advanced topics, without explaining step one.

The most helpful thing will be, to show two diagrams of the paths of light in a telescope, one diagram of a telescope with a weaker magnification, and one diagram of a telescope with a stronger magnification, so the difference will be obvious.

So my question is why does an objective lens with a longer focal length cause the image to be larger, and why does an eyepiece with a shorter focal length magnify better. And I know that magnification means covering a larger angle when entering the eye. But my question is how do telescopes cause this.

And here is why I don't understand why an eyepiece with a shorter focal length makes the image appear bigger: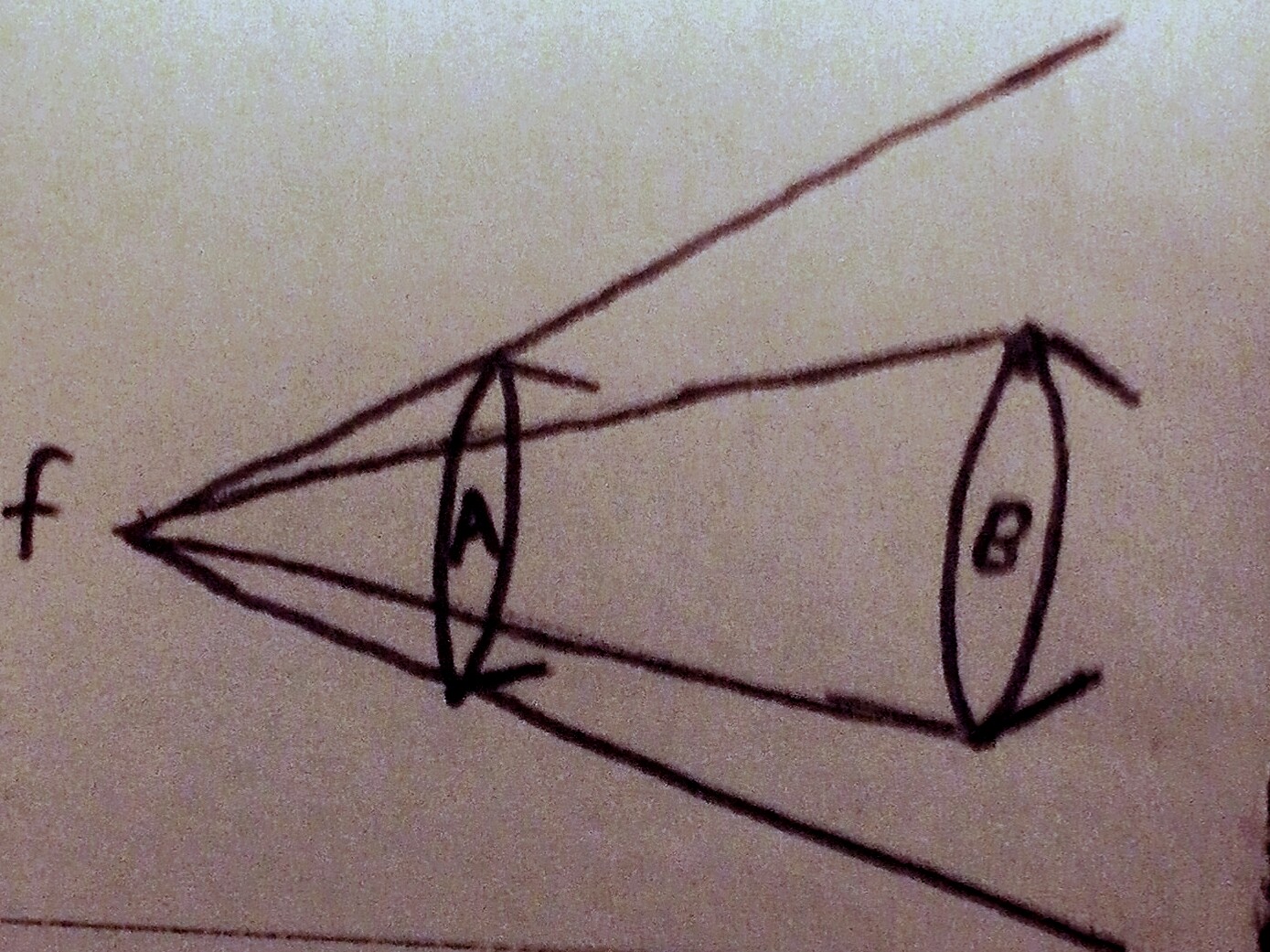 Here f is the focal point, and there are two eyepieces, A and B. It seems that eyepiece B would magnify better for two reasons. First, because it deals with a smaller portion of the image. Second, if both eyepieces have the same bending power, then eyepiece B would give out rays that are stronger converging (because the incoming rays are less diverging), making it appear bigger (covering more arc degrees).

Links to articles or books that answer my question will be highly appreciated.

Answer 1

First I wanted to point out that there is really a focal plane, and not a focal point like I read in many articles. They are misleading.

Here is a diagram of what is happening inside the telescope. The focal plane is at 5. Now let's see the role of the objective lens. Let's imagine that we enlarged the left side of the above diagram, till (and including) the focal plane. What happened? The focal length was enlarged, and the image (=the focal plane) was enlarged. The objective lens was enlarged too, but it doesn't affect the magnification, as seen in the above diagram, that every part of the objective lens gives the image of the same size. The angle of the incoming rays stays the same, meaning that we are viewing the same object.

So now we have: Longer focal length =Bigger image.

Now let's see the role of the eyepiece. What happens when we reduce the size of the right part of the above diagram, till the focal plane? The focal length is shortened, the image is reduced, and the exiting rays stay the same. That means that with a shorter focal length, a smaller image covers the same angle (looks the same) as a larger image would cover with a longer focal length. And since the apparent size depends on the angle between the rays entering the eye, we have: Shorter focal length =Bigger magnification.

Answer 2

With a distant object, the objective lens (or mirror) of a telescope produces a real inverted image at the focal length of the lens. The angle subtended by the image is the same as that subtended by the object. As the focal length gets longer, the image gets bigger. This image can be recorded on film (or detected by a CCD) or be enlarged by passing through other lenses (small object distance to a larger image distance). When being viewed by the eye through a short focal length convex lens, the lens is placed so that the image being viewed is inside the focal length of the lens. This produces a final virtual image which the eye sees at large (and comfortable) distance. Since the original image is now close to the eye it's angular size as seen by the eye is much larger than the angular size of the original object. (A magnifying glass lets the object being viewed stay in focus while being brought closer to the eye.)

Answer 3

Telescopes are about magnifying angles. They make some distant target that subtends an angle $\theta$ look like it subtends $n \theta$, appearing $n$ times larger/closer. If you focus on that idea, telescopes are easier to understand.

The object lens makes an image of the target. Imagine the top and bottom of that. The eyepiece brings light from those points into the eye at different angles: the shorter the focal length, the steeper/larger the angles, and the larger the angular magnification.

Those angles are also steeper if the object lens has made a larger image, so a larger image also increases magnification. Longer objective focal lengths do this, because the image is farther from the lens: the light coming in at the target’s fixed angles can spread out to form a larger image.

In formulae, for a target of angle $\theta$:

The image size is $h_i = f_o \theta$

The eye sees an angle of $h_i / f_e = f_o/f_e \theta$ so the magnification is $f_o / f_e$