4
$\begingroup$

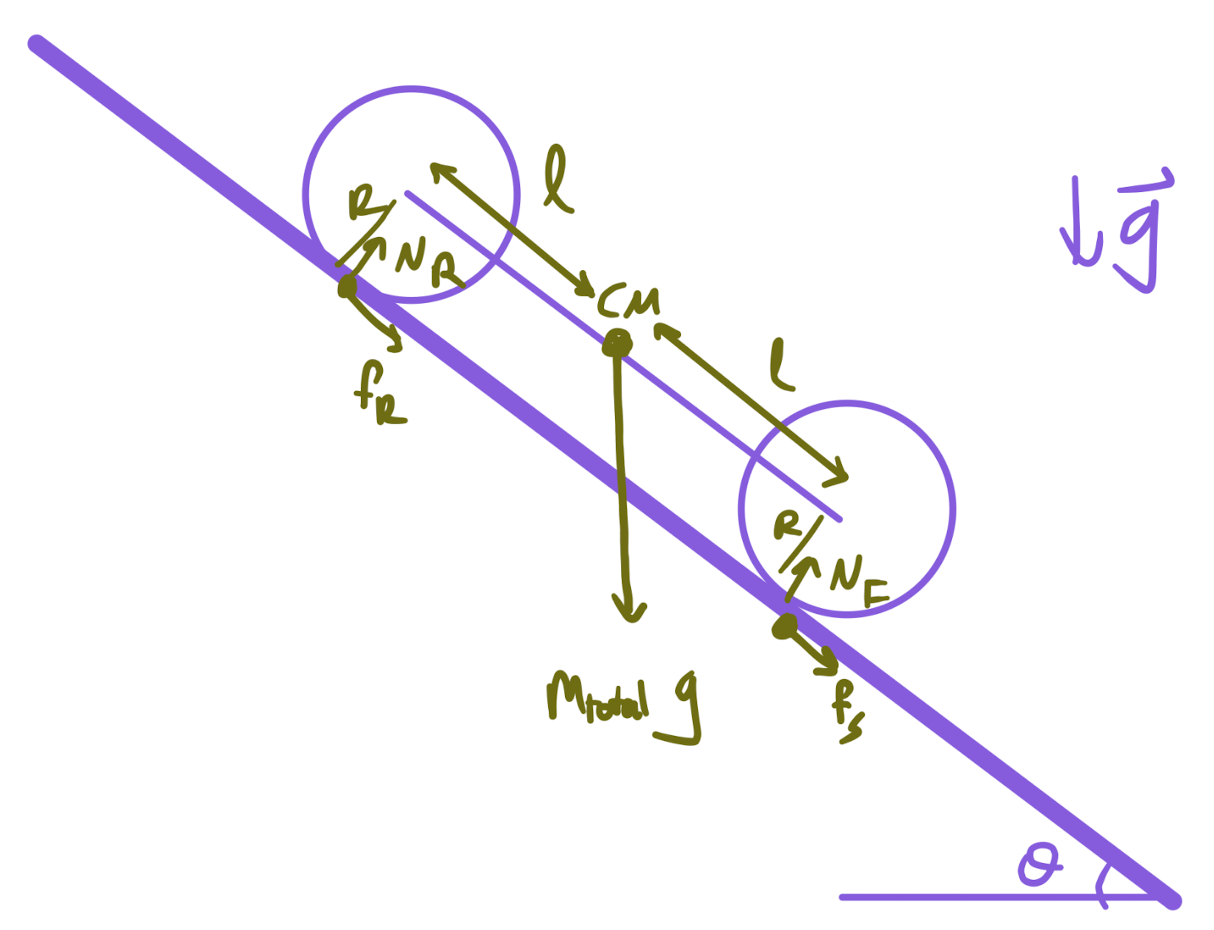
Our professor has solved a problem in class regarding normal forces on a wheel moving without slipping with acceleration (assume the wheel is put on a ramp with gravity acting on it). Afterwards, we added another wheel connected to the first one by their axes. Analyzing the net torque on this 2 wheel 1 rod system yields different normal forces for each wheel, regardless of the mass of the connecting rod (assuming total mass of the system is constant).

This sounds counterintuitive to me since I can't see the difference between this system with an arbitrarily small rod mass, and 2 wheels rolling down separately without a rod between them, since all the rod does (in my opinion) is giving us the constraint that the distance between the centers of the wheels stay constant, a constraint already satisfied in the 2 separate wheels case naturally, assuming same initial speeds.

2 Wheels 1 Rod System

The calculation we did to find normal forces is as follows:

$\tau_{CM}=N_Fl-N_Rl+f_RR+f_FR=0$

$f_R+f_F+m_{total}g\sin(\theta)=m_{total}a$

$N_F+N_R=m_{total}g\cos(\theta)$

From these equations we calculate different $N_F$ and $N_R$, something I'm having trouble understanding.

Furthermore, after analyzing the forces on the wheels individually, I find that there should be a net torque on the rod, which doesn't make sense given the system's movement. This is, however, a different question from what I'm asking for here.

How do I make sense of this result, or is there something else missing?

Improve this question
$\endgroup$
8
  • $\begingroup$ I think the problem is that your equation for torque about the center of mass is incorrect. The two N * l terms are appropriate if N is being applied at the wheel centers, not at the contact points. Each f * R term gives the torque about the wheel center, not about the center of mass. $\endgroup$
    – BaddDadd
    Commented Oct 20 at 16:41
  • $\begingroup$ @BaddDadd Are you sure about that? If I'm not mistaken, taking the cross product of the Force times Distance gives the values stated in the equation for the torque around the center of mass of the system. $\endgroup$
    – HideBehind
    Commented Oct 20 at 17:52
  • 1
    $\begingroup$ Not sure the second equation is correct. Surely the force on the wheels/rod system parallel to the slope is $f_R+f_F+m_{total}g \sin \theta$ ? $\endgroup$
    – gandalf61
    Commented Oct 20 at 18:23
  • $\begingroup$ @gandalf61 Whoops you're correct, edited it now! $\endgroup$
    – HideBehind
    Commented Oct 21 at 11:46
  • 1
    $\begingroup$ Maybe I'm missing something, but I don't see any difference between the normal forces acting on each wheel due to the weight of each wheel when they are independent, and the normal forces acting on each wheel when connected by the rod if the rod is massless. The only difference seems to be the total force acting perpendicular to the incline. On the other hand, if the rod has mass, its weight is supported by the wheels adding to the normal force of each. $\endgroup$
    – Bob D
    Commented Oct 22 at 12:25

2 Answers 2

Reset to default
2
$\begingroup$

The calculation we did to find normal forces is as follows:

$\tau_{CM} = N_Fl - N_Rl + f_RR+f_FR=0$

I disagree with the above. Why are you assuming the total torque is zero?

Note the the wheels are accelerating, therefore there must be net torque on the vehicle. It's just that the torque is going into rotation of the wheels and not into rotation of the vehicle. The total angular momentum of the vehicle is not constant, so a non-zero torque exists.

From a comment:

Our professor said that this ramp situation was equivalent to a braking/accelerating car, and braking/accelerating cars do indeed have different normal forces on the front and rear wheels (and not just because of the mass of the car's body).

The difference is that the brake or the transmission is coupling the torque applied to the wheel to the "vehicle". In your scenario with no brake/transmission, the vehicle does not pitch and there is no reason for the normals be different.

Rolling down the hill is not identical to a vehicle accelerating from internal torque.

Improve this answer
$\endgroup$
7
  • $\begingroup$ I think since the whole system is not turning as a whole with respect to its center of mass there shouldn't be any net torque, however, we should find a net torque if we look at the wheels individually as a subsystem. Correct me if I'm wrong in thinking this way. $\endgroup$
    – HideBehind
    Commented Oct 22 at 8:57
  • $\begingroup$ "therefore there must be net torque on the vehicle". Where does the OP say anything about a vehicle? The normal force acting on each wheel can be due simply to the weight of each wheel. $\endgroup$
    – Bob D
    Commented Oct 22 at 12:16
  • $\begingroup$ @BobD "vehicle" == "system". I don't think anything in my answer says anything about normal forces, so I'm not sure how to interpret your comment. $\endgroup$
    – BowlOfRed
    Commented Oct 22 at 15:52
  • $\begingroup$ @BobD in case it's not clear, I'm pointing out the error in the OP's logical chain that led to the conclusion that the normals are different. The normals do not have to be different. $\endgroup$
    – BowlOfRed
    Commented Oct 22 at 15:55
  • $\begingroup$ @BowlOfRed are you sure there is a net torque on the system? There is net torque on the wheels, that's apparent. However, the system itself isn't rotating with respect to its center of mass. $\endgroup$
    – HideBehind
    Commented Oct 22 at 20:44
2
$\begingroup$

enter image description here

Clearly $N_F l > N_R l$ in the above image, but this is only because the brakes are being applied. If there is no braking force, then $N_F = N_R.$

$N_F + N_R = m_{total} g \cos(\theta)$ From these equations we calculate different NF and NR, something I'm having trouble understanding.

If $N_F + N_R = m_{total} \ g \cos(\theta) $
then $N_F = N_R $
is a perfectly valid solution.

Since the connecting rod is not rotating we can conclude the net torque on the rod is zero. This means:
$$N_F l - N_R l = 0 $$ $$N_F = N_R $$

Your own argument in the OP that a massless rod connecting the wheels does not change the normal forces, is absolutely correct and is a perfect argument to support the conclusion that $N_F = N_R $. I am not sure why you are convinced that $N_F \ne N_R$? Is that what your teacher said the answer is?

The torque ($m \ g \ \sin(\theta) R $) that acts on the wheels, that causes their angular momentum to increase as they roll down the slope, does not act on the connecting rod or make the normal forces on the wheels unequal, if there is no friction between the wheels and the connecting rod.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Yep, the professor did say that. He included friction forces in the calculation of the net torque on the vehicle, so he found that $N_Fl-N_Rl+f_RR+f_FR=0$, thus $N_F \not= N_R$. I think there needs to be a friction force between the wheels and the connecting rod, which is why this system behaves differently than 2 separate wheels. $\endgroup$
    – HideBehind
    Commented Oct 22 at 20:39
  • $\begingroup$ Looking back at this answer after reading the other one, I think I understand now the logic behind it. You're correct that friction between the rod and the wheels are necessary for the rod and wheels to influence each other. $\endgroup$
    – HideBehind
    Commented Oct 22 at 23:32

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.