Debye Theory Ensemble Perspective

Question

I am learning the Debye Model and am having trouble understanding how to interpret it within the framework of statistical mechanics.

I understand that in the Einstein model, we consider a solid as our system, and then consider one atom in that solid as a closed subsystem, i.e. energy can pass between that atom and the rest of the lattice. We can then treat this setup using the canonical ensemble. Letting the atom be a quantum SHO which is independent of any other atom in the lattice and has a fixed frequency parameter $\omega$, we see that its microstates are just the quantized excitations of the oscillator, and from there we can find the partition function and all of the other fun quantities such as $\langle E\rangle$, $C_v$, etc.

In the Debye model, we make some fundamental changes to the model: atoms are now coupled and so we can no longer consider just one atom as its own independent subsystem, so the previous approach using the canonical ensemble will not work. However, we can turn to the grand canonical ensemble and one of its main results, which is that if we treat an actual state of a system as an open subsystem, we can find a distribution function associated with it which depends on the type of particles which can occupy it (leading to the Bose-Einstein, Planck, Fermi-Dirac, and Boltzmann distributions). This is where my confusion starts. From what I've read online:

• oscillations in the lattice are carried by the displacement of atoms from their equilibrium position, and there are three possible modes of displacement: 1 longitudinal and 2 transverse
• oscillations in the lattice must adhere to the boundary condition of being periodic, which introduces a quantization of the wavenumber $k_n$ of the allowed oscillations
• because we care about macroscopic bulk quantities (e.g. heat capacity), the boundary shape of our model shouldn't actually matter, so we can choose to make our solid a perfect cube with sidelength L
• each oscillation with a unique wavenumber can be considered a state (why?) which is occupied by 'bosons' (why?) so we can apply our distribution results to each one to see that it has an average of $n_B(E)$ bosons occupying it
  • I suspect this last answer has to do with the fact that increasing the level of a harmonic oscillator is somehow "the same as" (why?) populating it with another boson with the energy identical to the oscillator's energy spacing $\Delta E = \hbar \omega$: is this only an interpretational thing or does this manifest in a physical way?
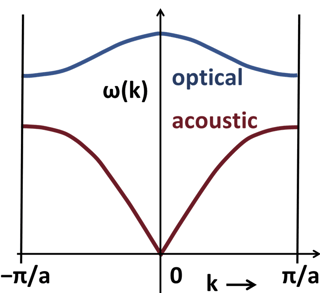
• we now consider the regime where the dispersion relation is roughly linear...my understanding is that the dispersion relation can be derived analytically from considering the mechanical equations of motion of the atoms in the solid, but my wave theory is really shoddy so I'm taking it as given for now that $\omega = v_s |\vec{k}|$
• we can find the density of states in quantization space, $g(n)$, and then do a transformation to get the density of states in k space ($g(n)dn = g(k)dk$) or energy space as needed
• the allowed wavenumber quantization is limited by an upper bound: because the lattice is discrete, the wave is a sinusoid which is sampled at discrete positions (the position of each atom), and if the wavelength is too small, aliasing will occur and the wave will be nonphysical

To summarize, my questions are:

• Why can each oscillation with a unique wavenumber be considered a state?
• Why can this state be "occupied" by some type of boson?
• How we know that the "normal modes" of the lattice are all of the states that need to be considered?
• Do these answers follow somehow from the Hamiltonian of the system? This question sort of touches on this.

Answer 1

Normal modes
Let us first take a classical view of coupled oscillators - e.g., balls connected by springs or atom in a lattice (which, for small displacements, can be reduced to the balls coupled by springs.) Classical mechanics teaches us that we can find the normal oscillation modes of such a system, and represent any oscillation as a superposition of such normal modes. Any single mode then obeys a harmonic oscillator equation: $$ \ddot{Q}_k(t)+\omega_k^2Q_k(t)=0,\\ H_k=\frac{P_k^2}{2}+\frac{\omega_k^2Q_k^2}{2}. $$ See here for an example of a dispersion relation that we can get:

Quantization
If we consider the same problem from the point of view of quantum mechanics, we expect that the excitations will be quantized. The quantization of Harmonic oscillator is a well-known problem - each mode will have discrete energies: $$ E_k=\hbar\omega_k\left(n_k+\frac{1}{2}\right), $$ where $n_k$ characterizes the level of excitation of modes. Note that, given the finite crystal size (i.e., the finite number of atoms - say $N$ atoms) we have finite number of modes, $k_1,...,k_N$, but the excitation numbers can be arbitrary.

Second quantization
We now can perform second quantization or, in a simpler and oscillator-specific language, describe the modes in terms of raising and lowering operators: $$ H_k=\hbar\omega_k\left(a_k^\dagger a_k+\frac{1}{2}\right). $$ Note that the number of the oscillators is still $N$, but the operators now work in the Fock space (filling numbers space), so the level of excitations can be arbitrary.

Phonons
The last step is calling these new excitations - phonons. In this case, $a_k^\dagger, a_k$ are no more raising and lowering operators, but phonon creation and annihilation operators, whereas $n_k$ becomes a number of phonons excited in mode $k$. In many ways phonons behave just any other particles, although for the sake of caution one calls them quasiparticles. There exist many other similar excitations, which are obtained from oscillatory modes - plasmons (for electron plasma in metals), magnons (for spin waves), polaritons (in Bragg lattices or cavities), etc.

Synthesis
In other words, the canonical ensemble in Einstein model is applied to a finite number of atoms, while the grand canonical ensemble in the Debye model is applied to the quantized excitations of the normal modes (phonons)

Note the significance of every word in term quantized excitations of normal modes:

• normal modes are not atoms themselves, but collective emotion of the atomic chain
• excitations are mode energy - the number of modes is finite, but the energy of excitations is not.
• quantized - the energy of the modes is discrete, rather than continuous - the discreteness of mode energy is no less crucial here than in photo-effect or Planck formula (without it, the energy would diverge.)

Related
Is differentiating particle and quasiparticle meaningless?
Do quasiphotons have mass?

Reading
Charles Kittel, Introduction to solid state physics - standard text
H. Haken, Quantum field theory of solids - an accessible introduction to solid state physics from quantum quasiparticles viewpoint

Answer 2

Just to say: although many presentations bring in phonons and grand ensemble to deal with this, you do not need either. You simply treat the vibrations as a collection of normal modes. Each normal mode behaves like a quantum harmonic oscillator. These normal modes are distinguishable systems. You just have a collection of them. They are weakly interacting, which means they can be treated separately and the total energy of the system is simply the sum of all the contributions.

Again: no need for phonons, no need to mention indistinguishability (or any particles at all), no need for grand canonical ensemble.

This method handles the quantum field theory exactly and correctly.

I have a set of lecture notes on subjects like this which you can find on my Oxford University website.