1
$\begingroup$

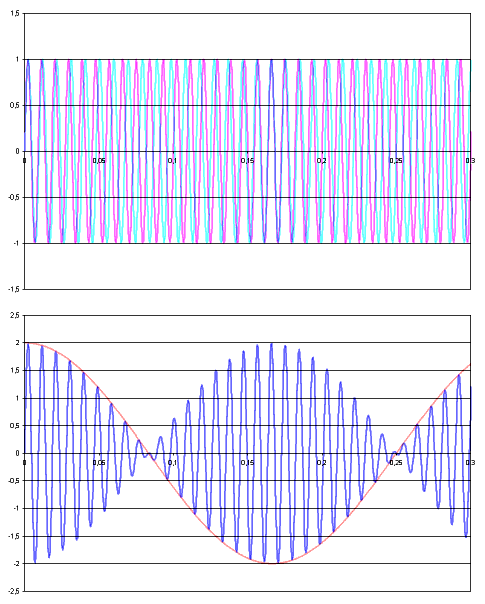
According to what I have been reading in various places, when we combine two close non-harmonic frequencies, we get the beating effect and a resulting frequency as the average of both frequencies. That is, if we have a $500 \,\text{Hz}$ signal and a $502\,\text{Hz}$ signal, we get a combined signal of $501\,\text{Hz}$. However, I can't understand how we can get a constant frequency signal of $501\,\text{Hz}$ if we have two signals of different frequencies. In other words, those two signals combined cannot be equal to emitting a single $501\,\text{Hz}$ signal.

I have tested this with some tuners and they seem to give $501\,\text{Hz}$, but I think it is an inability or inaccuracy of the plugins to understand the phenomenon. Furthermore, if we take a $500\,\text{Hz}$ signal and a $702\,\text{Hz}$ signal, do we get a $601\,\text{Hz}$ signal? It doesn't make sense. Besides, music analysis plugins understand it differently when there are more distance between frequencies.

Two signals, unrelated by their harmonics, should be changeable on an oscilloscope, right? How do you calculate the resulting wave frequency? (In these examples I wouldn't be interested in the amplitude difference of the beating effect, just let's talk in frequency terms).

Improve this question
$\endgroup$
9
  • 5
    $\begingroup$ $\sin(a) + \sin(b) = 2 \sin((a + b)/2) \cos((a - b)/2)$ $\endgroup$
    – John Rennie
    Commented Sep 25 at 8:52
  • 1
    $\begingroup$ when you combine a 500Hz and a 502Hz signal you do not get a 501Hz signal; instead, you get a signal exactly consisting of one 500Hz and one 502Hz oscillation. It is true that if the frequency separation between them is small compared to their average, then you can view the combination as an amplitude modulated signal whose carrier is 501Hz with amplitude modulation rate (beat) 1Hz, but that model is does not work for wideband separation. $\endgroup$
    – hyportnex
    Commented Sep 25 at 8:52
  • $\begingroup$ This doesn't seem like a homework problem at all. Voting to reopen. $\endgroup$
    – Michael Seifert
    Commented Sep 25 at 14:05
  • $\begingroup$ @hyportnex, I think your comment blurs the distinction between human physiology and physics. If you add two pure, acoustic tones that are close enough in frequency, human ears perceive a single, modulated tone, half-way between the two frequencies. If they're farther apart, the person simply hears the two separate tones. But mathematically, there is no difference between the sum of the the two pure tones, and actual, physical, AM modulation of the center frequency. You can get the same exact waveform by either method. $\endgroup$
    – Solomon Slow
    Commented Sep 26 at 15:33
  • $\begingroup$ @SolomonSlow you are right in that mathematically there is no difference between wide or narrow separation of two tones, but there is a great deal of physical or engineering difference between the two situation when either generation or detection of such tone combination is to be involved. Speaking "engineeringwise", how would you modulate the amplitude of a 500Hz (or 500kHz) signal with a 499.99Hz (or 499.99kHz) signal and detect it after suppressing the carrier? $\endgroup$
    – hyportnex
    Commented Sep 26 at 16:27

1 Answer 1

Reset to default
2
$\begingroup$

Beating is not the same as a monochromatic signal at frequency $\omega$. Assuming $\omega\ll\Omega$: $$\cos((\omega + \Omega)t)+\cos((\omega - \Omega)t)=2\cos(\omega t)\cos(\Omega t), $$ that is adding two monochromatic signals of type $\cos((\omega\pm \Omega) t)$ produces a signal with carrying frequency $\omega$ whose amplitude is slowly varying in time as $\cos(\Omega t)$. This is what we call beating - it is clearly not the same as simply $\cos(\omega t)$.

In case of a music signal, beating would be perceives as a constant tone, whose intensity is varying in time.

In communication we usually have a signal at very high radio or optical frequency (i.e., ranging from $10^6$Hz to $10^{15}$Hz) modulated by a much lower frequency acoustic signal - such as music or human speech ($~10-10^4$Hz). The modulation is achieved either by varying the amplitude (AM modulation) or by varying frequency or phase (FM and PM.) Since the modulating frequency is much lower than the carrying frequency, we would often treat the radio signal as a monochromatic, when describing its propagation, dispersion, etc.

enter image description here
(image source)

Remark
Many examples of acoustic beating can be found here. It is instructive to compare, how the beating of close frequencies is resembling a single tone with rising and lowering amplitude, while for two not very close frequencies, the presence of two distinct tones becomes obvious.

Improve this answer
$\endgroup$
6
  • $\begingroup$ Thank you for the answer! I think I understand the formula. In fact, I read this post explaining it, dsprelated.com/showarticle/189.php but I keep missing a deep understanding why we have a carrier with a kind of static frequency at 501Hz and we don't say that this is the same of a single sine signal of 501 Hz (in terms of frequency, not amplitude where the difference is clear). If we pick one cycle of the signal, we are saying that we have a 501Hz signal and that has to be false, we don't have same 'signals' in terms of frequency. There is something missing that I don't understand $\endgroup$
    – Logan
    Commented Sep 25 at 10:15
  • $\begingroup$ @user432591 in one case the signal is monochromatic (there is nothing but this frequency), whereas in the other case it is modulated - the frequency is only the carrying frequency. The key here is that the carrying frequency is much higher than that of the envelope, so for one period the modulation is unnoticeable. More generally we have a packet - in Fourier space the frequencies are clustered around a central frequency, and the width of the distribution is much smaller than this frequency. $\endgroup$
    – Roger V.
    Commented Sep 25 at 10:46
  • $\begingroup$ It still sounds counterintuitive for me but your answers and this example help: "Consider, for example, what happens when we play two tones with frequencies 400 Hz (approximately the note G4) and 500 Hz (approximately the note B4). The resultant waveform will look rather like a wave of 450 Hz whose amplitude varies at a rate of 100 times per second" I understand the amplitude modulation and the psychoacustic effect, but my thought was that the carrier frequency should be frequency modulated from 400Hz and 402Hz, that was the final wave in my imagination, not a carrier wave in 401Hz.... $\endgroup$
    – Logan
    Commented Sep 25 at 12:07
  • $\begingroup$ My problem is if I make a signal of 401 Hz with that amplitude modulation, do I get that 400Hz and 402Hz in a spectrum analyser? How is that possible? Very counterintuitive for me... Sorry. And in terms of 1 cycle, my oscilloscope becomes crazy when I have a signal of 500 Hz and another one of 702Hz and I can't see any waveform (maybe due to the fast amplitude modulation?) $\endgroup$
    – Logan
    Commented Sep 25 at 12:07
  • $\begingroup$ @user432591 spectrum analyzer is essentially performing expansion in Fourier harmonics (i.e., the inverse of the first equation in the answer above), in other words, it represents any signal as a sum of sine and cosine waves. So the analyzer will show two frequencies (if it has high enough resolution.) $\endgroup$
    – Roger V.
    Commented Sep 25 at 12:09

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.