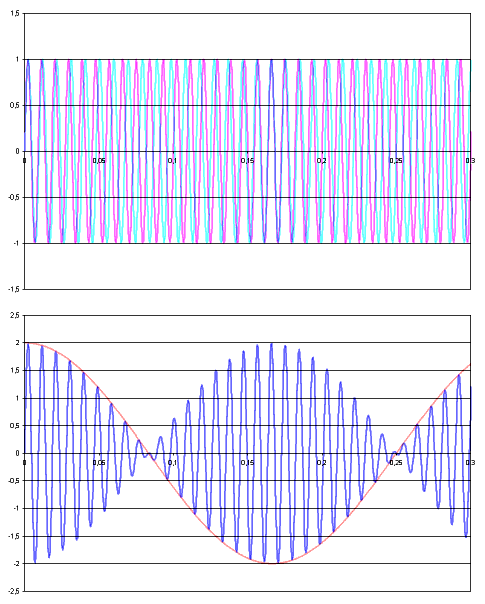
Beating is not the same as a monochromatic signal at frequency $\omega$. Assuming $\omega\ll\Omega$: $$\cos((\omega + \Omega)t)+\cos((\omega - \Omega)t)=2\cos(\omega t)\cos(\Omega t), $$ that is adding two monochromatic signals of type $\cos((\omega\pm \Omega) t)$ produces a signal with carrying frequency $\omega$ whose amplitude is slowly varying in time as $\cos(\Omega t)$. This is what we call beating - it is clearly not the same as simply $\cos(\omega t)$.
In case of a music signal, beating would be perceives as a constant tone, whose intensity is varying in time.
In communication we usually have a signal at very high radio or optical frequency (i.e., ranging from $10^6$Hz to $10^{15}$Hz) modulated by a much lower frequency acoustic signal - such as music or human speech ($~10-10^4$Hz). The modulation is achieved either by varying the amplitude (AM modulation) or by varying frequency or phase (FM and PM.) Since the modulating frequency is much lower than the carrying frequency, we would often treat the radio signal as a monochromatic, when describing its propagation, dispersion, etc.
Remark
Many examples of acoustic beating can be found here. It is instructive to compare, how the beating of close frequencies is resembling a single tone with rising and lowering amplitude, while for two not very close frequencies, the presence of two distinct tones becomes obvious.