The electric potential at the center on the axis of an electric dipole is zero as the potentials due to both the charges of the dipole cancel out. Imagine I place a positive charge at this point. It begins to move towards the negative charge because the field is directed from positive to negative. My question is, if there is zero potential at the center, why does any charge begin to move? Does it not have zero potential energy and thus no place to fall? How do we explain this in context of energy? I know I am missing something here. What am I missing here?
$\begingroup$
$\endgroup$
Add a comment
|
5 Answers
$\begingroup$
$\endgroup$
For a test charge to remain still in an electric potential the requirement is that it is at a stationary point of the potential, aptly named. A stationary point is one where the gradient of the potential is zero, not necessarily a point where the potential itself is zero. In the middle of an electric dipole, the gradient $\nabla\phi$ points towards the positive charge, and a positive test charge will then be accelerated in the opposite direction (where the potential decreases).
Energetically this just means that zero potential energy is not the minimum. Moving towards the negative charge results in negative potential energy, which is less than at the center of the dipole, so this is energetically possible.
Also, since the mechanics are only determined by differences in potential, we could simply add a constant to the potential, which would shift the points where the potential is zero. Since this can't make a difference in the physics, since all the differences in potential remain the same, the zeros of the potential can't have any physical significance.
$\begingroup$
$\endgroup$
It moves because it can achieve lower potential by going near the negative charge.
$\begingroup$
$\endgroup$
3
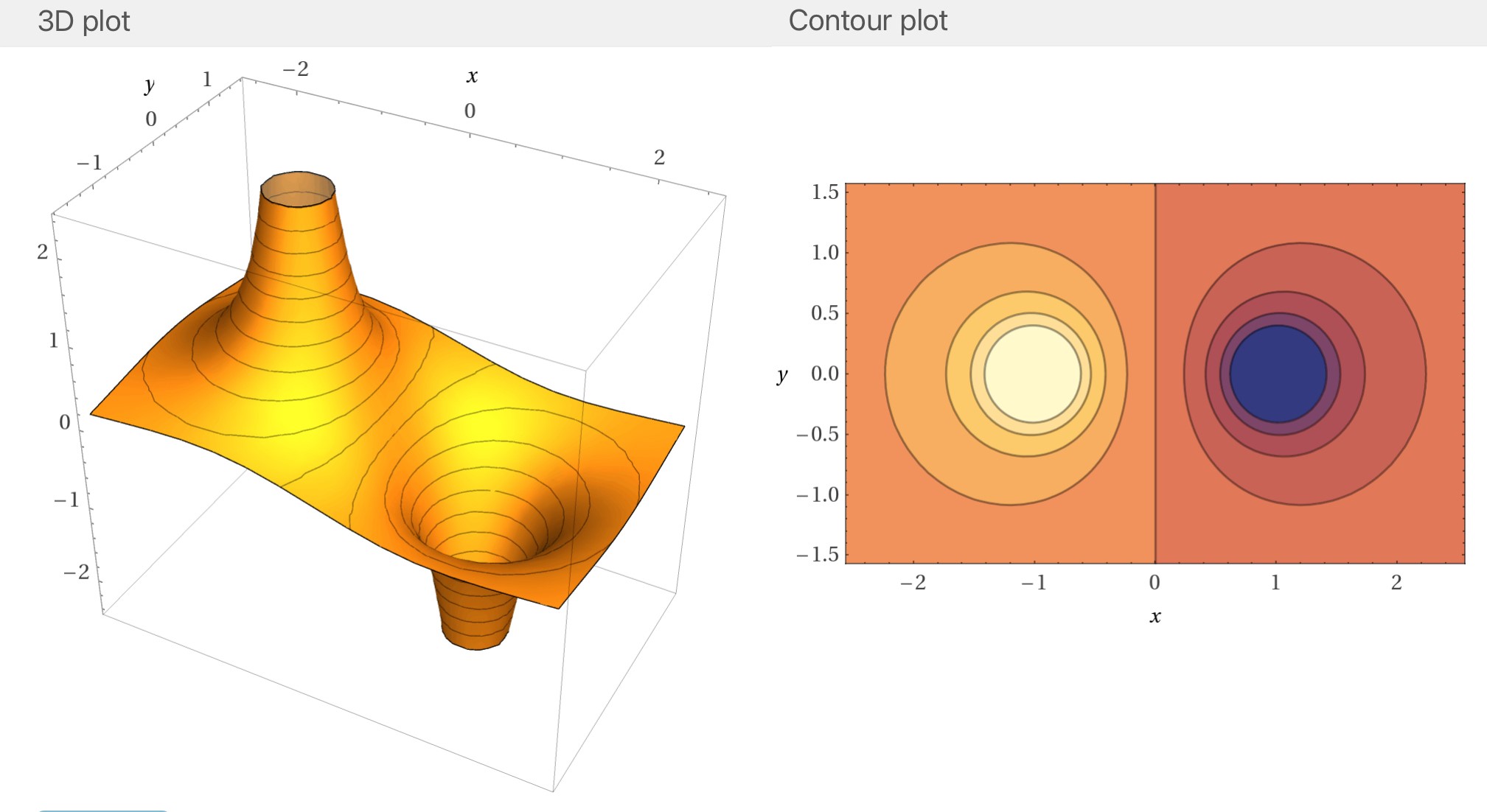
A plot of potential $\phi$ in the xy-plane for two opposite sign equal magnitude charges situated at $(\pm 1, 0)$ shows the along the $x=0$ line the potential (zero) does not change, ie no force, but it does change along the $y=0$ line the potential is changing at $x=0$, ie there would be a force due to an electric field, $\vec E = -\nabla \phi$, on a positive test charge towards the negative charge at $x=1$.
-
$\begingroup$ great job!!!, how did you make the graphs though? they're so good and well made! $\endgroup$ Commented Sep 17 at 8:28
-
$\begingroup$ WolframAlpha and choose show contour lines rather than show mesh. $\endgroup$– FarcherCommented Sep 17 at 8:38
-
$\begingroup$
$\endgroup$
My question is, if there is zero potential at the center, why does any charge begin to move?
The potential is zero because it requires no work to bring a test charge from infinity to a point in the middle of the dipole along the equipotential path shown here: Work required to bring a charge from an infinite distance away to the midpoint of a dipole
But that doesn't mean the charge won't move if placed at the center of the dipole as there is an electric field there directed perpendicular the line of equal potential.
Hope this helps.
$\begingroup$
$\endgroup$
Lower the potential energy, lower the energy, more stable the system. Negative is less than zero, so moving towards negative potential is stabler than staying at zero potential.
