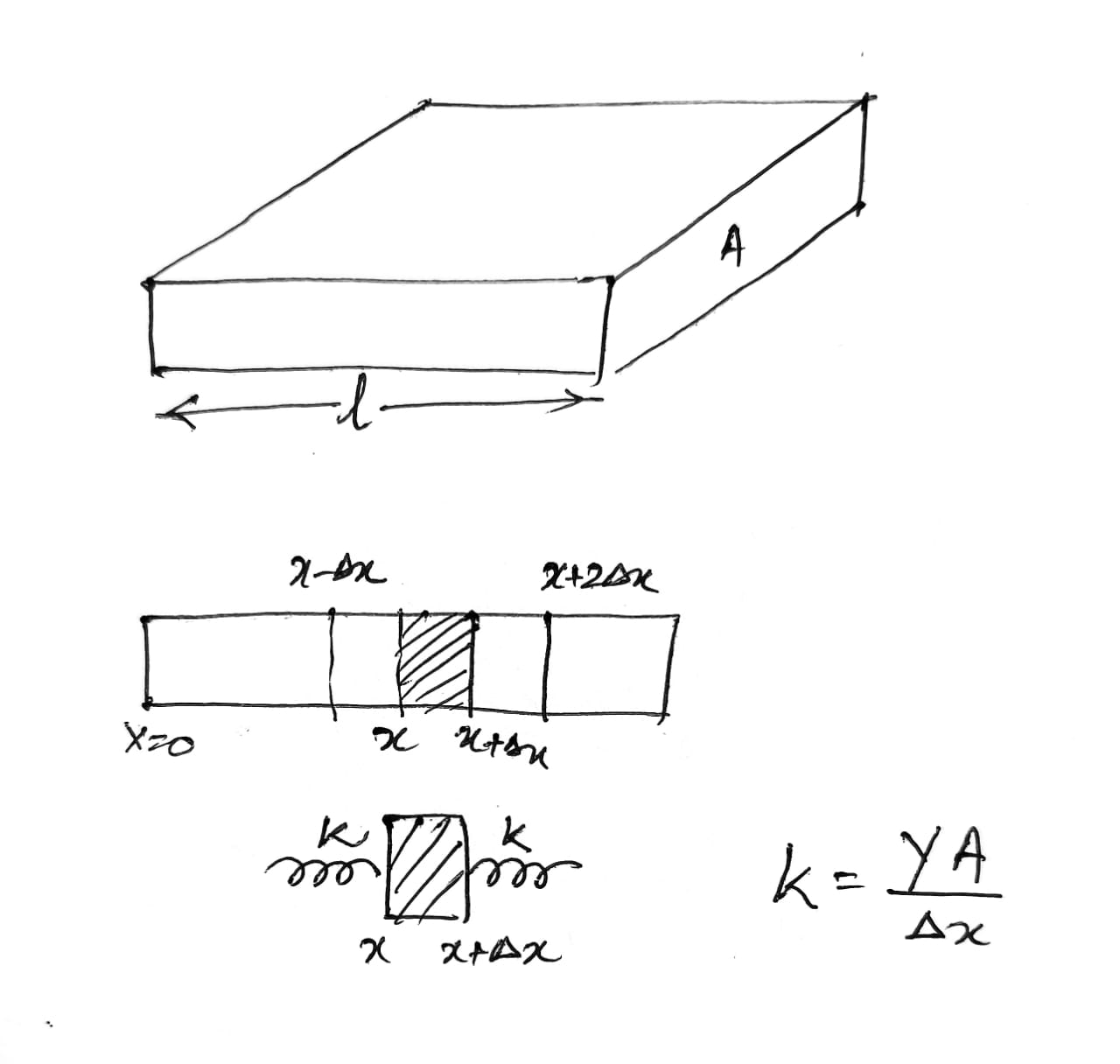
To derive the differential equation for longitudinal waves, my professor proceeded like this:
We are using the concept of $N$-coupled oscillators. Consider a slab of length $l$ and cross sectional area $A$. Let $\xi(x)$ denote the displacement of particle at position $x$ from mean position. We consider an elemental slab from $x = x$ to $x = x + \Delta x$. Its mass $\Delta m = \rho A \Delta x$ where $\rho$ is volume mass density.
$$\rho A \Delta x \frac{\partial^2 \xi}{\partial t^2} = \frac{YA}{\Delta x} \left ( \underbrace{-\xi(x+\Delta x, t) + \xi(x+2\Delta x, t) - \xi(x,t) + \xi(x-\Delta x, t)}_{(*)} \right ).\tag{1}$$ So we get, $$\boxed{\rho \frac{\partial^2 \xi}{\partial t^2} = Y \frac{\partial^2 \xi}{\partial x^2}.}\tag{2}$$
My doubt begins here. I believe $(*)$ isn't correctly simplified. I can show this with any of these two results:
(1) Taylor's theorem
(2) LMVT with a useful result: $f(a+h) - f(a) = hf'(a+\theta h)$ for some $\theta \in (0,1)$. Now $\lim_{h \rightarrow 0} \theta = \frac{1}{2}$.
Proceeding with (2), $(*)$ simplifies to $$\Delta x \xi'(x+1.5\Delta x, t) - \Delta x \xi'(x - 0.5\Delta x, t)$$ which further simplifies to $\color{red}{2}(\Delta x)^2 \xi''(x,t)$. So it must have given then $$\rho \frac{\partial^2 \xi}{\partial t^2} = \color{red}{2}Y \frac{\partial^2 \xi}{\partial x^2}.$$
What is wrong in the derivation then?