Current through points with no voltage drop

Question

I've had a problem with switches in between parallel circuits.

In this textbook question:

The answers state "Note that there is no voltage drop across the voltmeter". However, there is a current across the wire. This goes against what I have been taught - that a flow of charge can only occur in areas of varying voltage.

Also considering this question:

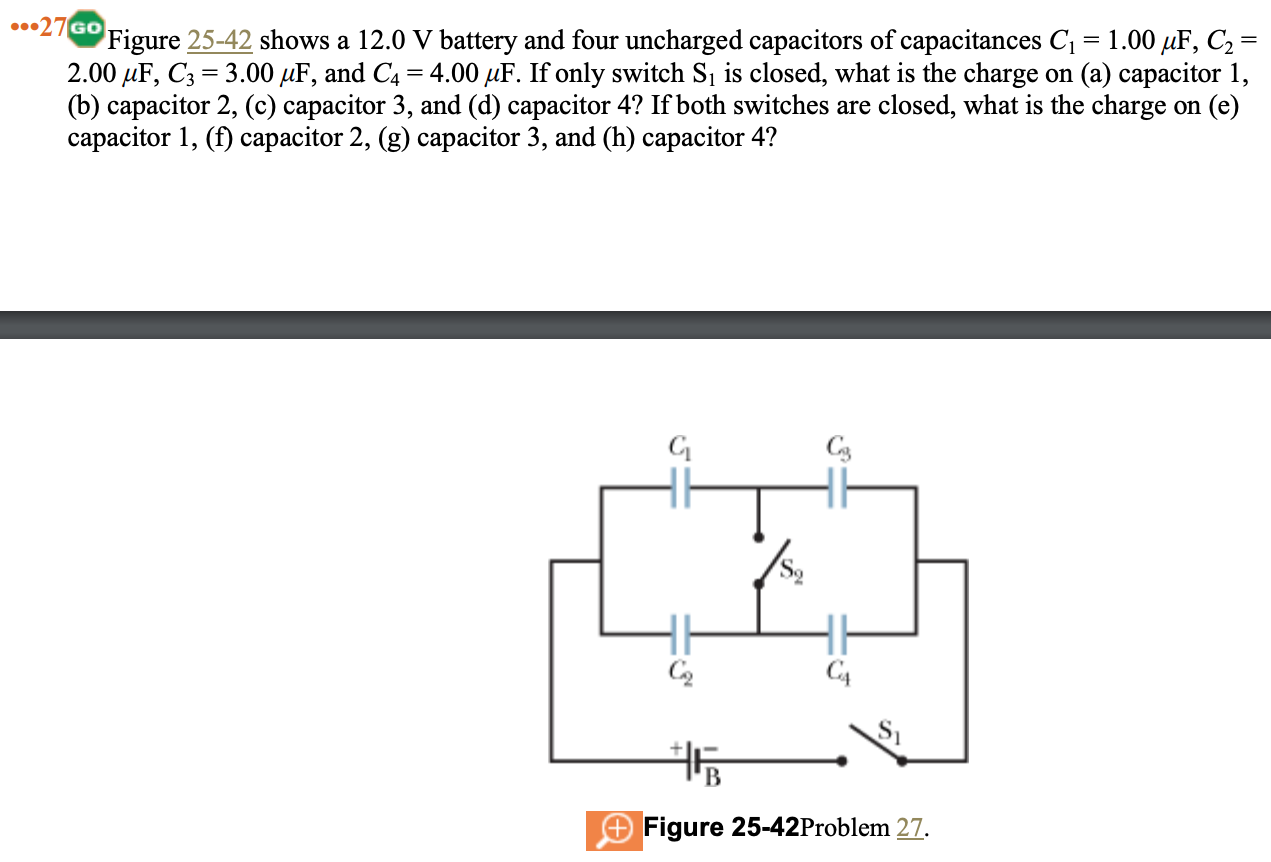
For parts after e), there is also no voltage drop. I suspect that this is due to the nature of capacitors to store charge, thus evening out the voltage, but I am still unsure.

In the latter case, however, the system could be solved by considering two parallel sets of capacitors in series, which allows the flow of electrons. However, in the first, this can only be done to work out the equivalent resistance, but not the final solution (instead requiring the loop rule). Why is this the case?

The rules of whether charge flows seem quite arbitrary and confusing, as in a Wheatstone bridge, there is no current in between, but nor a potential difference. Thus, a clarification would be greatly appreciated.

Answer 1

That's an ammeter, not a voltmeter, and "there's no voltage drop" just suggests the ideal-ammeter characteristic of zero resistance, as the correct approximation to apply.

There can, of course, be current flow in regions of no voltage drop (charged droplets of oil drop due to gravity in a Millikan experiment apparatus, for instance). This case, however, just treats the ammeter as being much lower resistance than the resistors.

The switched-capacitor problem is slightly complicated because there is no reason to believe the initial condition does not include charge on the (isolated) regions connected to S2: unless one arbitrarily calls those zero charge as a starting condition, there's a Kirchoff equation missing for a complete solution.

Answer 2

Just because it looks like a Wheatstone bridge arrangement do not treat it as a Wheatstone bridge.

This goes against what I have been taught - that a flow of charge can only occur in areas of varying voltage.
I would guess that all the circuits that you have drawn so far have symbols for components connected with "black" lines.
Those black lines represent a conductor between components which has no resistance, $R$, and the potential difference across the conductor, $V$, is assumed to be zero.
Thus you have $\underbrace{V}_{=0}=I \times\underbrace{R}_{=0}$ allowing the current $I$ to have any value constrained by the rest of the circuit.

---

If switch $S_1$ is closed, capacitors $C_1$ and $C_3$ are in series and capacitors $C_2$ and $C_4$ are in series with the series combinations being in parallel with each other.
If both switches are closed then capacitors $C_3$ and $C_4$ are in parallel and capacitors $C_1$ and $C_2$ are in parallel with the parallel combinations being in series with one another.
When two capacitors are in series the total charge stored on the plates which are joined always stays as zero as they are electrically isolated from their surroundings and so the charge stored on each capacitor must be the same.
When two capacitors are in parallel the voltage across each of them must be the same.