If we apply some torque to spinning wheel in a vacuumed space in body frame y axis, wheel try to precess due to gyroscopic effect but it will be prevented by obstacles as seen from picture,
Before going into the specifics of this setup I need to point out the following: the gyroscopic effect occurs in response to motion.
Of course: that looks like useless nitpicking; if there is an unopposed torque then motion will occur anyway.
The reason for emphasizing this distinction: it is not the case that a gyro wheel starts precessing motion instead of yielding to the torque. In order for the precessing motion to start going the gyro wheel must yield a little to the exerted torque.
This must-yield-a-little was experimentally confirmed with a table-top experiment, by Svilen Kostov and Daniel Hammer (2010). Article available on Arxiv "It has to go down a little, in order to go around"
The mechanics of the gyroscopic effect is described in a 2012 answer by me: Gyroscopic effect
For the following I will assume that you have absorbed the description in that 2012 answer.
I will assume a specific friction is available to provide quick damping of any nutation. (In classroom demonstrations it is customary to suppress nutation. Most demonstrators are unaware that they are actually applying an intervention; suppression of nutation. Nutation is an essential aspect the dynamics.)
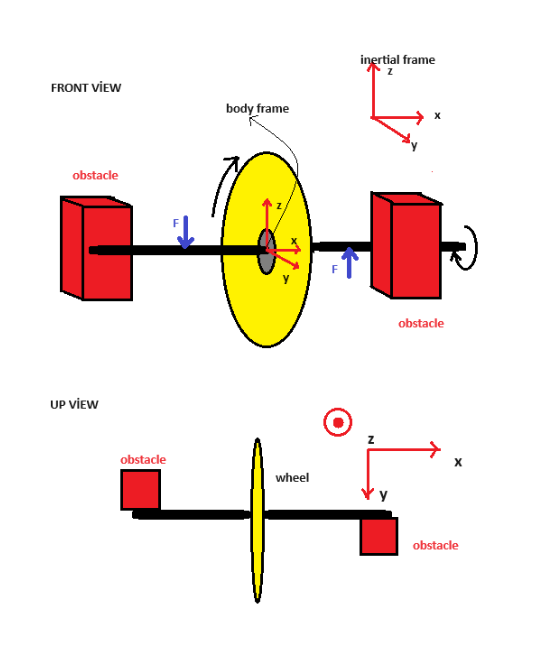
For the setup in the images you provided:
Without the obstacles the wheel would, after yielding to the torqe a little, start moving in precessing motion. That precessing motion gives rise to a tendency to rotate in the direction opposite to the exerted torque. That opposition acts against the tendency of the exerted torque.
For the setup in the images: the obstacles prevent onset of precessing motion. Without that precessing motion there is no opposition to the exerted torque. As a consequence the wheel will just keep yielding to that torque.
The motion of yielding to the torque causes the axle to push against the obstacles. Since this is an idealized thought demonstration we assume that that contact is frictionless. If the contact is frictionless then the push against the obstacles is without effect
The reaction torque of a gyroscopic flywheel is always such that it will align the rotation of the flywheel with the input torque rotation. This makes it easy to predict the behaviour of a gyroscope. Once the flywheel rotation is aligned with the input torque the precession stops.
what will be reaction of the wheel?
If the wheel is not allowed to precess the wheel will no longer react and if the wheel axis is supported only at the right hand end the wheel will fall towards the ground like a normal weight. The behaviour of a flywheel depends on it being able to freely precess.
Start with no spinning mass and think of a tilting plane as a seesaw, not mounted to the ground, but when you tilt it, it has a tilt axis running through the center that is the fulcrum for the seesaw. A tilting plane is an absolute condition, and it has only one absolute axis defining this tilting condition. Tilting has a direction, and the tilt axis follows any new tilt direction like an axle of a cart. The axle is always perpendicular to the direction of the cart. Now add mass spinning within this tilting plane. The plane does not spin, and the seesaw tilting ends and tilt axis are the same as they were with no mass spinning inside.
The thing about a seesaw is that one end goes up while the other goes down -opposing end velocities. NOW, given that mass is spinning inside this tilting spin plane, it means that the mass must TOTALLY reverse tilting end velocities each time it passes between tilting seesaw ends. That is a massive amount of acceleration focused at the tilt axis running through the spin plane, AND it's perfectly perpendicular to the spin plane and spin velocity (will not affect spin). This acceleration normal to the spin plane begins pushing the spinning disk to precess, but in order to precess, this is a change in tilt direction which takes the axle of the cart with it producing perpendicular acceleration the whole time, so the axle ends up aligning itself with the force that caused all of this in the first place. If you stop precession, the acceleration production just disappears, because there is no tilting end velocity going through the tilt axis.
This paper describes the acceleration produced by a tilt axis, but not the directional aspect:
Paul Rood, "'Action Equals Reaction'—Even in Gyroscopes," Am. J. Phys. 13, 175-177 (1945).
My video is an engineering simulator gyro showing the causal components. My math technique is based on the path that an atom in a gyro follows in space. Take the second derivative of this path and isolate the normal components of force. This is actually a force field shaped like a cylindrical hoof. Most people cannot see this, because "cause" is not intuitive.
Proof! Angular Momentum Is Not Causal
