0
$\begingroup$

1

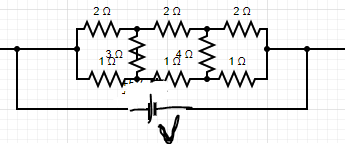
If we calculate the potential at both ends of these $3\,\Omega$ and $4\,\Omega$ resistors, that comes out to be the same. It is $V/3$ at both ends of the $3\,\Omega$ resistor and $2V/3$ at both ends of the $4\,\Omega$ resistor. So, we can safely say that because there is no potential difference, no current will be flowing through $3\,\Omega$ and $4\,\Omega$ resistors, so they can be removed from the circuit.

My question is simple and straight: Can we assume the above circuit consists of two balanced Wheatstone bridges, and straightaway remove the $3\,\Omega$ and $4\,\Omega$ resistors, without actually calculating the potentials at the ends? Because the ratio of resistances is the same (the required condition for a balanced Wheatstone bridge).

When I asked the same question to my physics teacher he said that the answer is coming by chance and we cannot consider it to consist of two balanced Wheatstone bridges. However, I am not convinced much so I decided to verify from Stack Exchange.

Improve this question
$\endgroup$
2
  • $\begingroup$ I may lack of imagination, but I can't see two Wheatstone bridges there $\endgroup$
    – basics
    Commented May 29 at 17:47
  • $\begingroup$ @basics First bridge. Since the voltage across the $4\Omega$ resistor is zero, you can replace the $4\Omega$ resistor with a wire. Then the part of the circuit consisting of the left-hand top-2 $2\Omega$ resistors and bottom-2 $1\Omega$ resistors, plus the left hand wire, plus the $4\Omega$ resistor (which we now think of as a wire), plus the $3\Omega$ resistor -- which has zero voltage across it -- form a Wheatstone bridge. Second bridge. Very similar, but use the right hand part of the circuit, and now the $3\Omega$ resistor acts like a wire and the $4\Omega$ resistor is the bridge. $\endgroup$
    – Andrew
    Commented May 29 at 18:36

1 Answer 1

Reset to default
0
$\begingroup$

You've done all that is needed in your first paragraph – essentially you have treated the top and bottom rows of resistors as potential dividers.

In my opinion the Wheatstone bridge approach adds nothing, but you could regard the five left hand resistors as forming a Wheatstone bridge, as both ends of the 4 ohm are at the same potential and might just as well be connected together at a potential of –(2/3)V with respect to the left hand end of the resistor network. Similarly you could regard the five right hand resistors as forming a Wheatstone bridge – if you really feel the urge to do so! You will, at the same time, have shown that both bridges are balanced.

Improve this answer
$\endgroup$
3
  • $\begingroup$ You said - ... as both ends of the 4 ohm are at the same potential.. , we know that they are at same potential after doing page long calculation and solving a system of four equations in four variables. My question is can we just straightaway remove those 3 and 4 ohm resistors, without actually calculating the potential at junctions? $\endgroup$
    – Physics_enthus
    Commented May 30 at 4:17
  • $\begingroup$ Can we say that the equivalent resistance of the given circuit is independent of the values of 3 and 4 ohm resistors? $\endgroup$
    – Physics_enthus
    Commented May 30 at 4:31
  • $\begingroup$ Yes. If the 4 ohm resistor wasn't there (between points C and D, let's say), we know from potential divider theory applied to the top three resistors and to the bottom three resistors that the pd between C and D would be zero. Therefore there'll be no current through any conductor that we connect between C and D. So this 'bridging' conductor (resistor!) might as well not be there. This is exactly how we justify the balance condition for a Wheatstone bridge – in which, originally, the bridging component was a (low resistance) galvanometer. There is no need for a Kirchhoff's laws treatment. $\endgroup$
    – Philip Wood
    Commented Jun 1 at 10:30

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.