Wheatstone bridge and potential difference

Question

above we have a balanced Wheatstone bridge and we know that the potentials at $b$ and $d$ are equal, hence current does no flow through $bd$. However, in a conducting wire the potential difference between two points is zero but still currently flows through the wire.....What's different in this case?Also if I remove the resistor in wire BD and replace it with a conducting wire what will happen now?

will it short as $bd$ is the least resistive path?

[I'm a high school student and my knowledge about circuits is quite less, all answers are welcomed]

w

Answer 1

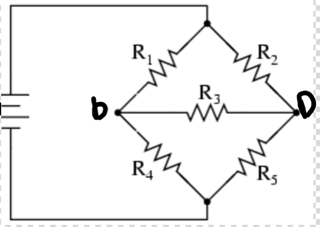
Replacing R$_3$ with a wire is just replacing in with a very small resistor. Wires do have some resistance, just much smaller than a normal resistor. For a balanced bridge, V$_{BD} = 0$. So

$$I = V_{BD}/R_{wire} = 0$$

If you were to consider the wires from the battery to the Wheatstone Bridge as very small resistors, R$_0$ and R$_6$, you would find the total resistance is

$$R = R_0 + R_{bridge} + R_6 \approx R_{bridge}$$

So the total current would be approximately unchanged

$$I = V/R \approx V/R_{bridge}$$

The voltage drop across R$_0$ and R$_6$ would be approximately $0$, but not exactly $0$.

$$V_0 = I R_0 \approx 0$$

---

It might help to think of an analogous set of pipes with water flowing through them.

Voltage is like water pressure. Water pressure pushes water through pipes. A voltage difference pushes electrons through wires.

An electric current is like a water current. A water current is the amount of flowing water per second. An electric current is the amount of flowing electrons per second.

A resistor is like friction in a pipe. A narrow pipe has more friction. A resistor impedes the flow of electrons.

In a balanced bridge, there is no voltage difference between B and D. Nothing pushes electrons to flow, even if there is a low resistance. Just like if the water pressure was equal, there would be nothing pushing water to flow.

The situation is different for R$_0$ and R$_6$. The battery is like a source of high pressure water. Water flows through big fat pipes to and from the bridge. There is very little to impede the flow, so there is very little pressure difference between the ends of those pipes. Lots of water flows. The amount depends on how restrictive the pipes in the bridge are.

Answer 2

Thanks to satan 29 and John Rennie for a better understanding of the provided question.

My explanation is as follows. Consider the following circuit:

Let us assume that the resistance of the wire is $0$ (hypothetical situation) but not of battery. In that case the current flows throughout the wire with constant speed.

Reason? It's because, just when we closed the circuit the electric field was created and charge starts to arrange itself so that the $\textbf{E}$ inside conductor is $0$ with an acceleration of $e\textbf{E}/m$. But after some very very small time, the Electric field goes to $0$ inside the conductor and this time charge would not accelerate and it would move with constant speed. When it enters the battery it gains eV of energy, but this is of no use since it is lost due to the resistance of the battery. So we can say that charge travels uniformly despite the fact that $\mathrm dV = 0$ between red and yellow or green and yellow.

In case $R$ is not equal to $0$ the situation is the same, but this time the charge comes to rest due to collision with atoms (resistance). Due to this the $\bf E$ inside the conductor gets disturbed and it accelerates again to create $\bf E$ such that $\bf E$ inside conductor is 0. This happens again and again such that the charge seems to be moving with constant velocity (drift velocity).

Now about the Wheatstone bridge:

In the above circuit, the battery was connected and the charge started to move as explained above, due to non-zero wire resistance. The potential difference between the point $B$ and $D$ is $0$, so the charge does not move to $R_3$, even if it is a $0$ resistance path. But as we saw earlier, charge does move with constant speed when $\mathrm dV = 0$ and $R = 0$.

Why does that not happen here?

First, remember that the current does not flow through $R_3$ even if we put $0$ resistance in place of $R_3$. The reason is as follows:

When you read the first example, the answer is hidden in that $\mathrm dV$ was $0$ in it $(R = 0)$. When, not in the beginning when battery was just connected, but instead after some very small time, it got accelerated and it happened because charge started to move when $\textbf{E}$ was applied. In the process, $\mathbf{E}$ inside conductor became $0$ and then the charge never accelerated. Similarly in this question the $\mathrm dV$ between $B$ and $D$ is $0$ from the beginning. This is because of the arrangement of resistors required for the Wheatstone bridge -- if electrons in the bridge wire were at rest and after connection of battery due to absence of electric field in that region, how would it accelerate? That is why, despite the resistance of the bridge wire, no charge flows through that wire as there is no potential difference and hence no acceleration or no change in velocity.