In order to workout the method for establishing the formula of thin lens, my teacher says that the optical path is:
$PA + AQ = PS_1 + nS_1S_2 + S_2Q$ ($n$ is the refractive index of the lens)
Why is she multiplying $S_1S_2$ by $n$?
Also, she says that for spherical surface $S_1$, with centre of curvature $C_1$, we can write from geometry,
$h^2 = 2(S_1C_1 - OS_1) OS_1 $
How is she deriving this?
1)
The optical path length for light travelling in a medium is defined as the path length that will produce that same phase difference(or rather contains same number of wavelengths) as light would when travelling in vacuum.
Suppose the medium has a length(thickness) $t$.
A slab of the medium of thickness $t$ contains $\frac{t}{(\frac{\lambda}{\mu})}$ wavelengths which is equivalent to a slab of vacuum of length $\mu t$ which will contain $\frac{\mu t}{\lambda}=\frac{t}{(\frac{\lambda}{\mu})}$ wavelengths.
Therefore in order to find the optical path length in a medium we have to multiply by the refractive index.
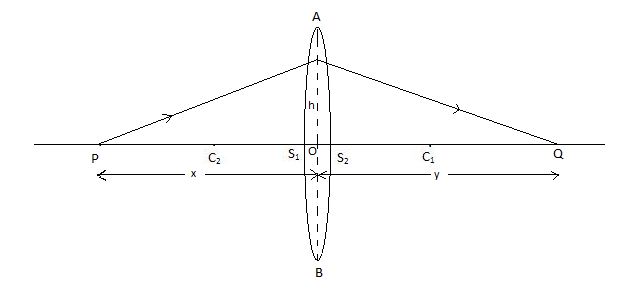
2)Consider the following notation:
$x=OS_1$
$y=OC_1$
$h=OA$
$R=$radius of curvature of the first surface$=C_1S_1=C_1A$
Consider the Pythagoras' theorem in $\triangle AOC_1$,
$R^2=h^2+y^2$
Also we have $C_1S_1=R=C_1O+OS_1=x+y$
Using this in the above equation we get,
$x^2+2xy+y^2=y^2+h^2$
$2xy+x^2=h^2$
Since the lens is thin,$x<<y$ and so we can safely neglect the $x^2$ term,
$h^2=2xy$ which is exactly what we had to prove.
