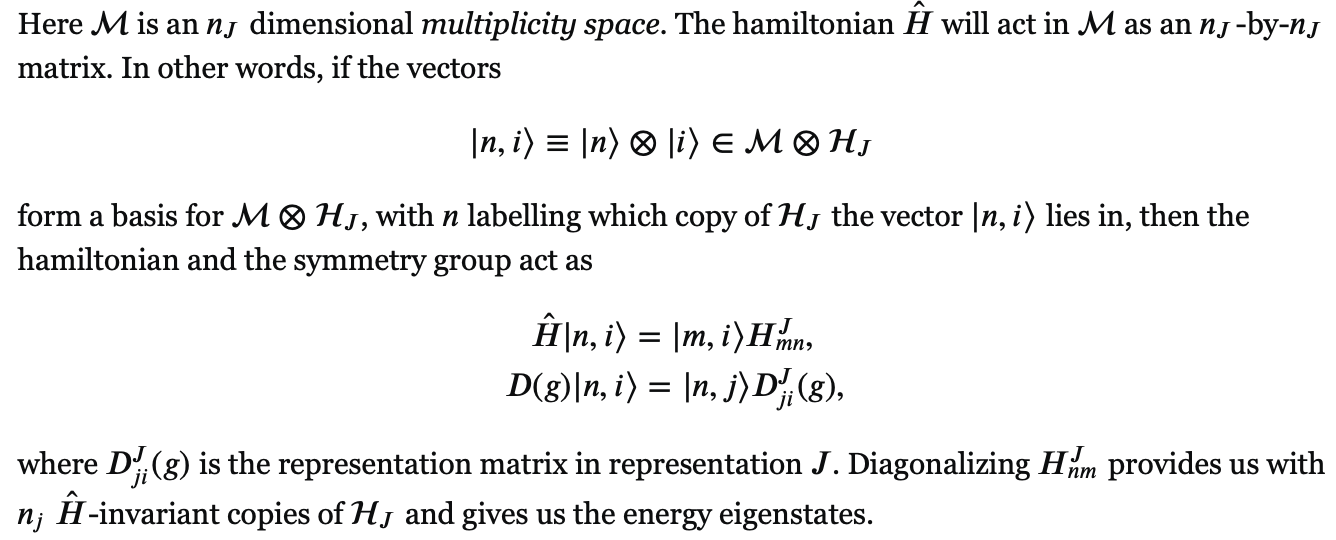My question in the title stems from not completely understanding the last three lines of this answer. I list specific questions at the end of this post.
Setup. Consider a quantum system described over a Hilbert space $\mathcal{H}$ and a symmetry group $G$ of the system. Let $D$ be a representation of $G$ carried by $\mathcal{H}$. Suppose that $D$ is decomposable into a number of irreps where the multiplicity of certain irreps is greater than 1. Let ${H}$ be the Hamiltonian describing the system. Suppose $$ D(g) {H} = {H} D(g), \forall g \in G.$$ $H$ is then an intertwining map for $D$ with itself so by Schur's lemma, $H$'s action on each irrep is proportional to identity.
Multiplicity and Hamiltonian action. Suppose an irrep $J$ occurs $n_J$ times in the decomposition of $\mathcal{H}$. Let $\mathcal{H}_J$ be the subspace associated with $J$. We introduce the $n_J$-dimensional ``multiplicity space'' $\mathcal{M}$ via $$\mathcal{H}_J\oplus \mathcal{H}_J\oplus\cdots \mathcal{H}_J=\mathcal{M}\otimes \mathcal{H}_J $$ where there are $n_J$ copies of $\mathcal{H}_J$.
Here's where I get confused in this answer I linked to above (screenshot because mathjax in the copied text isn't working...):
Specific questions:
What exactly is $H_{mn}^J$? Is it related to a matrix representation of $\hat{H}$ in terms of the basis to $|n, i\rangle \in \mathcal{M}\otimes \mathcal{H}_J$?
What exactly is $D_{ji}^J(g)$?
How does one prove the claims that mike stone makes leading to the last two equations in the answer: $$\hat{H} |n,i\rangle = |m,i\rangle H_{mn}^J, \\ D(g)|n,i\rangle = |n,j\rangle D_{ji}^J(g)? $$ Is the proof related to the argument at the top of page 7 of Noah Miller's notes showing that if $[H, (\pi \oplus \pi)(g)]$ for all $g\in G$,then $H$ must have the following form $$ H = \begin{pmatrix} A \mathbb{I} & B\mathbb{I} \\ C\mathbb{I} & D\mathbb{I} \end{pmatrix}, $$ where $A, B, C$ and $D$ are scalars?