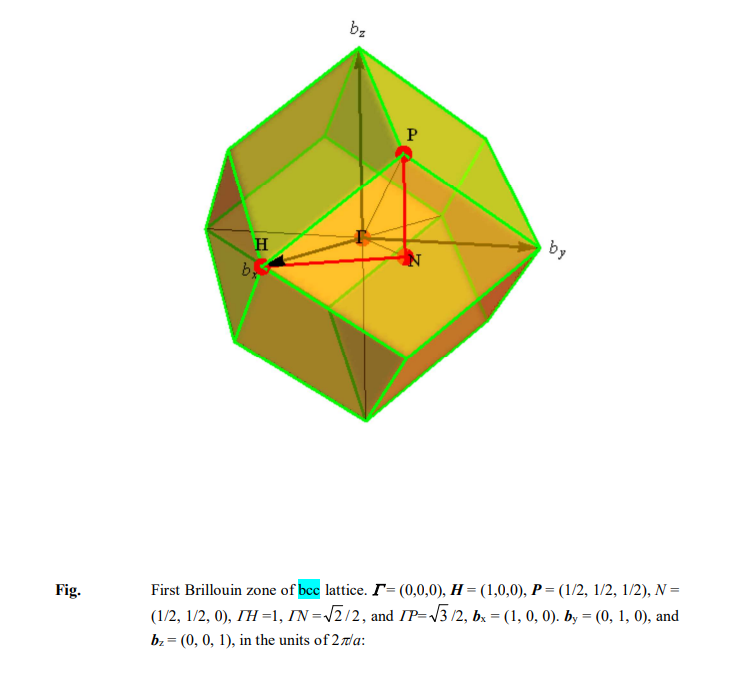
According to many online sources that the first Brillouin zone of a body center cubic (bcc) has the shape illustrated below. Along the $k_x$ (or $k_y$, $k_z$, $(100)$...) direction, basically the $\Gamma H$ direction, the zone edge is at $2\pi/a$. However, why is not H point at ($\pi/a$)? Isn't the Wigner-Seitz cell supposed to be bisecting the reciprocal lattice vectors which has length $2\pi/a$?
$\begingroup$
$\endgroup$
2
-
1$\begingroup$ I assume that $a$ is the length of the side of the standard cubic cell? If so, then note that the primitive unit cell of the bcc lattice is significantly smaller than the standard cubic cell in which it sits, making the points in reciprocal space farther apart for bcc compared to the reciprocal lattice of the sc lattice. This likely explains why the points at the edges of the bcc BZ boundary seem like they're too far apart. I haven't sat down and computed the exact values yet though. $\endgroup$– marchCommented Apr 1 at 18:23
-
$\begingroup$ The "conventional" cubic unit cell with an atom in the center contains 2 atoms (the one in the center and 1/8th of each of the 8 corners). That is not a primitive unit cell which for bcc holds only 1 atom. $\endgroup$– Jon CusterCommented Apr 1 at 18:39
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
0
The BZ of the BCC lattice has a RLV of $4\pi/a$.
Recognize that the primitive vectors for the BCC lattice aren't to the corners of the conventional unit cell. They are the three that go to the nearer body center atoms. With those, you can work out the RLV of $4\pi/a$.
