A question related to Newton's Rings (SOLVED)

Question

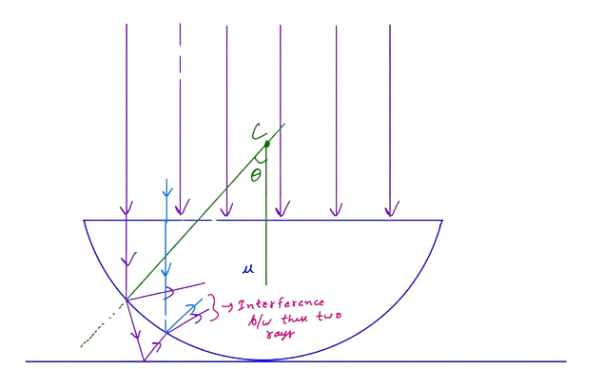
I was solving this question,

I arrived at the correct answer which is 'A' the reason 'C' and 'D' are incorrect is cuz the fringes would eventually converge as if we look at this question from another point of view it's similar to a light source striking a plano-convex lens (But the lens is cut out from a cylinder).

As an exercise I tried to find out the distance between the fringes produced by relating it with some variable that's changing with every fringe, which in my case was θ.

The text in pink says 'Interference between these 2 rays'.

I also found an article on Newton's Rings but I failed to understand how they arrived at the formula

$$r_N=\left[\lambda R\left(N-\frac{1}{2}\right)\right]^{1/2}$$

Could someone please help me out here, thank you!

EDIT:-

The solution has been linked in the comments to this post please check it out.

Answer 1

The Newton's rings formula arises from a condition on the optical path length difference $\delta$ between the ray reflected on the interface lens\air and the ray reflected by the glass plate and refracted at the interface air\lens.

This condition is the condition of interference. It is given by $N\lambda=\delta$ or $(N+1/2)\lambda=\delta$ if you consider bright or darks fringes ($N$ is an integer). One can show that $\delta=2e$ where $e$ is the vertical height between the glass plate surface and the incidence point of the ray on the convex surface of the lens. You can write $e$ as a function of the curvature radius of the lens $R$ and the horizontal distance $r$ between the incident point on the convex lens and the axis of the lens.

I'm pretty sure you can you find a derivation online ;)