Quantum position in molecular vibration

Question

Evere molecule consists of atoms that vibrate around their equilibrium positions. This can be viewed from a classical or a quantum perspective. However, I found a seeming inconsistency between these two approaches: in the first approach the particle is moving, but in the second approach (the expected value of) its position is constant in time.

In the classical approach, let $Q(t)$ be the deviation of an atom around its equilibrium position. Using Hooke's law one can derive the formula

$$Q(t)=\cos(2\pi\nu t)\tag{*}$$ for some constant $\nu$. This means that the position of the particle varies with time.

In the quantum approach, you can approximate the atom by a quantum harmonic oscillator. The QHO has Hamiltonian

$$\hat{H}= \frac{1}{2m}\hat{p}^2+\frac12\omega^2\hat{x}^2$$

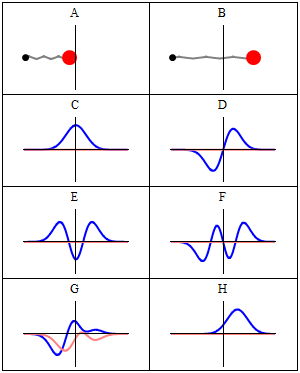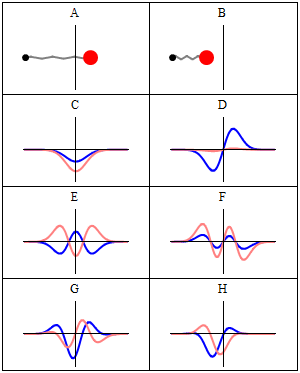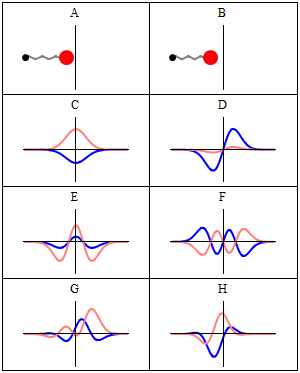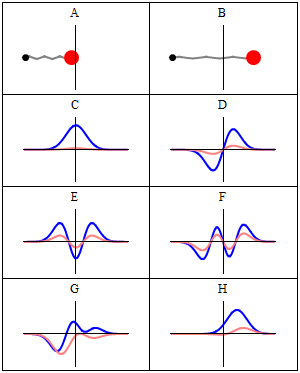
We can not speak about the 'exact position' because that does not exist in quantum mechanics; however, the wavefunction may be sharply peaked around a central position. Therefore the quantum analogue for position is $\langle \hat{x}(t)\rangle=\int_{space}x(t)|\psi(x,t)|^2$. For the QHO you can derive that $\frac{d}{dt}\langle \hat{x}(t)\rangle = 0$ for all eigenstates. That means that the (expected value of the) position of the particle does not change in time. The expected position is 'standing still'.

In certain situations, the quantum analogue reduces to the classical version. For example, Ehrenfest's theorem implies that $\frac{d}{d t}\langle x\rangle=\frac{1}{m}\langle p\rangle$. Replacing $\langle x\rangle$ by the 'classical' position and $\langle p\rangle$ by the 'classical' momentum, we obtain the usual relation. I would have expected that for molecular vibrations, you can replace $\langle \hat{x}(t)\rangle $ by $Q(t)$ to retrieve Equation (*). Why does this not work? Does this mean that the classical approach gives an 'incorrect' formula for $Q(t)$ and only the quantum approach is correct?

Answer 1

Your claim "for the QHO you can derive that $\langle \hat{x}(t)\rangle =0$ for all $t$" applies only to expectation values of energy eigenstates $|n\rangle$, but not for the general case of an arbitrary state vector $$|\psi\rangle = \sum\limits_{n=0}^\infty c_n |n\rangle, \qquad \sum\limits_{n=0}^\infty |c_n|^2 =1.$$ A well known case, where the expectation value of the position operator $$ \hat{x}(t) = e^{i \hat{H} t/\hbar} \hat{x}(0) e^{-i \hat{H} t/\hbar}= \hat{x}(0) \cos \omega t +\frac{\hat{p}(0)}{m \omega} \sin \omega t $$ of the harmonic oscillator performs a "classical" oscillation is the coherent state $$ |z\rangle= e^{-|z|^2/2} e^{z a^\dagger} |0\rangle = \sum\limits_{n=0}^\infty\frac{e^{-|z|^2/2} z^n}{\sqrt{n!}} |n\rangle, \qquad z \in \mathbb{C}. $$

Answer 2

You are right. In an energy eigenstate you have a stationary wavefunction like this: $$\Psi(x,t) = \psi_n(x)e^{-iE_nt/\hbar}$$ Look at examples C, D, E, F in the animation below, which are such states (for $n=0,1,2,3$). The expectation value of position for these stationary states is constant: $\langle \hat{x}(t)\rangle = 0$.

_{(animation from Wikipedia - Quantum harmonic oscillator)}

You get "moving" states when you superpose more than one energy eigenstates. Example G and H of the animation above are such states. G is a superposition of two eigenstates (with $n=2$ and $n=3$) $$\Psi_G(x,t) = \psi_2(x)e^{-iE_2t/\hbar} + \psi_3(x)e^{-iE_3t/\hbar}$$ and H is a superposition of infinitely many eigenstates ($n=0,1,2,3,...\infty$) $$\Psi_H(x,t) = \sum_{n=0}^\infty c_n\psi_n(x)e^{-iE_nt/\hbar}$$ In these states the expectation value of $\langle \hat{x}(t)\rangle$ actually varies with time $t$.