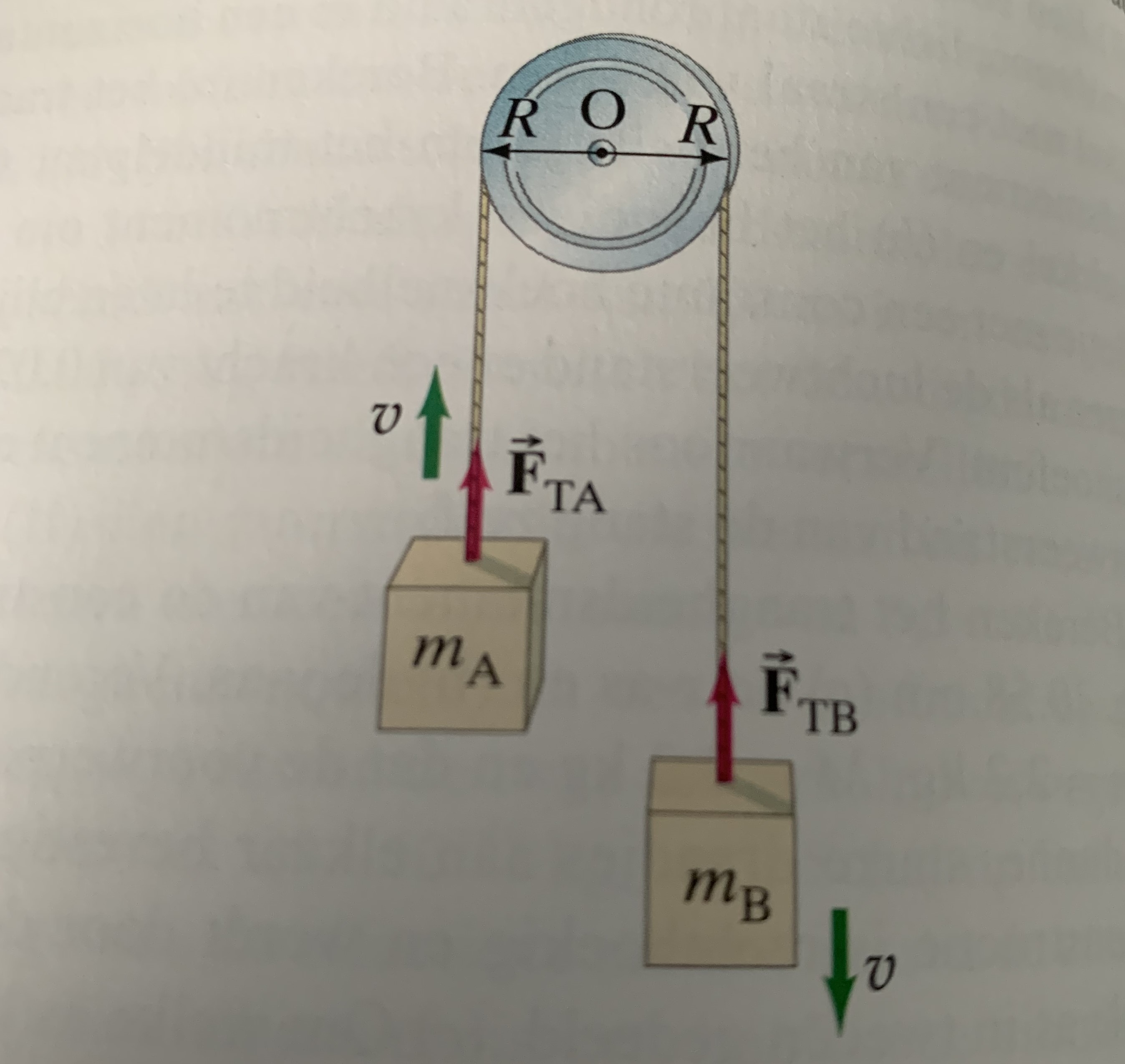
I encountered this problem while working on this exercise. The question is to find an expression for the acceleration of the two blocks. I started with writing newtons second law for the two blocks and newtons second law involving torque which is related to the angular acceleration of the pulley. My question is: is the tangential acceleration equal to the acceleration of the blocks? If so, why is this the case?
Is the tangential acceleration equal to the acceleration of the blocks?
As long as the rope does not stetch, and there is sufficient friction for the rope not to slip on the pulley, then we can take the angular displacement of the pulley $\theta$, multiply it by the radius of the pulley $R$ and get the amount by which the rope has moved. But this must also be the displacement of the blocks, $x$. So we have
$x = R \theta$
If we differentiate this once with respect to time we have
$v = R \omega$
where $\omega$ is the angular velocity of the pulley. If we differentiate again we have
$a = R \dot \omega$
where $a$ is the acceleration of the blocks and $\dot \omega$ is the angular acceleration of the pulley.
We consider that the linear acceleration of the two blocks is equal if the string is not stretchable. This means (if we imagine two blocks connected by a string on one straight line) that the distance between them is constant, so the change of position for them will be the same, which in turn means that both linear velocity and linear acceleration will be equal.
is the tangential acceleration equal to the acceleration of the blocks? If so, why is this the case?
Just posting the answers found in the comments. If there is no slipping between the rope and the pulley, then the tangential velocity (and hence the tangential acceleration as well) of the pulley must be equal to that of the rope. Since the blocks don't move relative to the rope, they have the same magnitude of acceleration as the tangential acceleration of the pulley.
