Why is it wrong to find centripetal acceleration using change of velocity over change of time?

Question

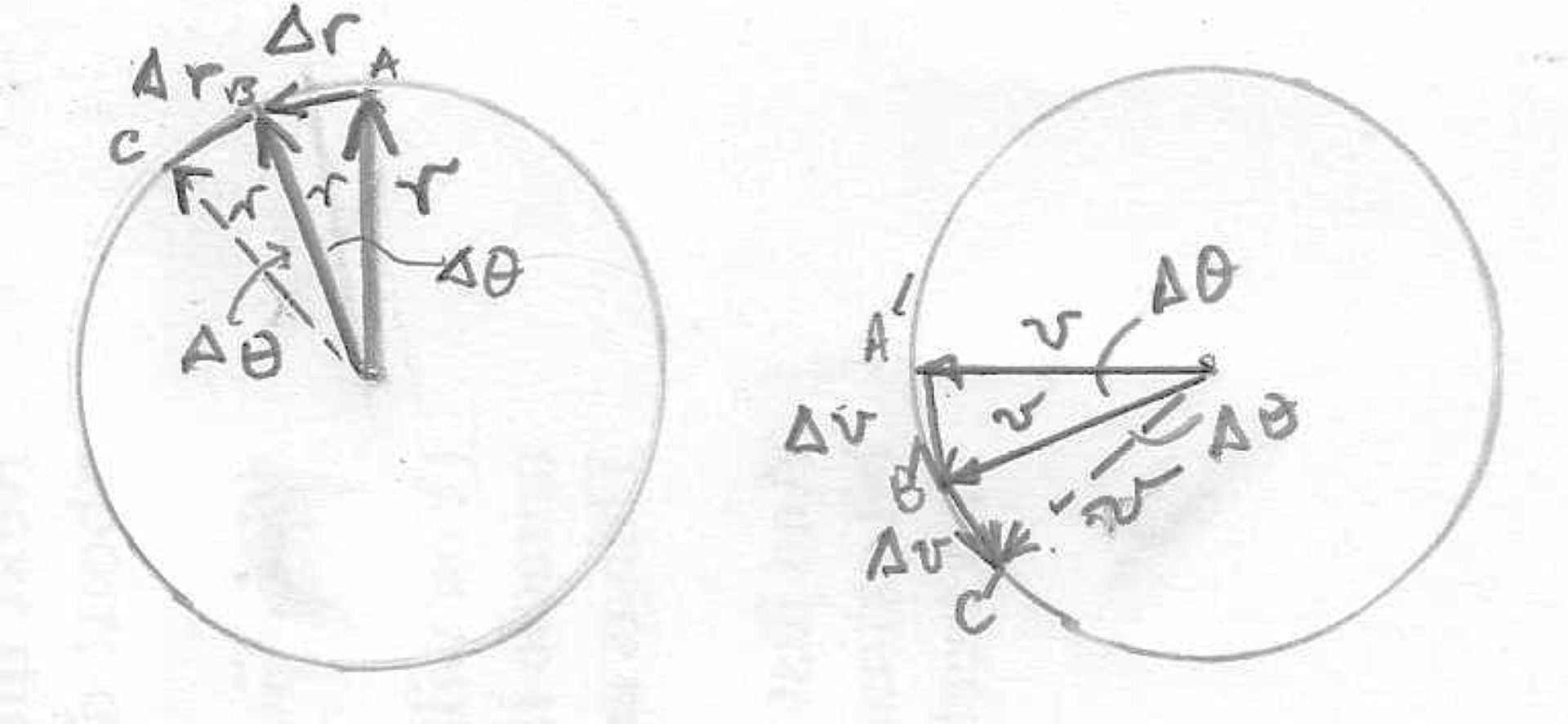
This question asks to find the centripetal acceleration by giving the initial and final velocity over the change of time.

As shown, my book combined two rules to find the acceleration. I utterly understand how my book solved it and why it solved it that way. What I don't understand, however, is why the usage of the formula below doesn't lead to the same answer or even close.

$a= \frac{v_f - v_i}{ ∆ t}$

$v = 640.31 (calculated), t = 24 (given)$

$a= \frac{(640.31--640.31)m/s}{ 24 s} = 53.4 m/s^2$

Judging by the two different answers, my approach and way of thinking must be wrong. Which is surprising since this formula $a= \frac{v^2}{r}$ is derived from $a= \frac{∆v}{∆t}$.

Answer 1

Your error is the use that you made of the equation $a= \frac{v_f - v_i}{ ∆ t}$.

Consider an object moving along a circular path of radius $r$ at a constant speed $v$.

In some time $\Delta T$ the object moves from position $A$ to position $B$ and then on to position $C$ in the same time.

the change in position $\Delta r \approx r\,\Delta \theta$ and $\Delta T \approx \dfrac{\Delta r}{v}$ with the approximation getting better and better as $\Delta \theta \to 0$.
If one goes around $2\pi$ radians and take the limit $T = \frac{2\pi r}{v}$.

Now do the same thing for the velocity vector in time intervals of $\Delta T$ shown by $A',\,B',\,C'$, etc. The change in velocity in time $\Delta T$, $\Delta v \approx v\Delta \theta $ again with the approximation betting better as $\Delta \to 0$.

This is the place you made the error.

You said that the change in velocity in going from position $A$ to a position where $\theta$ has changed by $\pi$ is $v-(-v)=2v$ but in fact it is $\pi v$ because you need to add up all those incremental changes in velocity which in the limit are on a circle of radius $v$.
If you are not sure about this look back to the cumulative change in position and consider whether you would evaluate is as $r-(-r)=2r$?

To finish off in the limit $\Delta \theta \to 0$ in a time $T$ the cumulative change in velocity is $2\pi v$ and so the acceleration $a=\dfrac{2\pi v}{\left (\frac{2\pi r}{v}\right)} = \dfrac {v^2}{r}$.

Answer 2

What you have calculated is the average acceleration, or at least its magnitude. This is different than the centripetal acceleration which is the instantaneous acceleration towards the centre. The average acceleration is not useful in this context of pilots trying not to lose consciousness. For an extreme example consider that the average acceleration for a pilot completing any complete loop would be zero regardless of the speed or radius of curvature. This is a bit like considering dropping something, say an egg, onto the floor. The initial and final velocities might be zero leading to a zero average acceleration but the egg still ends up broken because for some time interval the acceleration is large.

Answer 3

First thing to is rotate the coordinate system so the velocity is (SI units implied):

$$ v_{x'}(t_1=0) = \sqrt{410000} \equiv v_0 $$

$$ v_{x'}(t_2=24) = -\sqrt{410000} = -v_0$$

The question is, in $(v_{x'}, v_{y'})$ space, how long is the path traced out by the velocity? Its $\pi v_0$, so:

$$ a = \frac{\pi v_0}{t_2-t_1} = 83.81 $$

Appendix: The point here, is we don't care about the radius of the turn, we don't care about configuration (position) space. In velocity space, you have:

$$ \vec v(t) = v_0 \big( \cos\frac{2\pi t}T \hat v_{x'} + \sin\frac{2\pi t} T \hat v_{y'}\big)$$

with $T=2 \times 24\, s$. You don't want to write the math down, because you can imagine a semi-circular arc in your head and solve the problem fast.

In polar coordinates, which is more useful, that's:

$$ v_r(t) = v_0 $$ $$ v_{\theta} = v_0 \times \frac{2\pi t}{48} $$ so:

$$ \dot v_r = 0$$ $$ \dot v_{\theta} = \frac{2\pi}{48}v_0 = 83.....$$

No need for position coordinates.

Addendum: I would protest this problem for using the wrong two parts of a pythagorean triple. $\sqrt{41}$...just, no.