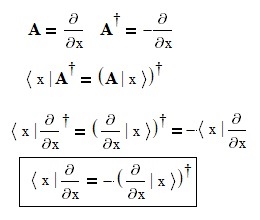Is the derivative of the adjoint the adjoint of the derivative? [closed]

Question

Does $\frac{d}{dx}\langle u|= (\frac{d}{dx}|u\rangle)^\dagger$? Here $|u\rangle$ is any vector in a Hilbert space and $\dagger$ is the adjoint/conjugate-transpose.

It seems to me that this should not be the case since $(\frac{d}{dx}|u\rangle)^\dagger = \langle u| (\frac{d}{dx})^\dagger = - \frac{d}{dx} \langle u|$. Here we used that $(\frac{d}{dx})^\dagger=-\frac{d}{dx}$ since the momentum operator $p=-i \frac{d}{dx}$ is Hermitian so $p^\dagger = p$ and $p^\dagger = i (\frac{d}{dx})^\dagger$.

Related to Is the time derivative of the adjoint equal to the adjoint of the time derivative? , but possibly with a different conclusion for the space derivative and time derivative.

Does my logic make sense for why the derivative of the adjoint is not the adjoint of the derivative, or where did I make a mistake? Thanks!

Answer 1

The derivative of a bra function is indeed the negative of the adjoint of the corresponding ket acted on by the "derivative operator". This statement is kind of confusing because maybe its not clear that there are 2 different derivative operators at play here (also I worded it badly on purpose lol).

To make things precise, we need to distinguish the derivative of a function, $\frac{\mathrm d}{\mathrm dx}$, and the matrix operator that acts on position kets like a derivative, which I'll denote $D_x$.

For our purposes, the former operator acts on functions of a real numbers, say $f(x)$, as $$\frac{\mathrm d}{\mathrm dx}f(x) = \lim_{h\to0}\frac{f(x+h)-f(x)}{h}$$ Since "variable" position bras and kets are also functions of real numbers, taking in a value for $x$ and outputting a bra/ket with that eigenvalue, this derivative can also act on them $$\frac{\mathrm d}{\mathrm dx}\langle x| = \lim_{h\to0}\frac{\langle x+h|-\langle x|}{h}$$ $$\frac{\mathrm d}{\mathrm dx}|x\rangle = \lim_{h\to0}\frac{|x+h\rangle - |x\rangle}{h}$$ Notice this equates $(\frac{\mathrm d}{\mathrm dx}|x\rangle)^\dagger = \frac{\mathrm d}{\mathrm dx}\langle x|$.

The matrix operator $D_x$ exactly like a derivative on position kets, but these need not be functions. Given any fixed position ket, say $|x_0\rangle$, the matrix operator acts as $$D_x|x_0\rangle = \lim_{h\to0}\frac{|x_0+h\rangle-|x_0\rangle}{h}$$ It still can also act on variable position kets and in fact the operators are equal in this case $$\frac{\mathrm d}{\mathrm dx}|x\rangle = D_x|x\rangle$$ Notable differences between operators are that $D_x$ doesn't act on component functions while $\frac{\mathrm d}{\mathrm dx}$ does: $$D_x(\psi(x)|x\rangle) = \psi(x)D_x|x\rangle, \text{ while } \frac{\mathrm d}{\mathrm dx}(\psi(x)|x\rangle) = \frac{\mathrm d \psi}{\mathrm dx}|x\rangle + \psi\frac{\mathrm d}{\mathrm dx}|x\rangle$$ and that $\frac{\mathrm d}{\mathrm dx}$ sets constant kets to $0$ while $D_x$ can still act on constants $$\frac{\mathrm d}{\mathrm dx}|\psi\rangle = 0, \text{ while }D_x|\psi\rangle = \int \psi(x')D_x|x'\rangle\mathrm dx'$$

So with all this clarified, on to your question. Since everything was still ambiguous when you wrote your question I'll cover a few combinations of operators and you can choose which one you were wondering about:

• $\frac{\mathrm d}{\mathrm dx}\langle u| = (\frac{\mathrm d}{\mathrm dx}|u\rangle)^\dagger$ - This is true for arbitrary states $u$ since both sides are $0$ lol. Less trivally, this is true if you replace $|u\rangle$ and $\langle u|$ with variable position states $|x\rangle$ and $\langle x|$ since $$\frac{\mathrm d}{\mathrm dx}\langle x| = \lim_{h\to0}\frac{\langle x+h|-\langle x|}{h} = \left(\lim_{h\to0}\frac{|x+h\rangle-|x\rangle}{h}\right)^\dagger = (\frac{\mathrm d}{\mathrm dx}|x\rangle)^\dagger$$
• $\langle x|D_x = (D_x|x\rangle)^\dagger$ - This is a statement that $D_x$ is Hermitian, i.e. $D_x^\dagger = D_x$, and we will see that this is not true and instead $D_x$ is actually anti-Hermitian, $D_x^\dagger = -D_x$. Since position states form a complete basis we just need to compute its components $\langle x'|D_x|x\rangle$ and compare to the adjoint components $\langle x|D_x|x'\rangle$. $$\langle x'|D_x|x\rangle = \lim_{h\to0}\langle x'|\frac{|x+h\rangle - |x\rangle}{h} = \lim_{h\to0}\frac{\delta(x-x'+h) - \delta(x-x')}{h} = \delta'(x-x')$$ Similarly, $$\langle x|D_x|x'\rangle = \delta'(x'-x)$$ Since $\delta'(x)$ is an odd function, $\delta'(x'-x) = - \delta'(x-x')$ so $D_x^\dagger = -D_x$. Therefore, $$\langle x|D_x = -(D_x|x\rangle)^\dagger$$
• $\frac{\mathrm d}{\mathrm dx}\langle x| = (D_x|x\rangle)^\dagger$ - This is true, from the statement from earlier in the answer $D_x|x\rangle = \frac{\mathrm d}{\mathrm dx}|x\rangle$ and from the first point $\frac{\mathrm d}{\mathrm dx}\langle x| = (\frac{\mathrm d}{\mathrm dx}|x\rangle)^\dagger$.
• $\langle x|D_x = (\frac{\mathrm d}{\mathrm dx}|x\rangle)^\dagger$ - This is not true, since by anti-Hermiticity $$\langle x|D_x = (D_x^\dagger |x\rangle)^\dagger = -(\frac{\mathrm d}{\mathrm dx}|x\rangle)^\dagger$$

We can also look at how the momentum operator works: $$\langle x|P|\psi\rangle = -i\hbar \frac{\mathrm d}{\mathrm dx}\psi(x)$$ So it turns out that the momentum operator in matrix operator form is actually $$P = i\hbar D_x$$ which has a + instead of -. Kinda neat.

Answer 2

The derivative of a bra is equal to the derivative of the adjoint of the corresponding ket with changed sign.

Answer 3

Your question is hard to answer, as it is not quite well defined. In any case, here is how you get out of the woods. Recall $$ \langle x| \hat p / \hbar = -i\partial_x \langle x| \Leftrightarrow \\ (\langle x| \hat p / \hbar)^\dagger = \hat p / \hbar|x\rangle = i \partial_x| x\rangle, $$ so that $$ \langle x| \hat p / \hbar |\psi\rangle= -i\partial_x \psi(x), \\ \langle \psi|\hat p / \hbar|x\rangle= i \partial_x \psi(x)^*, $$ hence $$ \langle x|i \hat p / \hbar |\psi\rangle= \partial_x \psi(x), \\ \langle \psi|-i\hat p / \hbar|x\rangle= \partial_x \psi(x)^* \\ = ( \langle x|i \hat p / \hbar |\psi\rangle)^\dagger , $$ as $i\hat p$ is antihermitian.

You then see that, e.g., $$ \int\!\! dx~~ \psi(x)^* \partial_x \psi(x) = -\int\!\! dx~~ \psi(x)\partial_x \psi(x)^* , $$ so that $\partial_x$ may be thought of as antihermitean in the coordinate representation.

Few would try to formalize this the arguably meaningful way you did.