Comparison between Jones vectors and spin state vectors

Question

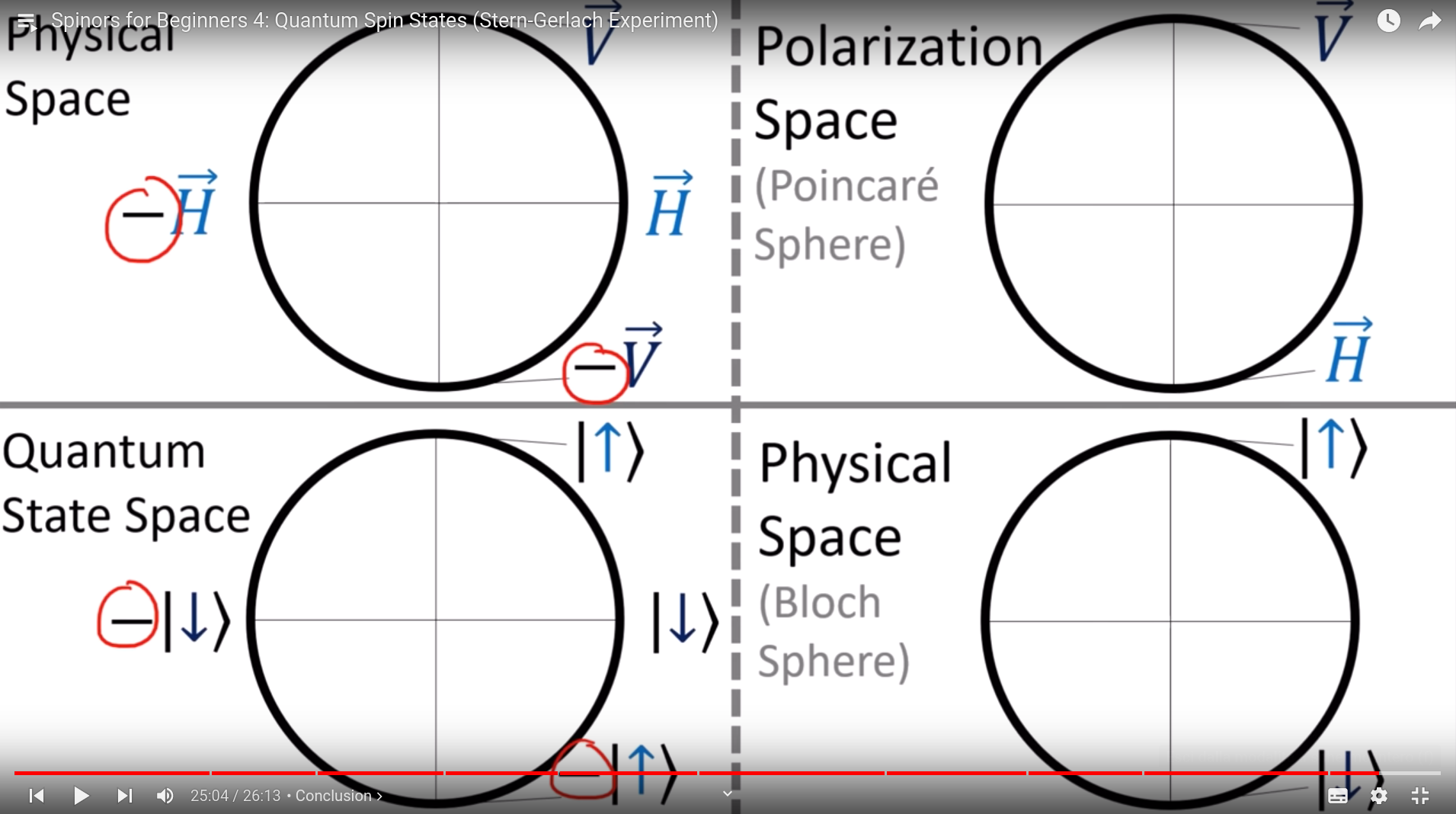
I'm trying to learn about spinors by myself: I've found what it seems to me a very good series of videos on youtube which explains them starting from basic concepts and examples. Now, in this video, the author gives two examples of spinsors, Jones vectors and spin state vectors, since their state space is bound to the physical state by an angle doubling relation. Now, if I understand it right, Jones vectors can be represented as usual vectors (or rotating vectors) in physical state, where each of their component represents the x and y phase factor for the electric field:

$$ \vec{J} = \begin{bmatrix} A^x e^{i \phi_x}\\ A^y e^{i \phi_y} \end{bmatrix} = A^x e^{i \phi_x} \begin{bmatrix} 1\\ 0 \end{bmatrix} + A^y e^{i \phi_y} \begin{bmatrix} 0\\ 1 \end{bmatrix} = A^x e^{i \phi_x} \vec{H} + A^y e^{i \phi_y} \vec{V}$$

on the other hand, it is possible to say that overall phase can be ignored, hence $\vec{J}$ and $-\vec{J}$ represent the same polarization state. Therefore, as the author states, the verse doesn't matter and the state space of the polarization vectors can be represented by the Pointcaré sphere.

Now I find it more difficult to understand this concept for spin state vectors: it seems to me that their behaviour is the opposite of the Jones vector's one. The author carries a parallelism between the Pointcaré sphere for Jones vectors and Bloch sphere for spin states, but, as I understand, the Block sphere represents physical space, where the verse of the spin state vectors matters; in fact the two poles of the Block sphere are occupied by two of the standard basis spin states, that have - physically - an opposite verse, usually $\left\vert z \right\rangle$ and $\left\vert -z \right\rangle$. Now, assuming my understanding is correct up until this point, following the parallelism in state space the verse of spin state vectors should not matter, but, since it holds:

$$ \left\langle +x \vert -x \right\rangle = \left\langle -x \vert +x \right\rangle = 0$$ $$ \left\langle +y \vert -y \right\rangle = \left\langle -y \vert +y \right\rangle = 0$$ $$ \left\langle +z \vert -z \right\rangle = \left\langle -z \vert +z \right\rangle = 0$$

I could represent, for example, $\left\vert +z \right\rangle$ and $\left\vert -z \right\rangle$ as two orthogonal vector in their state space, the same for the other two couples of spin states. Now, how is it possible to say that, since the phase factor can be ignored, the verse of a state vector doesn't matter? I've tried to explain it to myself with the difference between $-\left\vert z \right\rangle \neq \left\vert -z \right\rangle$, but for hypotheses, it is assumed that for $\left\vert +z \right\rangle$ the spin is pointing up, and for $\left\vert -z \right\rangle$ it is pointing down, therefore the previous relation should not be false... I can't find the problem here: if the parallelism holds between polarization states and spin states, I should expect that the state space of spin states has the same characteristics of the physical state of the polarization vectors.

My questions are: is it correct to consider the Bloch sphere to be a geometric representation of the physical space for spin vectors? If so, how could the verse be ignored in the spin state space?

Thank you

Answer 1

Everything seems good here except the link between the Bloch Sphere and what you call "physical space". There are a number of reasons not to think of the Bloch Sphere as a sphere in physical space, but one of the best reasons is the very analogy you started with. The Poincare Sphere is clearly not a good representation of physical space, and the Bloch sphere is also clearly analogous to the Poincare Sphere.

Some other reasons: When you measure the z-component of the angular momentum of a spin-1/2 object, the uncertainty relations mean that you are not measuring the spin as pointing exactly in the z-direction, but rather at some angle to the z-axis. The Bloch Sphere abstracts away this x- and y- component which aren't zero. (Consider that $<S^2> = 3/4\, \hbar^2$, three times bigger than $<S_z^2>$.)

A more subtle reason is that the space of all spinors naturally lives on a 3-sphere -- the symmetry group is SU(2), not SO(3) -- but the Bloch sphere is only a 2-sphere. The way to drop from a 3-sphere to a 2-sphere is something called a Hopf Fibration, which roughly maps to removing the global phase. But evidently this changes the global geometry: a 3-sphere is not just a circle mapped to every point on a 2-sphere.

The conclusion, which I think you've already almost reached, is that Bloch spheres aren't physical.