Trying to get a metric like $ds^2 = -e^{2H_0t} dt^2 + dx^2$ from a higher dimensional Minkowski spacetime

Question

Since the 4D de Sitter spacetime can be found by slicing up a 5D Minkowski spacetime:

de Sitter space from generalized Minkowski spacetime

resulting in a metric like:

$ds^2 = - dt^2 + e^{2H_0t} dx^2$

I'm curious as to whether or not a spacetime where only time dilation occurs, something like:

$ds^2 = -e^{2H_0t} dt^2 + dx^2$.

I realize the curvature tensors for this metric are flat Minkowski, but I'm wondering if it (or the log(), in reverse) can be induced from a hyperboloid like de Sitter space can?

---

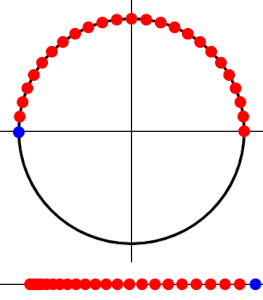
The reasoning for this is that I've found a universe with time dilation and no expansion to be a rather superb fit for Pantheon+SH0ES dataset. Although I'm told this isn't very convincing without a geometrical foundation. I thought that if time were shaped like a circle, with regularly occurring events spaced evenly along on the circle:

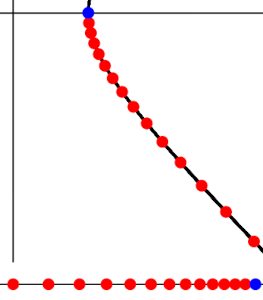
That if our measured coordinates were somehow our distance from the present to the past events in the 2D space, circular time would be observed as time contracted, and electromagnetic waves would have their periods squished, making them blueshifted. So hyperbola?

This seems promising. The question is, can you get there from the same general idea as starting with a higher dimensional Minkowski spacetime? And does it need to be $\mathbb{M}^{3,2}$, eg.:

$ds^2 = - dx_0^2 - dx_1^2 + dx_2^2 + dx_3^2 + dx_4^2$

?

Answer 1

Your metric is equivalent to the normal Minkowski one with $t>0$. The normal Minkowski metric is $$ ds^2 = -dt^2 + d\vec{x}^2 $$ Defining the new time coordinate via $$ dt = e^{H_0 T} dT $$ we get your metric $$ ds^2 = - e^{2H_0 T} dT^2 + d\vec{x}^2 $$ So you can think of your metric as being a flat spacetime, with a wonky time coordinate. You could think of this coordinate as describing a clock that effectively slows down as time goes on, not unlike a real clock with a battery that is going bad. Nothing physical should depend on the clocks we choose to use, so the physical predictions you obtain with these coordinates should be identical to the ones you would obtain with Minkowski spacetime in the usual coordinates.

We can explicitly solve the implicit equation for $T$ given above to get $T$ in terms of $t$ $$ t = T_0 e^{H_0 T} \implies T = H_0^{-1} \log\left(\frac{t}{T_0}\right) $$ where $T_0$ is an integration constant. From this, it's easy to see the coordinate transformation is valid for $t>0$. So your metric will cover the coordinate patch of the full Minkowski space for $t>0$. In that sense, it is similar to Rindler coordinates, which cover a patch of the full Minkowski space.

It is trivial to find ways to embed this spacetime in higher dimensions. For example, you could take a five dimensional Minkowski spacetime, with coordinates $t, x_1, x_2, x_3, x_4$, and restrict to $t>0$ and $x_4=0$.

I don't see how it would be possible for a flat spacetime metric to explain all cosmological observations to date. For example, big bang nucleosynthesis only works if there is a physical scale associated with the Universe's expansion that eventually causes the Universe to cool to the point that big bang nucleosynthesis stops.