How can I mathematically model the entrainment of air through a window?

Question

The Problem

Suppose I have an open window with an area $A$, and a uniform wind source (a fan) pointing towards the window, positioned at a distance $s$ away from the window.

At the exit of the fan, let the velocity of the air be, uniformly, equal to $u$. How can one calculate the average velocity $v$ across the window? If not calculate, how can one even model this system?

What I've found so far:

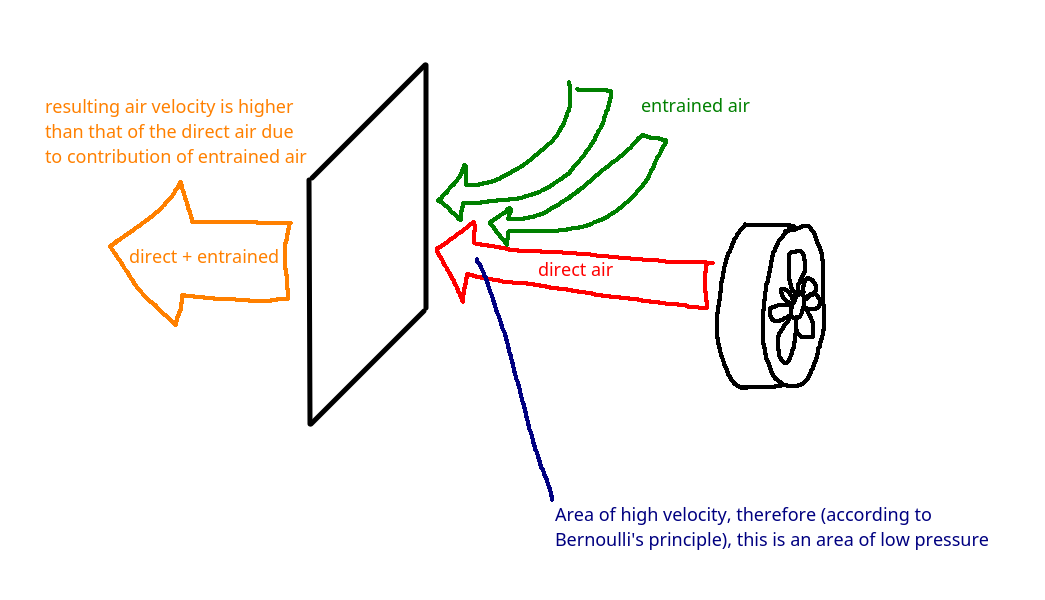
Bernoulli's principle tells us that in an area of high velocity in a fluid, there is also an area of proportionally lower pressure. This means that air from the surroundings is drawn in, and contributes some velocity to the air that makes it through the window. This is called entrainment.

What I'm missing:

Everywhere I've looked online, there is only a written explanation of entrainment like the one you see here. Nowhere can I actually find an equation or even a proportionality between the distance of the fan from the opening and the contribution of entrained air to the final velocity. Is there some equation that models entrainment? How can I even get started at modelling this system mathematically rather than intuitively?

I would really, really, really appreciate it if anyone could help me out with this.

Answer 1

To get a better feel for what's happening here, I would want to have a more concrete picture of the streamline pattern. I would have to include the rest of the room where the air circulation is occurring. Part of the room air is entrained and part passes through the fan. So there is a split in the streamlines between these two parts of the circulation.

Another consideration is that with air leaving through the window, there has to be a source of air seeping into the room from elsewhere in the house (and ultimately from outside), say the vent ducts. Otherwise the pressure in the room would keep dropping.

I would initially start out modeling this as a circular window (rather than rectangular), and a long cylindrical room (rather than "cubical") so that my early models and consideration of the streamline pattern would be 2D axisymmetric. I would add more geometric complexity later. The source for the air leaving the room would be a uniform stream of flowing air entering far down the cylinder (to the right). I would then start by drawing what I expected the streamline pattern to look like for this simplified geometric arrangement.

Hope this helps.

Answer 2

The idea that Bernoulli shows that the air in the rapidly moving stream has lower presure is false. They have the same pressure. This is one of the many myths perpetuated by badly written high-school textbooks. The Bournoili equation $v^2/2+P=constant$ applies only along streamlines and cannot be applied to the air inside and ouside a jet.

The Air is entrained because the interface seperating the rapidly flowing jet and the stationary air is unstable--- the Kelvin–Helmholtz instability. The resulting mixing of the fast-flowing air with the static air is what entrains the room air. The video in the linked article shows how complicated the resulting flow is. There are probably empiricle formulae, but you want an exact answer you need to buy a supercomputer.

Answer 3

I don't necessarily agree with @MikeStone's comment Bernoulli is 'wrong' but according to George Box's claim "all models are wrong, [but] some are useful" Even simple experiments demonstrate that higher velocity flows reduces pressure within the stream relative to stagnant gas outside the stream - and so potential to permit acceleration of surrounding stagnant gas I believe is necessary - but not sufficient. In laminar flows, low Reynold's number, streams tend not to cross one another. So he is right that turbulence needs to occur - rotation. There are papers discussing theories of eddies 'biting off" parcels of gas outside the jet causing mixing and entrainment into the jet. But you also need potential for efficient entrainment.

The 'geometry' of entrainment problems are all virtually different from one another and this will set the boundary conditions in space. What is also important and common to all fluid mechanics problems are the conservation laws: mass (continuity), momentum, and energy. You have to define a 'control volume' to bound the space under consideration, define the inputs/outputs of mass flow and energy (is the problem isothermal? , adiabatic?). And once all that is decided, apply the conservation laws to solve for what you decide is the 'output' as a function of the inputs and system parameters.

That type of a solution is a rough approximation based on a simplified 'model' and so as @MikeStone also said, if you need more accuracy you need to turn to numerical methods - computational fluid dynamics. I consider these 'Brute Force' since they force the problem into the framework of the Navier Stokes equations- yet another model. Even Navier Stokes is 'wrong', but often useful.