Is Time Dilation Directional Under Special Relativity, What Fundamental Concept Am I Missing Here?

Question

I'm starting college this summer and trying to learn physics. Special relativity is new. I thought I was getting a handle on it but nope... I'm just trying to nail the concepts down to give the math some context. I have tried to piece together special relativity bit by bit so any time I say 'I Learned' or 'I Was Taught' I probably misunderstood something. I really appreciate y'all's help and patience.

Here's the thought experiment I've had stuck in my head:

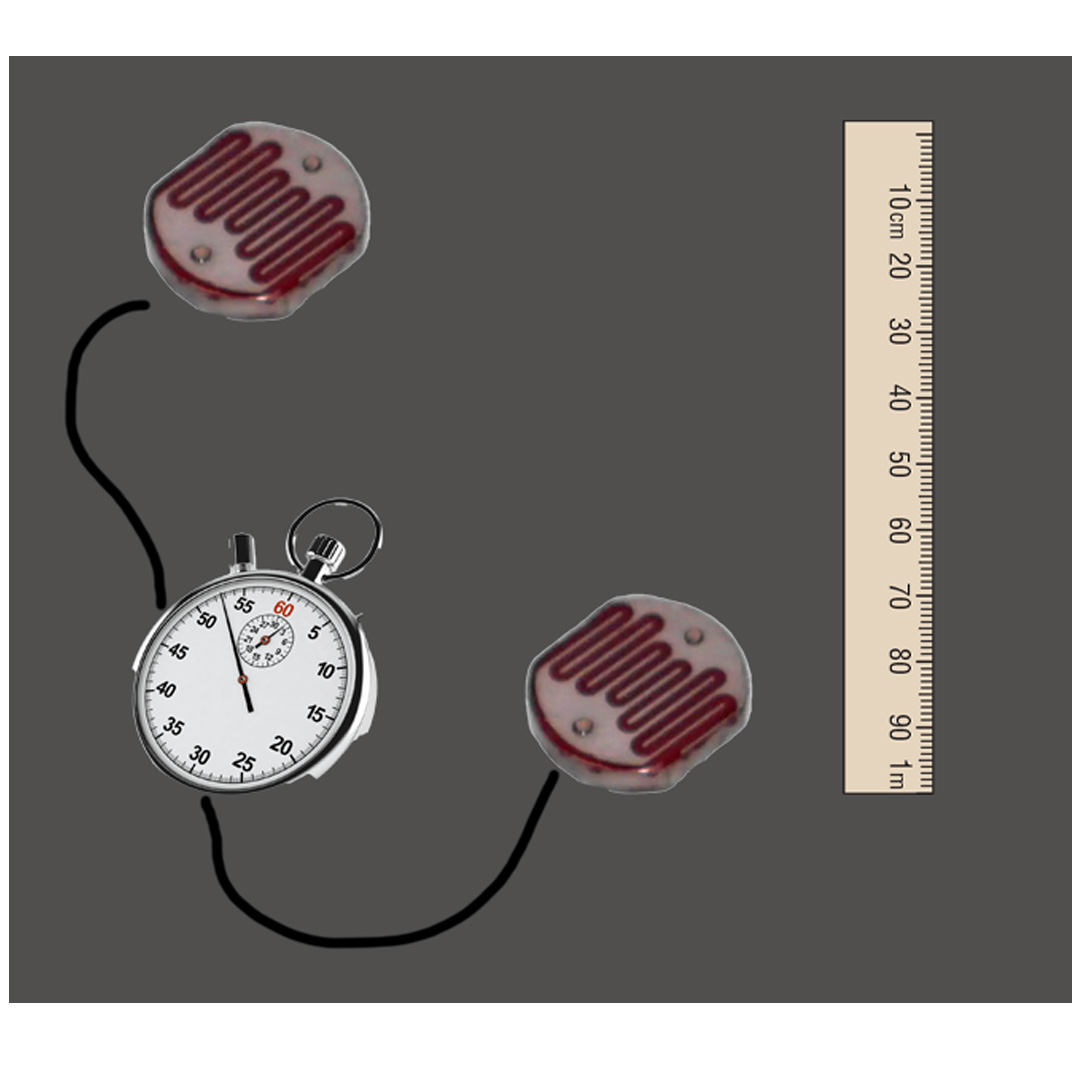
Lightspeed Measuring Device:

Just an imaginary device with two photocells, a clock, a ruler, intended to perfectly measure the speed of light from one direction

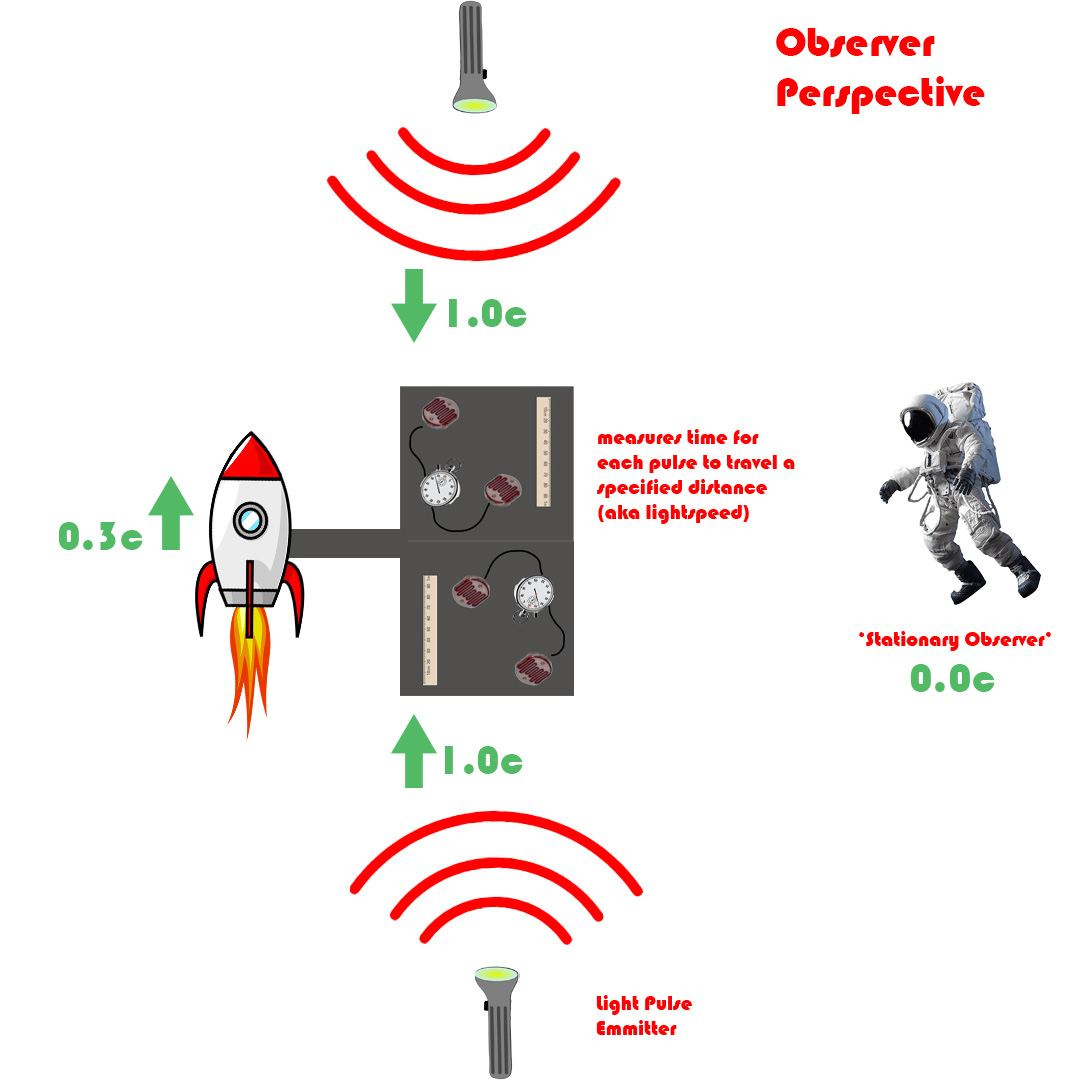
Stationary Observer's Perspective:

A stationary observer in space, a rocket travelling at 0.3c with two light measuring devices attached, pointing parallel to direction of travel, but in opposite directions. In both directions is a pulsing light, allowing the speed of light to be measured from forward and rear of the rocket.

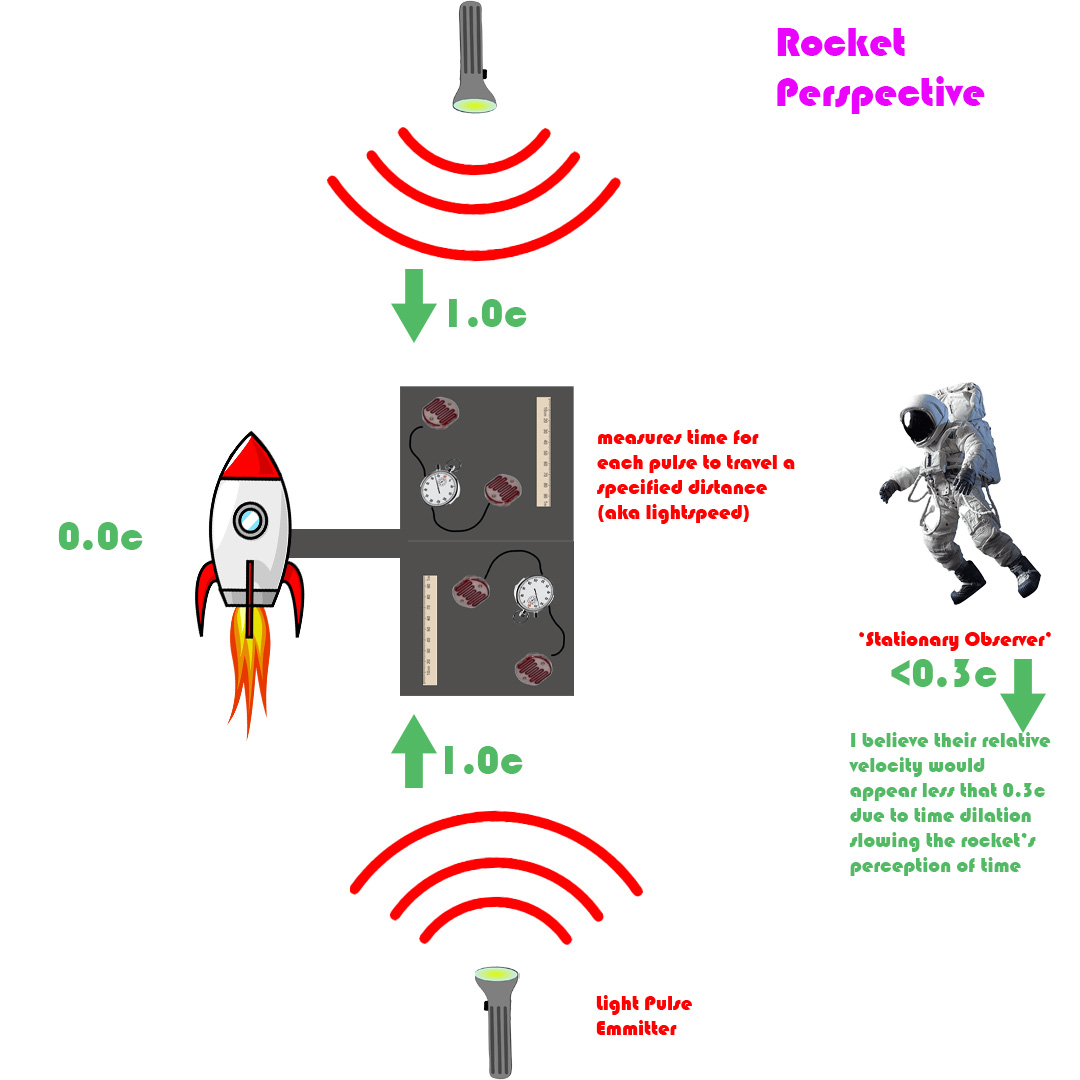
Rocket Perspective:

The same scene as above, but from the rocket's perspective. The stationary Observer is now traveling down relative to the rocket at less than 0.3c. The light is traveling to the rocket from opposite directions at a relative speed of 1c. And the rocket is merely stationary.

I've probably already got something wrong in this image. The stationary observer is moving away from the rocket at <0.3c because I know the rocket is experiencing relative time dilation from moving faster than the observer, so I imagine the observer's relative velocity will appear less because time is ticking slower. This scene is What I believe the "Correct" understanding of relativity looks like, but I don't yet know how to get here conceptually. The next scene should highlight my confusion and make you roll your eyes at my ignorance.

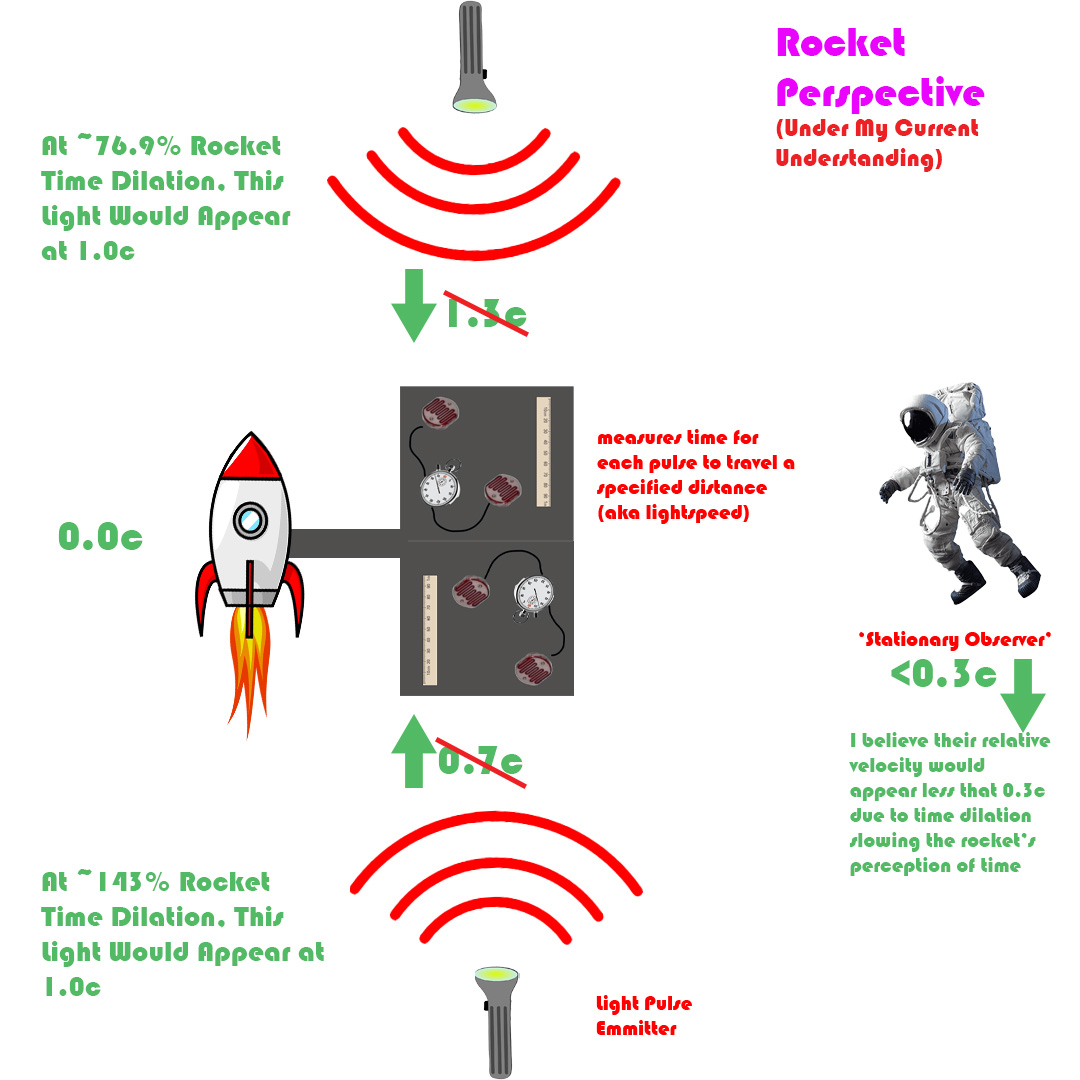
My Current Understanding Of The Rocket's Perspective

Okay, so here's where the s*** hits the fan...

My understanding is that without special relativity, the rocket's velocity would add to and subtract from the light's relative velocity in front and behind the rocket. Obviously this can't be the case for a lot of reasons. I believe the reason lightspeed can remain constant is because:

1. Speed = distance/time
2. When increasing speed, unit time 'scales' up, perception of time slows down.

So instead of measuring light from in front of the rocket at 1.3c, the measuring device would measure light at 1.0c because it is experiencing time at ~76.9% the stationary observer's perspective. Although that is probably where my misunderstanding lies, I'll finish my thought experiment. If you were to measure the light coming from behind the rocket, the rocket would need to experience time at ~143% the stationary observer's perspective in order for the light to be measured at 1c.

Once again, thank you all for your time I hope I can get this figured out as it's driving me nuts.

Answer 1

Measurements of relative velocity between massive objects (the rocket and the space suit) are symmetric. Measurement of time dilation is non-directional and symmetric. The rocket measures the astronaut traveling south at $v$ and time dilated by the Lorentz factor $\gamma (v)$, while the astronaut measures the rocket traveling north at $v$ and time dilated by $\gamma(v)$.

It is the order of events which is different between frames. If the north flash and south flash are simultaneous in the space suit's frame, the space suit sees the north-traveling rocket illuminated first by the north flash and then by the south flash because the rocket is traveling towards the north source and away from the south source. In the rocket frame, the north flash precedes the south flash, and the south-traveling space suit is illuminated by the later south flash at the same time as the earlier north flash because it was traveling towards the south emitter and away from the north emitter.

See: https://en.wikipedia.org/wiki/Relativity_of_simultaneity

---

Side note: if the rocket was instead flying away from or towards the space suit, they will also see each other's signals (including light bouncing off of them picked up by a telescope) "stretched" or "compressed" in time by doppler shift, similarly to how you hear a song faster when it is being blasted by a stereo approaching you and slower when the car is driving away.

Answer 2

This is really long and there is a lot going on, but I think your question is at the end regarding speeds. First of all, you can't think of velocity addition the intuitive way. The correct SR velocity addition and subtraction can be found here. Under this, if either velocity is c, the formula will evaluate to c. This means that light appears to be moving c no matter how an observer is moving. Everyone agrees on that velocity, rather than what we intuitively think of what people agree on (e.g. how long a ruler is or how many seconds ticked on a clock).

Settling "why" another observer sees something (so in this case, the stationary observer asks "why" the rocket bound observer sees something) is always settled with the Lorentz transformation. The "rules of thumb" you hear in popular science are roughly true but not precise enough. If you are confused by something and think you have a relativistic paradox, always use the transformation to settle what really happened.

When using the transform to settle a question, always remember that an "event" in relativity is a set of coordinates (time and however may space coordinates you are considering). "What the other observer sees" is non technical language referring to "the event according to the other observer" which literally means coordinates (space and time). Those coordinates are found by the transformation.

You can think of our intuitive view of nature as being that anyone can start a stopwatch at will, but time passage is the same for everyone. Also, anyone can stand wherever and face whichever way they want, but distances and angles do not change when this happens. Velocity has no effect on space and time. This is the "Galilean transformation" on which Newtonian physics is built. From a certain perspective, SR is just the same physical ideas built on the Lorentz transformation of space and time, which is really a kinematic change. SR still allows you to start the stopwatch at will and stand and face where you want, but if you are moving relative to another observer, you will not agree on time passage, all distances, or all angles.

I would recommend reading an actual introduction from a real physics book. The standard "Gen Phys" books for undergrad and high school generally have a chapter on SR which will be more precise than the pop science stuff you see in media, but still manageable by someone at your level.

I'm not just being pedantic. If, for example, your stationary observer sees both flashlights pulse on and off at the same time, and obviously the two pulses of light will arrive at him in the middle at the same time, the rocket bound observer likely will not agree that the two flashlights were pulsed at the same time. This breaking of the agreement upon simultaneity is a part of how the "paradox" is resolved that both observers see both light beams moving at c in opposite directions. Disentangling what actually happened is by no means obvious to a beginner.