What two spacetime points would a rocket observer have to choose to find a length which agrees with a laboratory observer?

Question

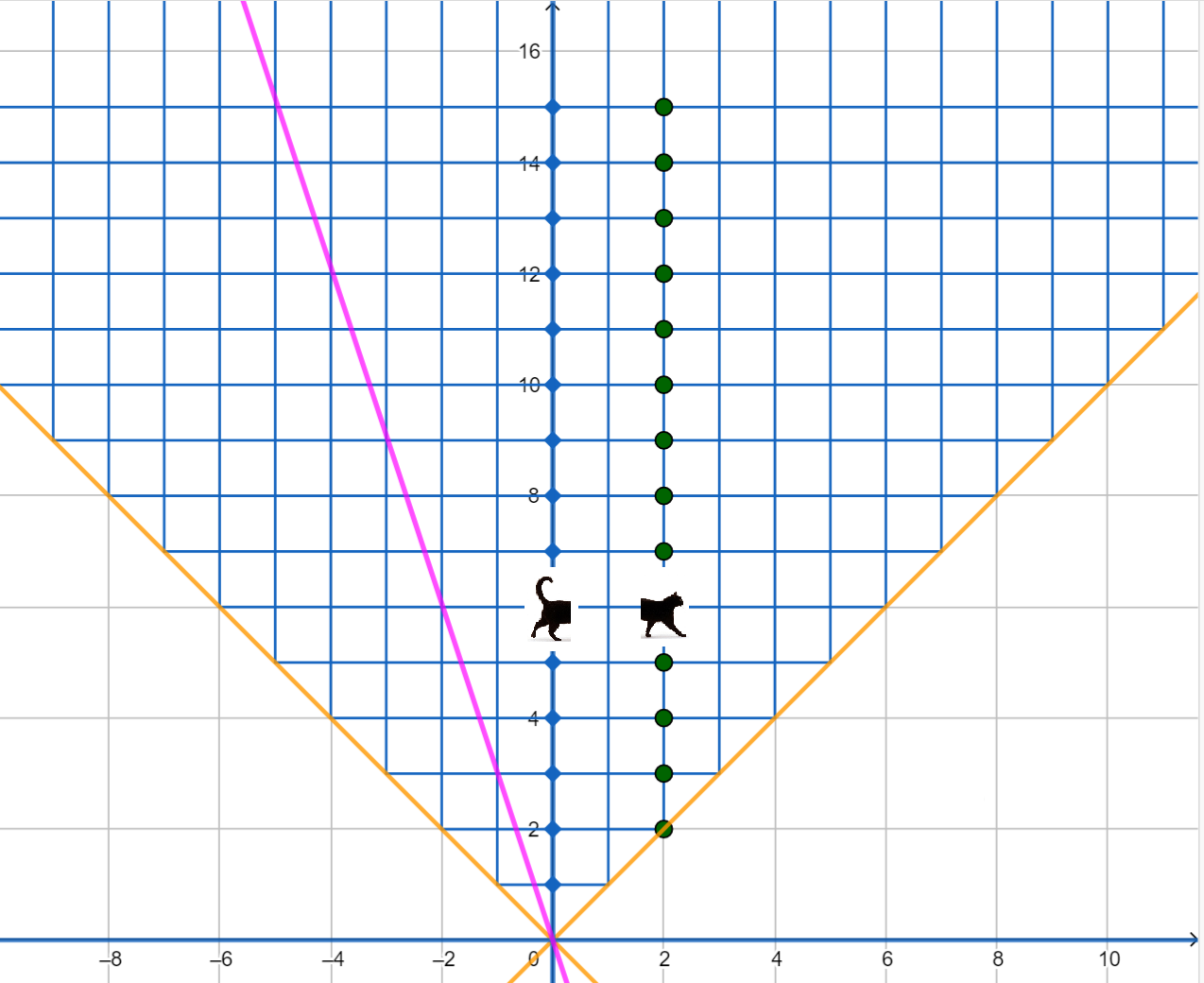
A cat is stationary in the laboratory frame, it's tail at $x = 0$ and its head at $x = 2$, so it's length is $l = 2$. A rocket (pink worldline) is moving to the left of the lab at one-third the speed of light.

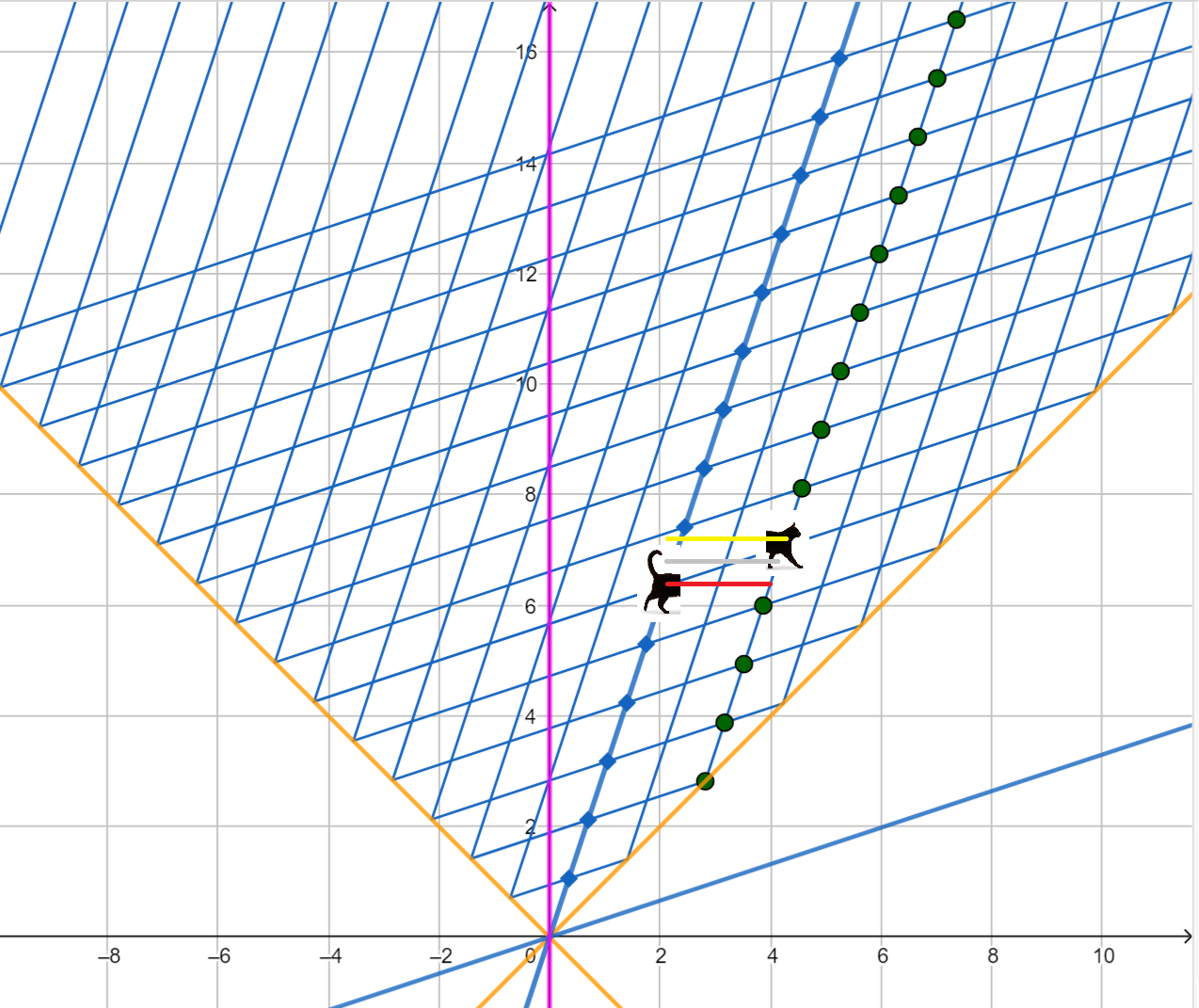
Now, let's do a Lorentz transformation to see things from the rocket's reference frame.

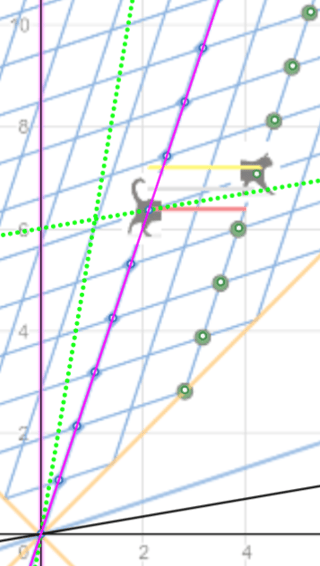
If the rocket observer measures the spatial distance between the two ends of the cat that he can observe at the same time, which I've represented with a red line, he'll find that the cat's length is $l' < 2$. This is length contraction and comes from the fact that, from the perspective of the laboratory observer, the rocket observer is measuring the distance between the "present tail" and the "past head".

Now, if the rocket observer marks the point in space where the lab's "present tail" is, waits a while, then marks the point in space where the laboratory's "present head" is, then measures the spatial distance between those two points, he'll find a length $l' > 2$. I've represented this with a yellow line.

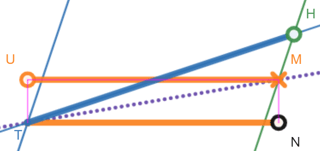
Between these two possibilities, of course, there's a way the rocket observer can compare the laboratory's "present tail" to some "not quite present head", a possibility I've marked with a grey line, that would result in $l' = 2$. My intuition tells me this midway point probably has some interesting physical or geometrical properties, but I can't really figure out what they would be. What is this point and what's its significance?

Answer 1

For simplicity, it's good to consider the measurements when the tail is at the meeting-event (that is, the left-event on the red segment is the origin meeting-event).

Furthermore, to make the arithmetic easier, it's better to choose a relative-speed from among (say) 0, 3/5, 4/5, or 5/13 (which leads to pythagorean triples [and rational Doppler factors]).

---

Assuming I am reading your diagram correctly, it seems that

• The grey segment can be interpreted as a certain identical cat at rest in the rocket frame (the rocket-cat) at the instant when the midway-event occurs in the rocket frame.

• When the lab-cat-tail-measurement is made [the left-event of the red segment], the tails of the lab-cat and this rocket-cat meet.

• The 'midway-event" [the right-event of the grey segment] occurs when the heads of the lab-cat and this rocket-cat meet.

I don't have time for a proof right now,
but I think you'll find that the following is true.

• Consider the "Center-of-Momentum frame" (akin to the worldline that bisects the relative-rapidity between the frames).
  In your case, it is the velocity $v_{CM}=\tanh(\mbox{arctanh}(-1/3)/2)=(2\sqrt{2}-3) \approx -0.1715728$ https://www.wolframalpha.com/input?i=tanh%28arctanh%28-1%2F3%29%2F2%29
  (For $v=-3/5$, we have $v_{CM}=-1/3$. For $v=-4/5$, we have $v_{CM}=-1/2$.)
• The tails-meet-event [the left-event on the red segment]
  and the "midway" heads-meet-event [the right-event on the grey segment]
  are simultaneous according to this CM-frame.
  Thus, one can locate this "midway-event" using the space-axis of the CM-frame.

Rephrasing,

• the "midway" heads-meet-event is the event that the CM-frame says is simultaneous with the tails-meet-event.
• geometrically, the "midway" event is the intersection of the lab-cat-head worldline and the rapidity-angle-bisector (with vertex at the tails-meet-event) between the space-axes of the lab and rocket frames.

---

update

Here's my attempt to draw over your diagram the line of simultaneity according to the CM-frame through the tails-meet-event.

---

update2

Here's a Minkowski-spacetime-geometric proof.

• Based on your spacetime diagram drawn from the "rocket frame", consider the following figure. In this frame, the lab and "lab-cat" move in the forward direction.

• TH is the "lab-cat", the cat at rest in the lab frame. TH has proper-length 2.

• M is the "midway" event on the cat-head-worldline,
  so chosen so that UM is your grey segment of length 2 (the rocket-instant of what I called the "rocket-cat").
  The parallel segment TN also has length 2.

• The open circles represent vertices with Minkowski-right-angles between the drawn lines that meet there.
  MN and TN are Minkowski-perpendicular since MN is parallel to the rocket-worldline and TN is a line of simultaneity for the rocket.
  MH and TH are Minkowski-perpendicular since MH is parallel to the lab-worldline and TH is a line of simultaneity for the lab.

• Observe that TM is a hypotenuse (opposite the right-angle at N) for right-triangle $\unicode{0x22BF}{\ TNM}$, with TN as the adjacent side. So, $TN=TM\cosh\theta_{NTM}$, where $\theta_{NTM}$ equal to the rapidity-angle between TN and TM.

• Observe that TM is a hypotenuse (opposite the right-angle at H) for right-triangle $\unicode{0x22BF}{\ THM}$, with TH as the adjacent side. So, $TH=TM\cosh\theta_{HTM}$, where $\theta_{HTM}$ equal to the rapidity-angle between TH and TM.

• But since TH=TN (and TM=TM), then $\theta_{NTM}$ and $\theta_{HTM}$ have equal magnitudes... thus, each is half of $\theta_{NTH}$. That is to say, TM is the rapidity-angle-bisector of $\theta_{NTH}$. ($\unicode{0x22BF} {\ TNM}$ and $\unicode{0x22BF}{\ THM}$ are isometric right-triangles in Minkowskian spacetime geometry.)

• Thus, TM is a line-of-simultaneity for the "center-of-momentum frame". (Use my calculation above to determine the velocity of that frame.)

• ($\unicode{0x22BF} {\ TNM}$ and $\unicode{0x22BF}{\ THM}$ are reflections of each other about the spatial-axis in the the center-of-momentum CM-frame. This reflection relationship is true in all frames… but may be more recognizable in the CM-frame.)

Answer 2

The Lorentz factor is a particular case of a factor which depends on the angle between space and time, for example to find the $\gamma$ factor directly, we use the Pythagore theorem $\vec{c}^{2}t'^{2}=\vec{v}^{2}t'^{2}+\vec{c_{0}}^{2}t^{2}$$,\;\;|\vec{c}|=|\vec{c_{0}}|=c$ (the case where $\vec{c}_{0}\perp \vec{v}$, i.e. the observer moves orthogonally away from the event ), in the ca where it is $\,\vec{c}\perp \vec{v} \,$ we have $K=\frac{1}{\sqrt{1+\frac{v^{2}}{c^{2}}}}\,$(i.e. the observer approaches the event orthogonally ).

So the calculation of the length depends on the angle and direction of the observer in relation to the event.

Answer 3

In the case in which the rocket observer calculates the proper length of the cat by taking one measurement earlier than the other, what observer is doing is taking two measurements at times that are simultaneous in the cat's rest frame.