Understanding simultaneity through male and female clocks which meet halfway

Question

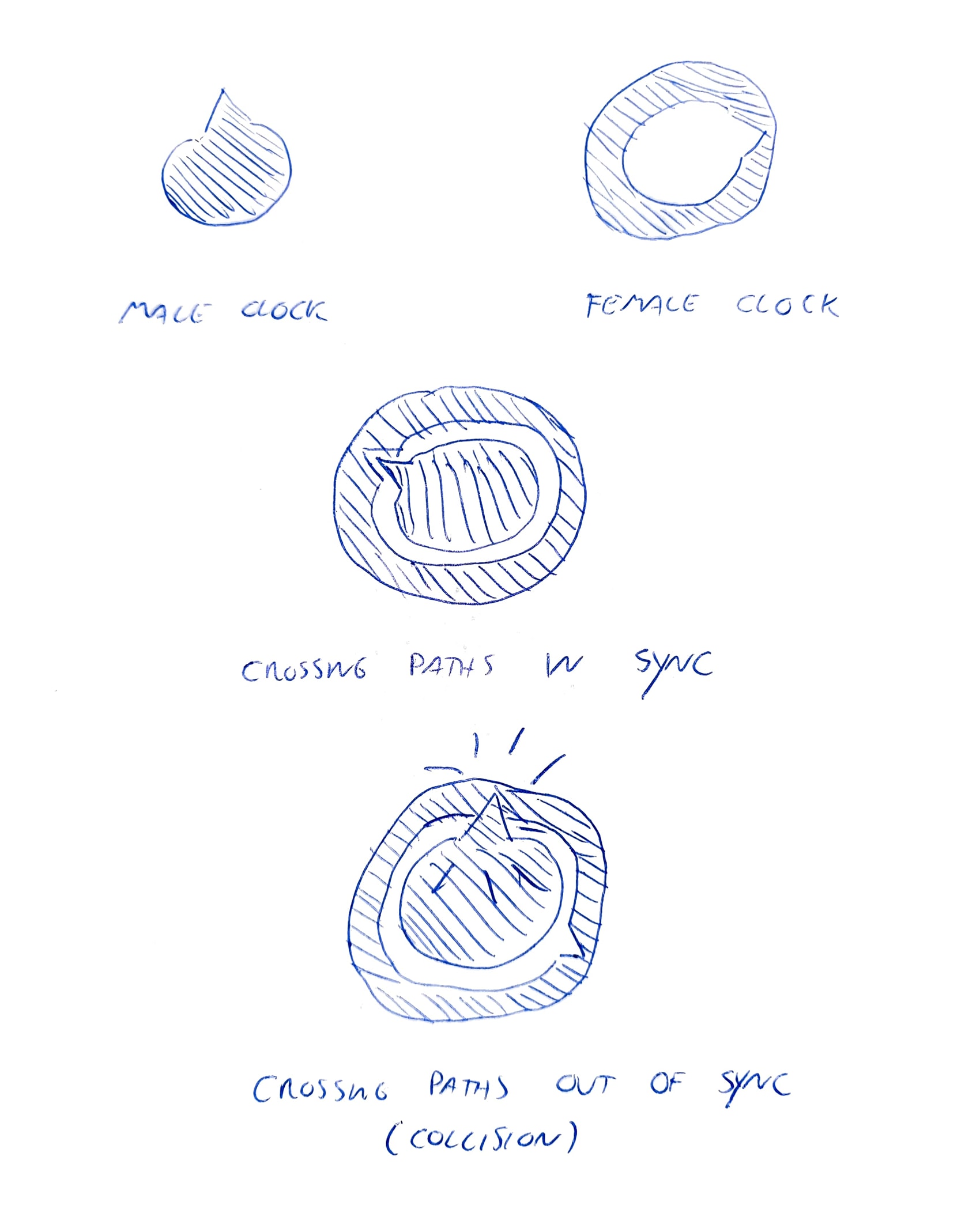
This is not a homework question, just a scenario I've come up with. Imagine I have a male and a female clock moving towards each other. If they're in sync, one will fit inside the other and they'll continue on their way. If not, they'll collide. (Apologies for the crude drawings.)

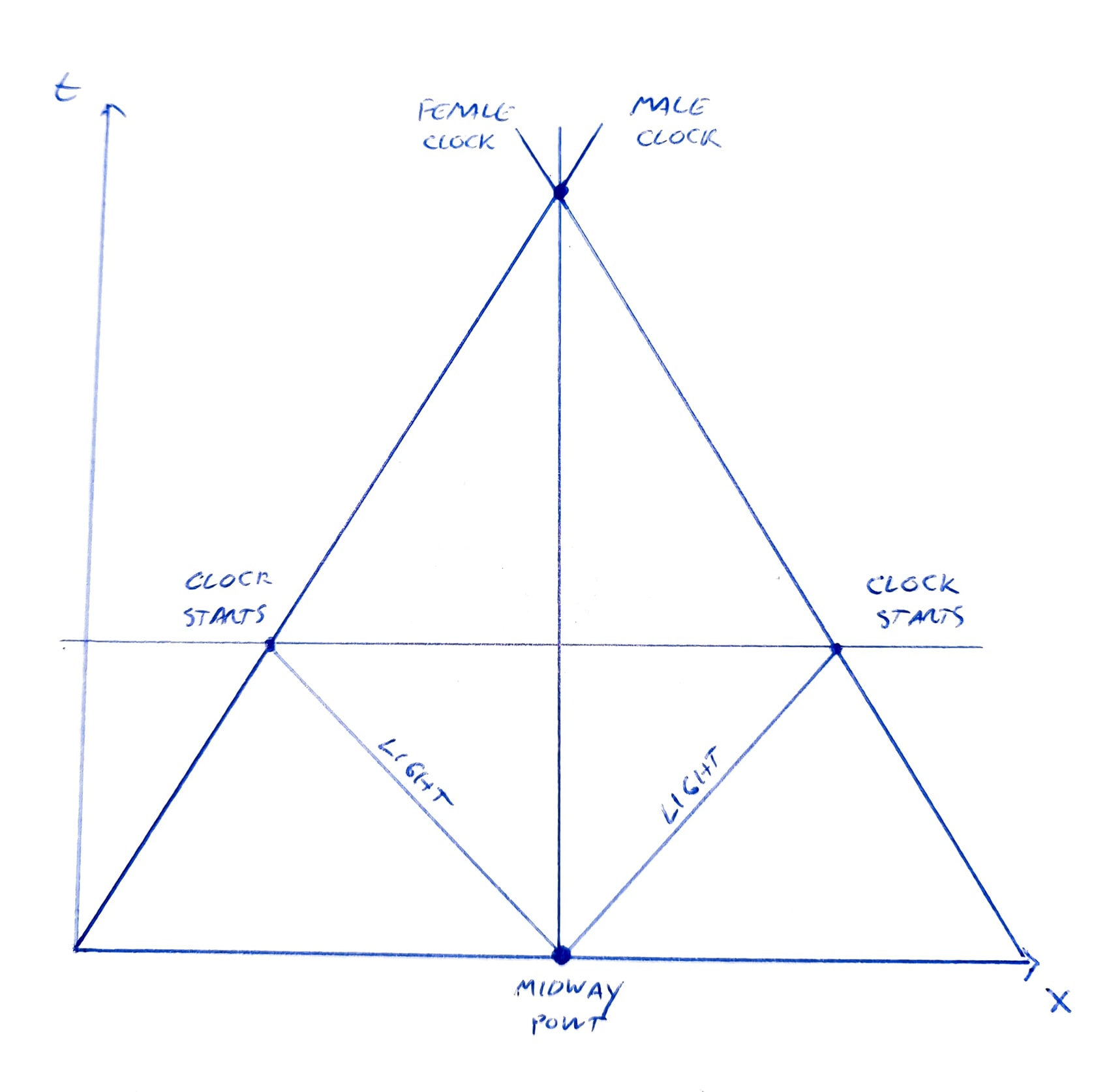
I place these clocks the same distance away from a midway point which contains a light bulb. The clocks travel toward the midway point at the same speed, meeting at that point some time later. Before the clocks start their journey, I set them to show time zero and turn them off. As soon as the clocks start, the light bulb emits a flash that travels in all directions. When that flash hits the clocks, they turn on and start keeping time.

From a reference frame at rest with respect to the midway point, the clocks will always be in sync and when they meet at the midway point the male clock will pass through the female clock with no issue. Therefore, this is the outcome we must expect in all reference frames.

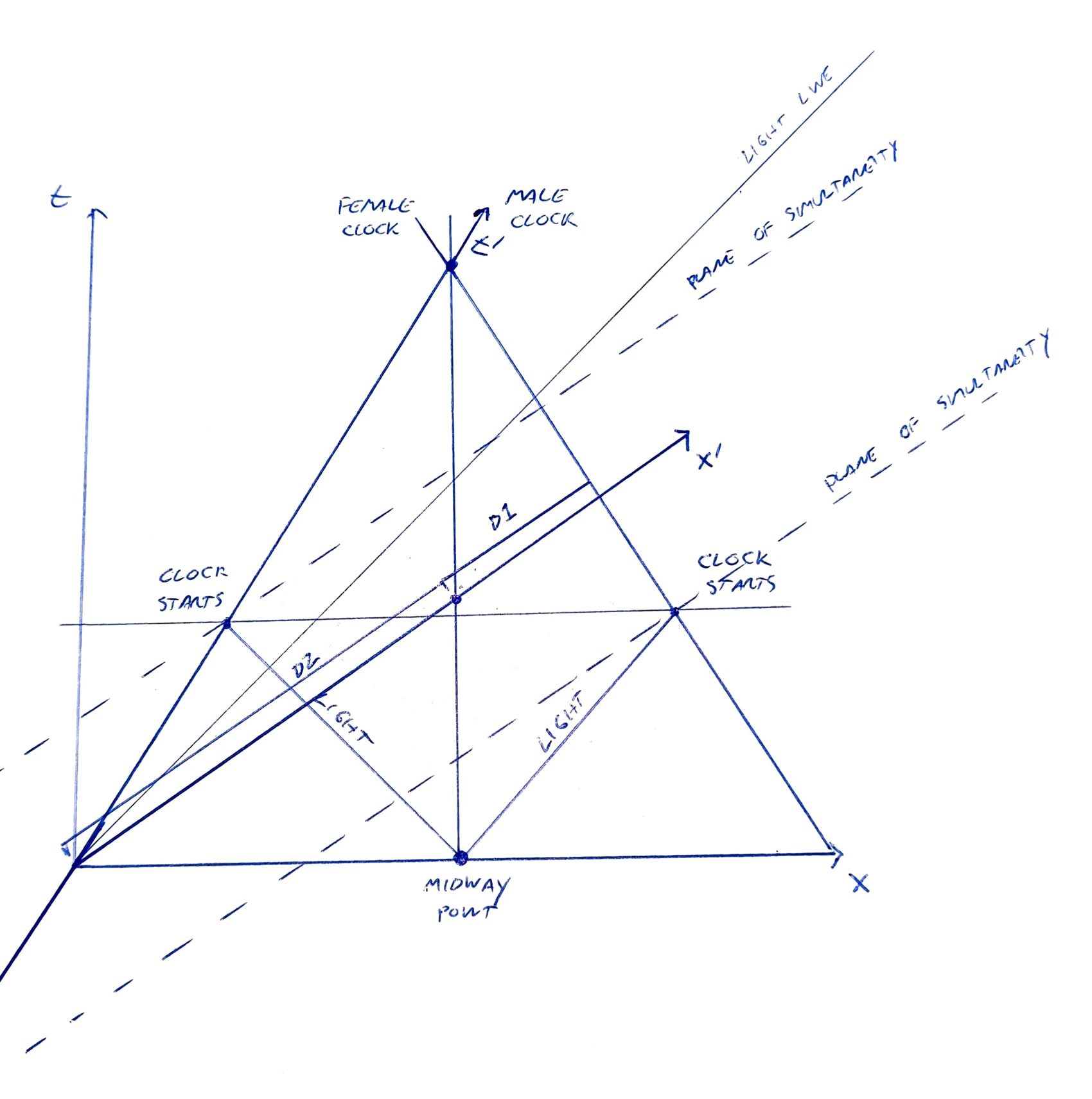
Now, let's analyze things from the reference frame of the male clock. In the image below, I've drawn the axes corresponding to that frame.

As we can see, from the male clock's frame the light flash starts the female clock first and only some time later starts the male clock. Therefore, the female clock gets a head start. However, from the male clock's frame the female clock also runs slow due to time dilation. To avoid a paradox, these two facts must combine in order to allow for a perfect synchronization of the clocks at the midway point.

What I'm having a hard time figuring out is how to make this analysis algebraic in order to demonstrate that this synchronization does happen from the male clock's frame. How can I show that from the male clock's frame, the female clock's head start is compensated by time dilation?

Bonus question (which is also confusing me): how come from the male clock's frame, at any given point in time, the distance D2 between the male clock and the midway point is larger than the distance D1 between the midway point and the female clock? Shouldn't I expect both distances to contract equally? This is the difficulty which has prevented me from drawing a new spacetime diagram from the perspective of the male clock (I can't figure out why the initial conditions would be spatially asymmetric).

Answer 1

How can I show that from the male clock's frame, the female clock's head start is compensated by time dilation?

This is rather tedious but straightforward using the Lorentz transform. I will use units where $c=1$ and I will use four-vectors of the form $(t,x)$.

In the original frame the worldlines of the male and female clocks are given by $$r_m(\tau_m)=\left( \frac{\tau_m}{\sqrt{1-v^2}},v\frac{\tau_m}{\sqrt{1-v^2}} \right) = (t,x_m)$$ $$ r_f(\tau_f)=\left( \frac{\tau_f}{\sqrt{1-v^2}},-v\frac{\tau_f}{\sqrt{1-v^2}} + 2d \right) = (t,x_f) $$ from this it is easy to show that $\tau_i^2 = \Delta t^2-\Delta x_i^2$ for $i \in \{ m,f \}$, so $\tau_i$ is the invariant proper time for each clock with the zero for each clock set at $t=0$. Then it suffices to show that at the collision, where $r_m=r_f$, we have $$\tau_m=\tau_f=\frac{d\sqrt{1-v^2}}{v}$$ Since the $\tau_i$ are frame invariant, this guarantees that in all frames they will line up at the collision. There is no need to go further.

However, if we want to go further we can. Suppose we Lorentz transform to a new (primed) frame moving relative to the first (unprimed) with speed $v$. Then, in the new frame we have $$r'_m(\tau_m)=\left( \tau_m,0 \right)=(t',x'_m)$$ $$ r'_f(\tau_f)= \left( \frac{-2dv\sqrt{1-v^2}+(1+v^2)\tau_f}{1-v^2}, \frac{2d\sqrt{1-v^2}-2v \tau_f}{1-v^2} \right) = (t',x'_f) $$ This expression is really ugly, but with some algebra we can set $t'=0$ and solve for $\tau_f$ to determine the amount that the female clock is set ahead in the primed frame at $t'=0$ due to the relativity of simultaneity, $\mathrm{ROS}$. When we do so we get $$\mathrm{ROS}=\frac{2dv\sqrt{1-v^2}}{1+v^2}$$ We can also take the derivative of $t'$ with respect to $\tau_f$ to get the time dilation of the female clock in the male clock's frame, $\gamma_f$. $$\gamma_f=\frac{1+v^2}{1-v^2}$$ So, in this frame the collision occurs at $$t'=\frac{d\sqrt{1-v^2}}{v}$$ and at that time we have $$\tau_f=\frac{t'}{\gamma_f}+ROS=\frac{d\sqrt{1-v^2}}{v} = \tau_m$$ indicating again that the male and female clocks line up at the collision.

Answer 2

I would like to complement Dale's excellent (as always) answer by addressing your subsidiary question about why the midpoint in the initial rest frame is not also a mid-point in the frame of either clock.

The answer is that lengths contract according to the velocity at which they appear to be moving. In your diagram there are three velocities. In the frame of the male clock, the female clock is coming closer at one speed, while an object at the midpoint in the initial rest frame will seem to be approaching at half that speed, so the distance to the object at the initial midpoint is length contracted to a lesser degree. You would only expect the initial mid point to remain the midpoint if the female clock was stationary in the initial rest frame.