Question regarding beats

Question

If two waves of different frequencies, say $x_1=A\sin(\omega_1t)$ and $x_2=A\sin(\omega_2t)$, are moving along in the same direction and these waves satisfy the condition $$|\omega_1-\omega_2|\ll \omega_1+\omega_2, \tag{1}$$ then we can observe a beat pattern.

Now the superposition of the wave can be given by $$x=A\sin\left(\dfrac{(ω_1-ω_2)t}{2}\right)\cos\left(\dfrac{(ω_1+ω_2)t}{2}\right).\tag{2}$$

Now I presume that the $A\sin((ω_1-ω_2)t/2)$ term of equation 2 doesn't change as fast as $\cos((ω_1+ω_2)t/2)$ because of condition $(1)$, so we can assume that the amplitude part of the equation is contributed by the term with $ω_1-ω_2$ and the frequency of the resultant wave is contributed by $ω_1+ω_2$. I understand up to this.

My question is, what is the beat frequency here? Why is it given by $ω_1-ω_2$ rather than $\dfrac{ω_1-ω_2}{2}$? As we discussed earlier, isn't the frequency of resultant wave given by $\dfrac{ω_1+ω_2}{2}$? Is beat frequency different from the frequency of resultant wave?

Answer 1

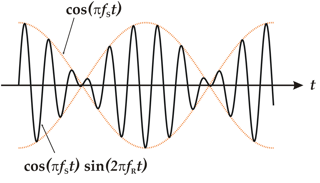
The equation $x=A\sin\left(\dfrac{(ω_1-ω_2)t}{2}\right)\times \cos\left(\dfrac{(ω_1+ω_2)t}{2}\right)$ can be thought of as consisting of two parts which are multiplied together.

The $\cos\left(\dfrac{(ω_1+ω_2)t}{2}\right)$ term which is an oscillation at a frequency which is the average of $\omega_1$ and $\omega_2$.

The $A\sin\left(\dfrac{(ω_1-ω_2)t}{2}\right)$ term which oscillates at a lower frequency, $\dfrac{(ω_1-ω_2)}{2}$, than the other term and has an amplitude $A$.

Adding some numbers, let $A=1,\,\omega_1 = 2\,\pi\,100.5$ and $\omega_2 = 2\,\pi\,99.5$, which is a difference of $1\,\rm Hz$.

Thus $\dfrac{(ω_1+ω_2)}{2} = 2\,\pi\, 100$ and $\dfrac{(ω_1-ω_2)}{2} = 2\,\pi\, \frac 12$ ie oscillations at $100\,\rm Hz$ are changing in amplitude at a frequency of $\frac 12\,\rm Hz$ as shown in the graph below.

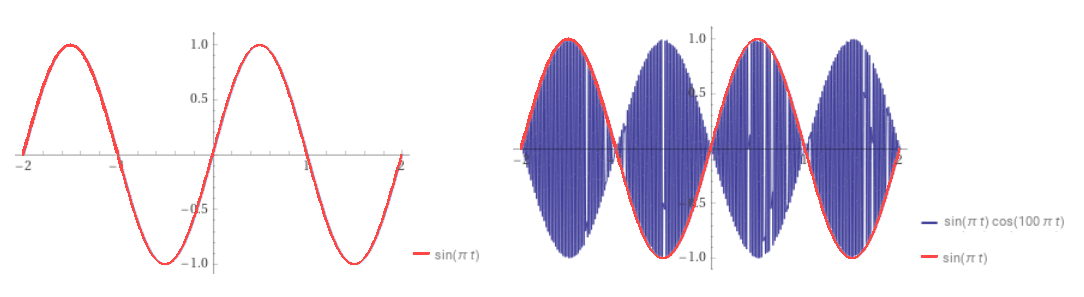
The variation in amplitude of the wave of frequency $100\,\rm Hz$ is shown in the right hand diagram.
The $100\,\rm Hz$ wave cannot be properly drawn because between $t=0\,\rm s$ and $t=1\,\rm s$ one hundred oscillations would have to be displayed.

What you will note is that there is zero amplitude of the $100\,\rm Hz$ wave at $t=0\,\rm s$, maximum amplitude at $t=\frac 12\,\rm s$, zero amplitude at at $t=1\,\rm s$, maximum amplitude at $t=\frac 32\,\rm s$, zero amplitude at at $t=2\,\rm s$.
Thus in $2$ seconds (the period of the $\sin \left(2\,\pi\,\frac12\right)$ term, the amplitude of the $100\rm \, Hz$ goes through two oscillations ie the beat frequency is $\dfrac{(ω_1-ω_2)}{2} \times 2 = ω_1-ω_2$ which in the case is $100.5-99.5=1\,\rm Hz$.

---

Perhaps what you actually hear will become clearer after you use this rather nice simulation, Interference in Time and Beats Simulation?

When first started the simulation sets $f_{\rm A}=300\,\rm Hz$ and $f_{\rm B}=305\,\rm Hz$.

On running the simulation you will hear a sound wave of frequency $302.5\rm\,Hz$ $pulsating$ at a frequency of $5\rm\,Hz$ which is the beat frequency.

Keeping frequency $f_{\rm A}$ the same change $f_{\rm B}$ to:

$\bf 1$ $301\,\rm Hz$ to hear a $1\rm\,Hz$ beat,

$\bf 2$ $300.1\,\rm Hz$ to hear a $0.1\rm\,Hz$, period $10\,\rm s$, beat,

$\bf 3$ $300.05\,\rm Hz$ to hear a $0.05\rm\,Hz$, period $20\,\rm s$, beat. [Do not worry that the hundredth of a hertz are not displayed as the simulation will still work.]

$\bf 4$ Have fun noting that the lowest available frequency is $40\,\rm Hz$ and the highest $1000\,\rm Hz$ so it is not possible with this simulation to show two ultrasonic frequencies producing an audible beat frequency eg $40,000\,\rm Hz$ and $40,100\,\rm Hz$ producing an audible $100\,\rm Hz$ tone

Answer 2

It's sort of a matter of definition, but the beat frequency is defined to be $\omega_1-\omega_2$ instead of half of that because that's the frequency at which the amplitude varies. That is, consider the following figure (from Wikipedia):

You can see that the period of the amplitude variation is actually half the period of the envelope function, and so the frequency is double what you would expect from the expression. This is the "correct" way to define it, because, for instance, if this wave was actually a sound, you would perceive the loudness of the sound vary with a frequency given by $\omega_1-\omega_2$, not half that.