Deciding amplitude for Beats

Question

I have two harmonic sound waves of nearly equal angular frequencies $\omega_1$ and $\omega_2$, and whose equations(which I have particularly modified for convenience), are $$s_1=a.\cos\omega_1 t$$ $$ s_2=a.\cos\omega_2t$$

Also, $s_1,s_2$ denote the transverse displacements.

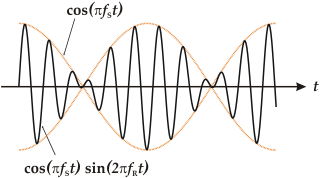
Assuming that $\omega_1>\omega_2$, and using Principle of Superposition of Waves, we get that the resultant wave is $$S=2a\cos\frac{(\omega_1-\omega_2)t}{2}.\cos\frac{(\omega_1+\omega_2)t}{2}.$$

Now how am I to decide the amplitude of this resultant wave? In all books, the (variable )amplitude is taken to be $\displaystyle A=2a\cos\frac{(\omega_1-\omega_2)t}{2}$. How do I assert this?

I mean, the amplitude may as well be $\displaystyle A=2a\cos\frac{(\omega_1+\omega_2)t}{2}$. What decides the above given amplitude?

Answer 1

At the beginning of your post you said the frequencies $\omega_1$ and $\omega_2$ are nearly equal.

Hence, $\omega_1 - \omega_2$ is much smaller than $\omega_1 + \omega_2$.

From that you can conclude that $\cos\frac{(\omega_1-\omega_2)t}{2}$ oscillates much slower than $\cos\frac{(\omega_1+\omega_2)t}{2}$.

Therefore it makes sense to call this slowly varying part the amplitude, like the red envelope line in this diagram from Wikipedia:Beating Frequency.